Revision NotesExam QuestionsPast Papers
A LevelPhysicsOCRRevision Notes6. Particles & Medical PhysicsElectric FieldsCoulomb's Law

Coulomb's Law (OCR A Level Physics)

Revision Note

Ashika

Author

Ashika

Last updated

Coulomb's Law

  • All charged particles produce an electric field around it

    • This field exerts a force on any other charged particle within range

  • The electrostatic force between two charges is defined by Coulomb’s Law

    • Recall that the charge of a uniform spherical conductor can be considered as a point charge at its centre

  • Coulomb’s Law states that:

    The electrostatic force between two point charges is proportional to the product of the charges and inversely proportional to the square of their separation

  • According to Coulomb's law, the electrostatic force between two point charges is calculated as follows:

F equals fraction numerator Q q over denominator 4 straight pi epsilon subscript italic 0 r squared end fraction

  • Where:

    • F = electrostatic force (N)

    • Q, q = magnitudes of the charges (C)

    • r = distance between the centres of the two charges (m)

    • ε0 = permittivity of free space = 8.85 × 10–12 C2 N–1 m–2

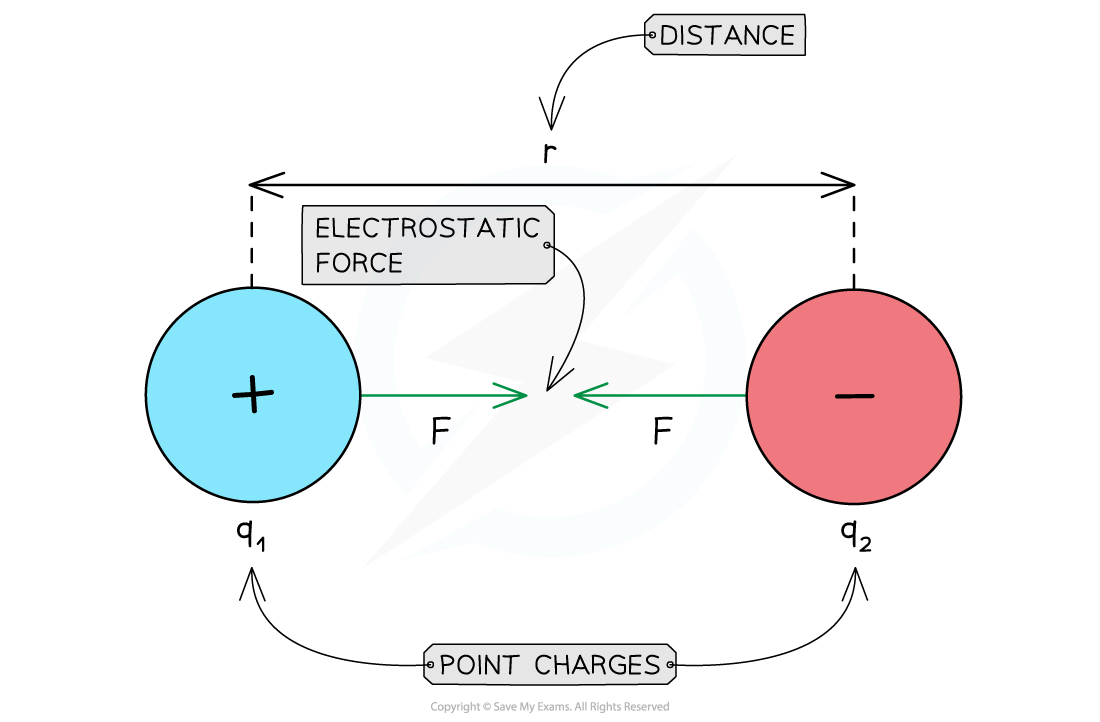
5-1-5-coulomb_s-law_sl-physics-rn

Attractive electrostatic force between two opposite charges

  • The 1/r2 relation is called the inverse square law

    • This means that when the separation of two charges doubles, the electrostatic force between them reduces by (½)2 = ¼

  • ε0 is the permittivity of free space

    • ε0 = 8.85 × 10–12 C2 N–1 m–2 and refers to charges in a vacuum

    • The value of the permittivity of air is taken to be the same as ε0

    • Any other material has a higher permittivity ε > ε0

    • ε is a measure of the resistance offered by a material in creating an electric field within it

Repulsive & Attractive Forces 

  • For like charges:

    • The product Qq is positive

    • F is positive

    • The charges repel each other

  • For opposite charges:

    • The product Qq is negative

    • F is negative

    • The charges attract each other

Worked Example

An alpha particle is situated 2.0 mm away from a gold nucleus in a vacuum.

Assuming them to be point charges, calculate the magnitude of the electrostatic force acting on each of the charges.

  • Atomic number of helium = 2

  • Atomic number of gold = 79

Answer:

Step 1: Write down the known quantities

  • Distance, r = 2.0 mm = 2.0 × 10–3 m

  • Atomic number of helium = 2

  • Atomic number of gold = 79

  • Elementary charge, e = 1.60 × 10–19 C (from the data booklet)

  • Permettivity of free space, ε0 = 8.85 × 10–12 C2 N–1 m–2

Note: that you must convert the distance from millimetres (mm) into metres (m)

Step 2: Calculate the charges of the alpha particle and gold nucleus 

  • The elementary charge e (when taken to be positive) is the charge of a proton

    • An alpha particle (helium nucleus) has 2 protons

      • So its charge is:

q = 2 × (1.60 × 10–19) = 3.2 × 10–19 C

  • A gold nucleus has 79 protons

    • So its charge is:

Q = 79 × (1.60 × 10–19) = 1.264 × 10–17 C

Step 3: Write down the equation to calculate the electrostatic force

F equals fraction numerator Q q over denominator 4 straight pi epsilon subscript italic 0 r squared end fraction

Step 4: Substitute the numbers into the equation 

F equals fraction numerator open parentheses 3.2 cross times 10 to the power of negative 19 end exponent close parentheses open parentheses 1.264 cross times 10 to the power of negative 17 end exponent close parentheses over denominator 4 straight pi open parentheses 8.85 cross times 10 to the power of negative 12 end exponent close parentheses open parentheses 2.0 cross times 10 to the power of negative 3 end exponent close parentheses squared end fraction

F = 9.1 × 10–21 N

Examiner Tips and Tricks

You do not need to memorise the numerical value of the Coulomb's constant k or that of the permittivity of free space ε0. They will both be given in the data booklet.

Unless specified in the question, you should assume that charges are located in a vacuum.

You should note that Coulomb's law can only be applied to charged spheres whose size is much smaller than their separation. Only in this case, the point charge approximation is valid. You must remember that the separation r must be taken from the centres of the spheres.

You cannot use Coulomb's law to calculate the electrostatic force between charges distributed on irregularly-shaped objects.

You've read 0 of your 5 free revision notes this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

    Test yourself

    Did this page help you?

    Previous:Electric Field StrengthNext:Electric Field Strength of a Point Charge
    Ashika

    Author: Ashika

    Expertise: Physics Project Lead

    Ashika graduated with a first-class Physics degree from Manchester University and, having worked as a software engineer, focused on Physics education, creating engaging content to help students across all levels. Now an experienced GCSE and A Level Physics and Maths tutor, Ashika helps to grow and improve our Physics resources.