Energy & Mass Equation (OCR A Level Physics) : Revision Note
Energy & Mass Equation
Einstein showed in his theory of relativity that matter can be considered a form of energy and hence, he proposed:
Mass can be converted into energy
Energy can be converted into mass
This is known as mass-energy equivalence, and can be summarised by the equation:
E = mc2
Where:
E = energy (J)
m = mass (kg)
c = the speed of light (m s-1)
Some examples of mass-energy equivalence are:
The fusion of hydrogen into helium in the centre of the sun
The fission of uranium in nuclear power plants
Nuclear weapons
High-energy particle collisions in particle accelerators
Energy Released in Nuclear Reactions
The binding energy is equal to the amount of energy released in forming the nucleus, and can be calculated using:
E = (Δm)c2
Where:
E = Binding energy released (J)
Δm = mass defect (kg)
c = speed of light (m s-1)
The daughter nuclei produced as a result of both fission and fusion have a higher binding energy per nucleon than the parent nuclei
Therefore, energy is released as a result of the mass difference between the parent nuclei and the daughter nuclei
Worked Example
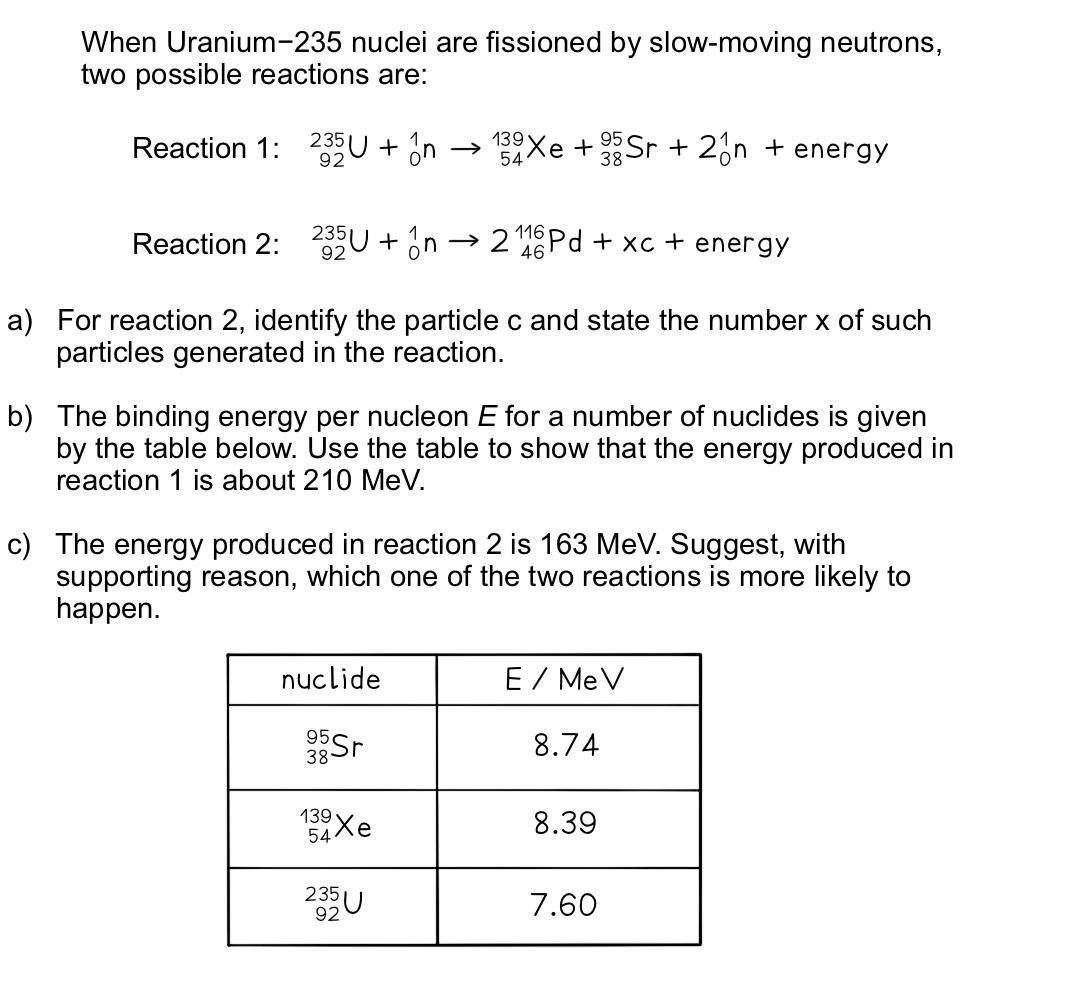
Answer:
Part (a)
Step 1: Balance the number of protons on each side (bottom number)
92 = (2 × 46) + xnp (where np is the number of protons in c)
xnp = 92 – 92 = 0
Therefore, c must be a neutron
Step 2: Balance the number of nucleons on each side
235 + 1 = (2 × 116) + x
x = 235 + 1 – 232 = 4
Therefore, 4 neutrons are generated in the reaction
Part (b)
Step 1: Find the binding energy of each nucleus
Total binding energy of each nucleus = Binding energy per nucleon × Mass number
Binding energy of 95Sr = 8.74 × 95 = 830.3 MeV
Binding energy of 139Xe = 8.39 × 139 = 1166.21 MeV
Binding energy of 235U = 7.60 × 235 = 1786 MeV
Step 2: Calculate the difference in energy between the products and reactants
Energy released in reaction 1 = ESr + EXe – EU
Energy released in reaction 1 = 830.3 + 1166.21 – 1786
Energy released in reaction 1 = 210.5 MeV
Part (c)
Since reaction 1 releases more energy than reaction 2, its end products will have a higher binding energy per nucleon
Hence, they will be more stable
This is because the more energy is released, the further it moves up the graph of binding energy per nucleon against nucleon number (A)
Since at high values of A, binding energy per nucleon gradually decreases with A
Nuclear reactions will tend to favour the more stable route, therefore, reaction 1 is more likely to happen

You've read 0 of your 5 free revision notes this week
Sign up now. It’s free!
Did this page help you?
