Mass Defect & Binding Energy (OCR A Level Physics): Revision Note
Exam code: H556
Mass Defect & Binding Energy
Mass Defect & Binding Energy
Experiments into nuclear structure have found that the total mass of a nucleus is less than the sum of the masses of its constituent nucleons
This difference in mass is known as the mass defect or mass deficit
Mass defect is defined as:
The difference between the measured mass of a nucleus and the sum total of the masses of its constituents
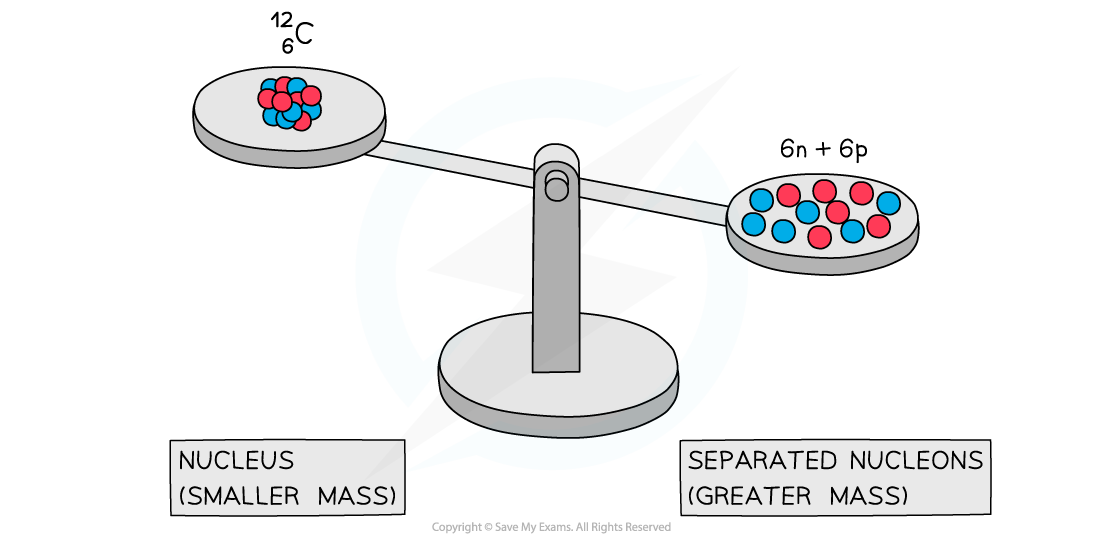
A system of separated nucleons has a greater mass than a system of bound nucleons
Due to the equivalence of mass and energy, this decrease in mass implies that energy is released in the process
Since nuclei are made up of neutrons and protons, there are forces of repulsion between the positive protons
Therefore, it takes energy, ie. the binding energy, to hold nucleons together as a nucleus
Binding energy is defined as:
The energy required to break a nucleus into its constituent protons and neutrons
Energy and mass are proportional, so, the total energy of a nucleus is less than the sum of the energies of its constituent nucleons
The formation of a nucleus from a system of isolated protons and neutrons is therefore an exothermic reaction - meaning that it releases energy
Examiner Tips and Tricks
Avoid describing the binding energy as the energy stored in the nucleus – this is not correct – it is energy that must be put into the nucleus to pull it apart.
Binding Energy per Nucleon Graph
In order to compare nuclear stability, it is more useful to look at the binding energy per nucleon
The binding energy per nucleon is defined as:
The binding energy of a nucleus divided by the number of nucleons in the nucleus
A higher binding energy per nucleon indicates a higher stability
In other words, it requires more energy to pull the nucleus apart
Iron (A = 56) has the highest binding energy per nucleon, which makes it the most stable of all the elements
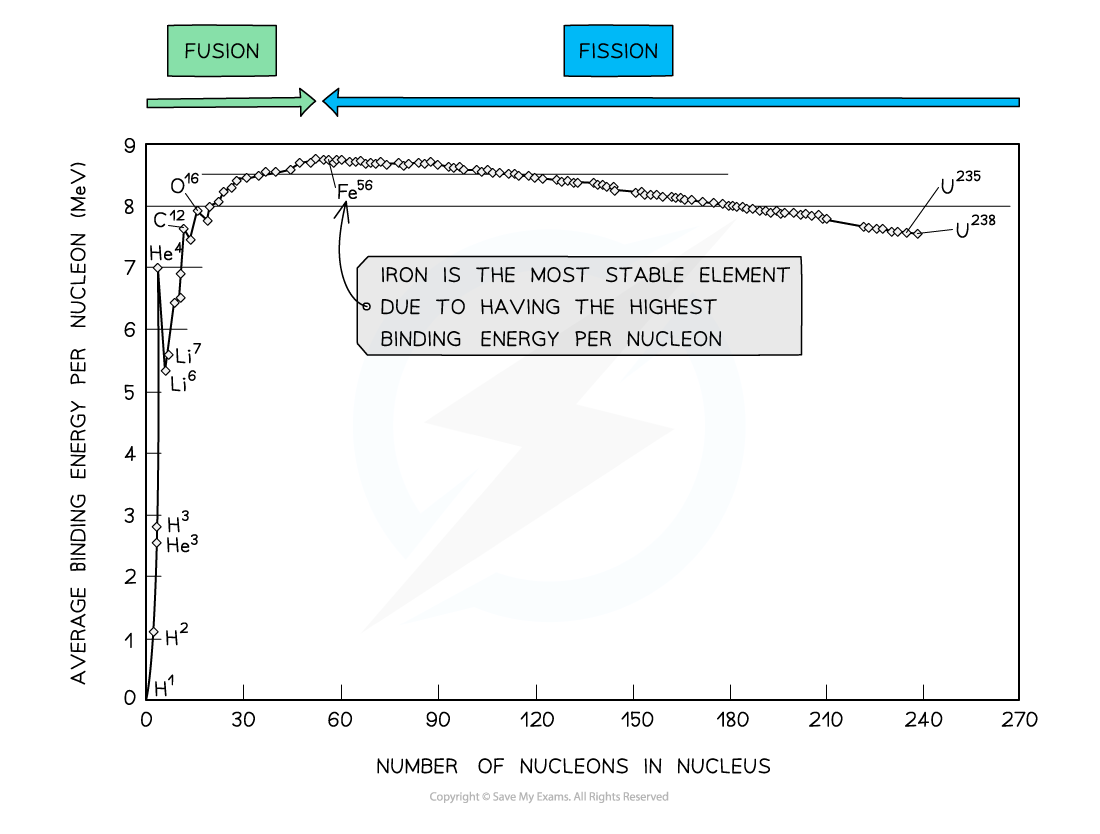
By plotting a graph of binding energy per nucleon against nucleon number, the stability of elements can be inferred
Key Features of the Graph
At low values of A:
Nuclei tend to have a lower binding energy per nucleon, hence, they are generally less stable
This means the lightest elements have weaker electrostatic forces and are the most likely to undergo fusion
Helium (4He), carbon (12C) and oxygen (16O) do not fit the trend
Helium-4 is a particularly stable nucleus hence it has a high binding energy per nucleon
Carbon-12 and oxygen-16 can be considered to be three and four helium nuclei, respectively, bound together
At high values of A:
The general binding energy per nucleon is high and gradually decreases with A
This means the heaviest elements are the most unstable and likely to undergo fission
Worked Example
The following equation represents one possible decay of the induced fission of a nucleus of uranium-235.
The graph shows the binding energy per nucleon plotted against nucleon number A.
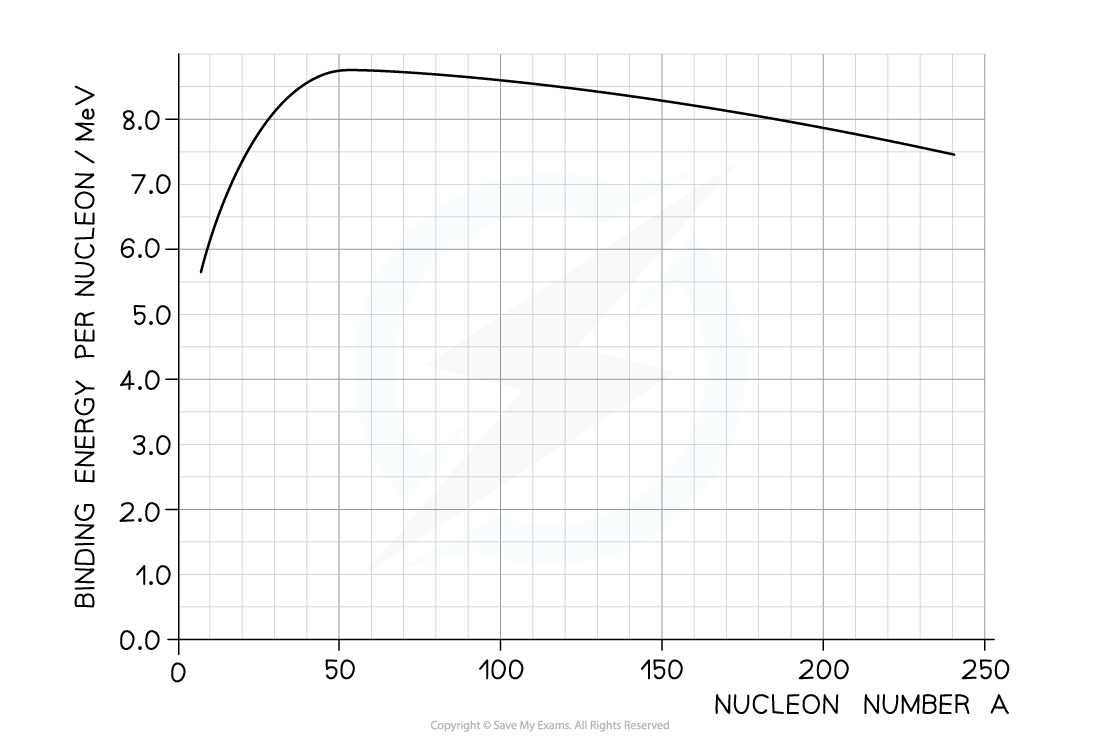
Calculate the energy released by the fission of uranium-235.
Answer:
Step 1: Use the graph to identify each isotope’s binding energy per nucleon
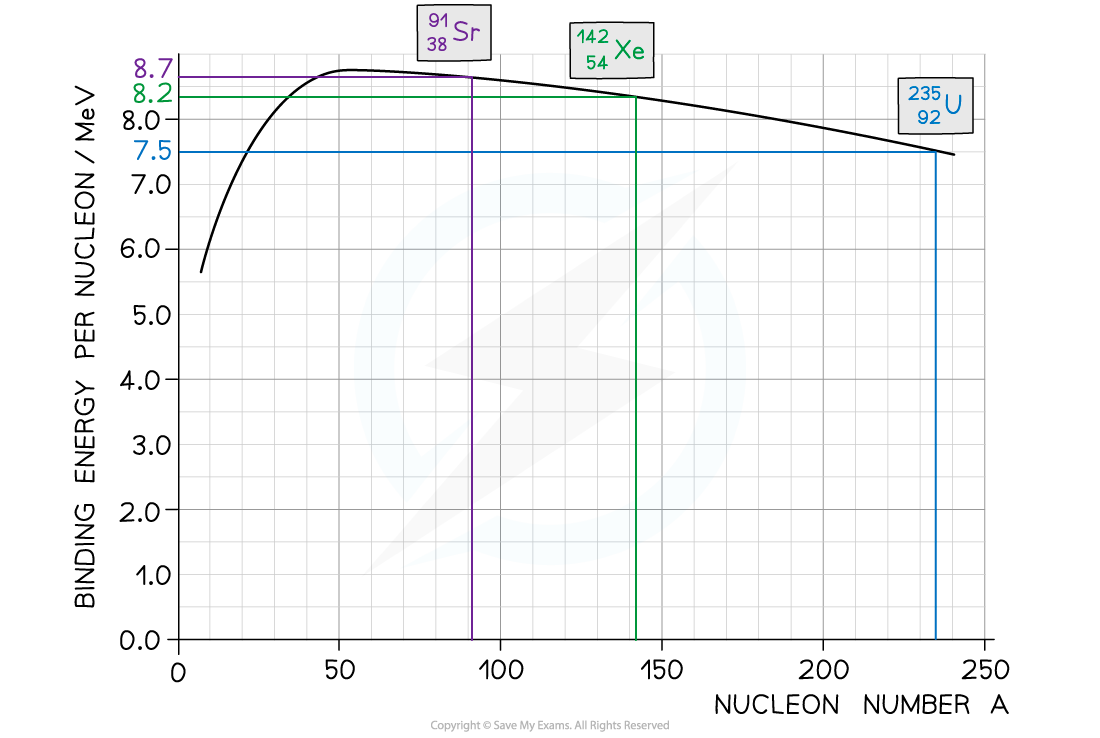
Binding energy per nucleon (U-235) = 7.5 MeV
Binding energy per nucleon (Sr-91) = 8.2 MeV
Binding energy per nucleon (Xe-142) = 8.7 MeV
Step 2: Determine the binding energy of each isotope
Binding energy = Binding Energy per Nucleon × Mass Number
Binding energy of U-235 nucleus = (235 × 7.5) = 1763 MeV
Binding energy of Sr-91 = (91 × 8.2) = 746 MeV
Binding energy of Xe-142 = (142 × 8.7) = 1235 MeV
Step 3: Calculate the energy released
Energy released = Binding energy after (Sr + Xe) – Binding energy before (U)
Energy released = (1235 + 746) – 1763 = 218 MeV
Examiner Tips and Tricks
Checklist on what to include (and what not to include) in an exam question asking you to draw a graph of binding energy per nucleon against nucleon number:
You will be expected to draw the best fit curve AND a cross to show the anomaly that is helium
Do not begin your curve at A = 0, this is not a nucleus!
Make sure to correctly label both axes AND units for binding energy per nucleon
You will be expected to include numbers on the axes, mainly at the peak to show the position of iron (56Fe)

Unlock more, it's free!
Did this page help you?