Capacitors in Series & Parallel Circuits (OCR A Level Physics) : Revision Note
Capacitors in Series
Consider two parallel plate capacitors C1 and C2 connected in series, with a potential difference (p.d.) V across them
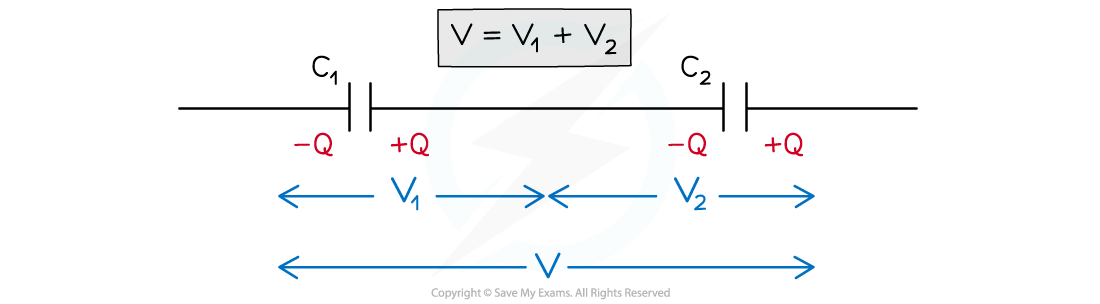
Capacitors connected in series have different p.d. across them but have the same charge
In a series circuit, p.d. is shared between all the components in the circuit
Therefore, if the capacitors store the same charge on their plates but have different p.ds, the p.d. across C1 is V1 and across C2 is V2
The total potential difference V is the sum of V1 and V2
V = V1 + V2
Rearranging the capacitance equation for the p.d., V, means V1 and V2 can be written as:
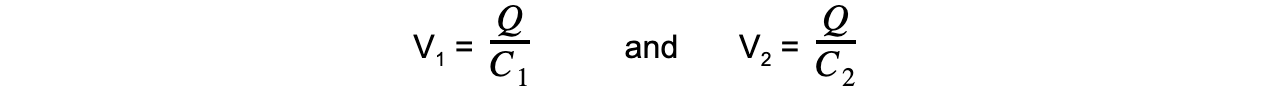
Where the total p.d. V is defined by the total capacitance
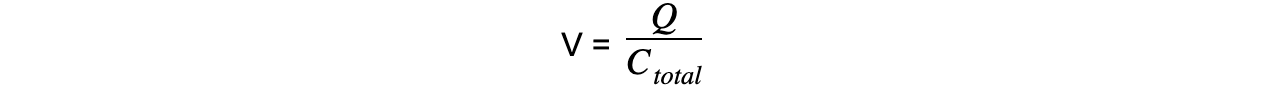
Substituting these into the equation V = V1 + V2 equals:
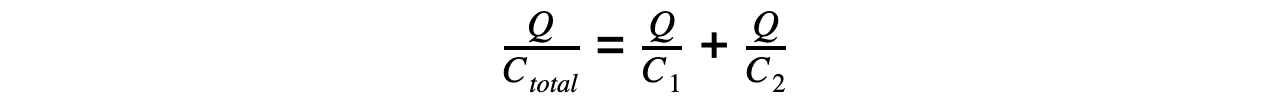
Since the current is the same through all components in a series circuit, the charge Q is the same through each capacitor and cancels out
Therefore, the equation for combined capacitance of capacitors in series is:
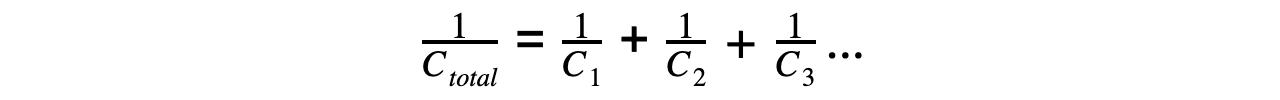
Capacitors in Parallel
Consider two parallel plate capacitors C1 and C2 connected in parallel, each with p.d V
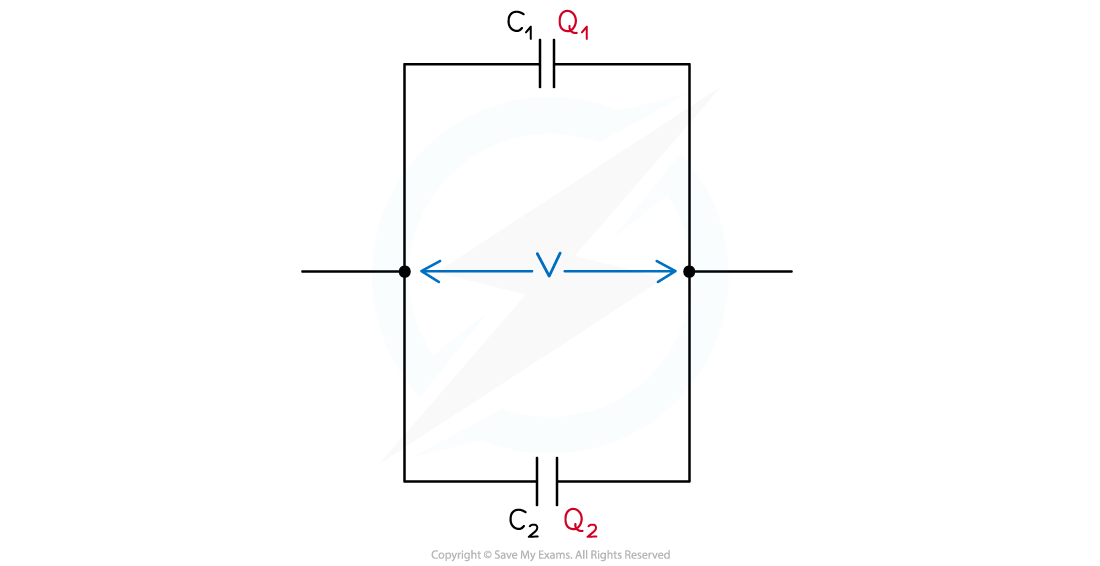
Capacitors connected in parallel have the same p.d across them, but different charge
Since the current is split across each junction in a parallel circuit, the charge stored on each capacitor is different
Therefore, the charge on capacitor C1 is Q1 and on C2 is Q2
The total charge Q is the sum of Q1 and Q2
Q = Q1 + Q2
Rearranging the capacitance equation for the charge Q means Q1 and Q2 can be written as:
Q1 = C1V and Q2 = C2V
Where the total charge Q is defined by the total capacitance:
Q = CtotalV
Substituting these into the Q = Q1 + Q2 equals:
CtotalV = C1V + C2V = (C1 + C2) V
Since the p.d is the same through all components in each branch of a parallel circuit, the p.d V cancels out
Therefore, the equation for combined capacitance of capacitors in parallel is:
Ctotal = C1 + C2 + C3 ...
Solving Problems with Capacitors in Series & Parallel
For capacitors in series:
For capacitors in parallel:
Worked Example
Three capacitors with capacitance of 23 μF, 35 μF and 40 μF are connected as shown below.

Calculate the total capacitance between points A and B.
Answer:
Step 1: Calculate the combined capacitance of the two capacitors in parallel
Capacitors in parallel: Ctotal = C1 + C2 + C3 …
Cparallel = 23 + 35 = 58 μF
Step 2: Connect this combined capacitance with the final capacitor in series
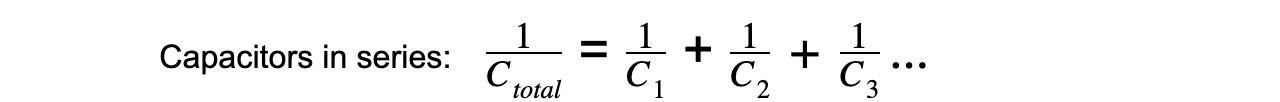
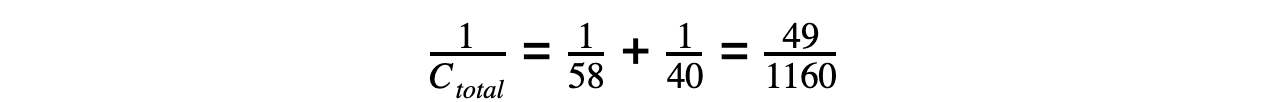
Step 3: Rearrange for the total capacitance
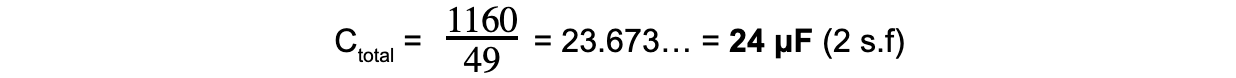
Examiner Tips and Tricks
Both the combined capacitance equations look similar to the equations for combined resistance in series and parallel circuits. However, take note that they are the opposite way around to each other!

You've read 0 of your 5 free revision notes this week
Sign up now. It’s free!
Did this page help you?
