Kepler's Three Laws of Motion (OCR A Level Physics)

Kepler's Three Laws of Motion

Kepler's First Law

• Kepler's First Law describes the shape of planetary orbits

• It states: 

  The orbit of a planet is an ellipse, with the Sun at one of the two foci

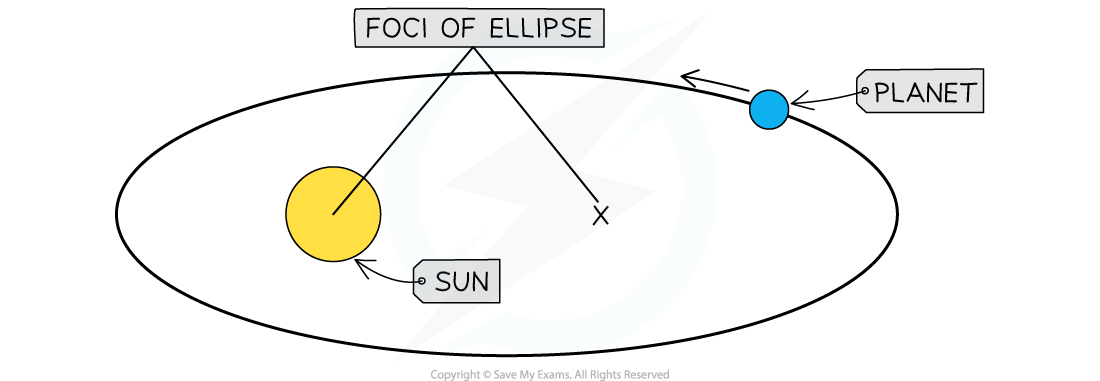
The orbit of all planets are elliptical, and with the Sun at one focus

• An ellipse is just a 'squashed' circle

  • Some planets, like Pluto, have highly elliptical orbits around the Sun

  • Other planets, like Earth, have near circular orbits around the Sun

Kepler's Second Law

• Kepler's Second Law describes the motion of all planets around the Sun

• It states: 

  A line segment joining the Sun to a planet sweeps out equal areas in equal time intervals

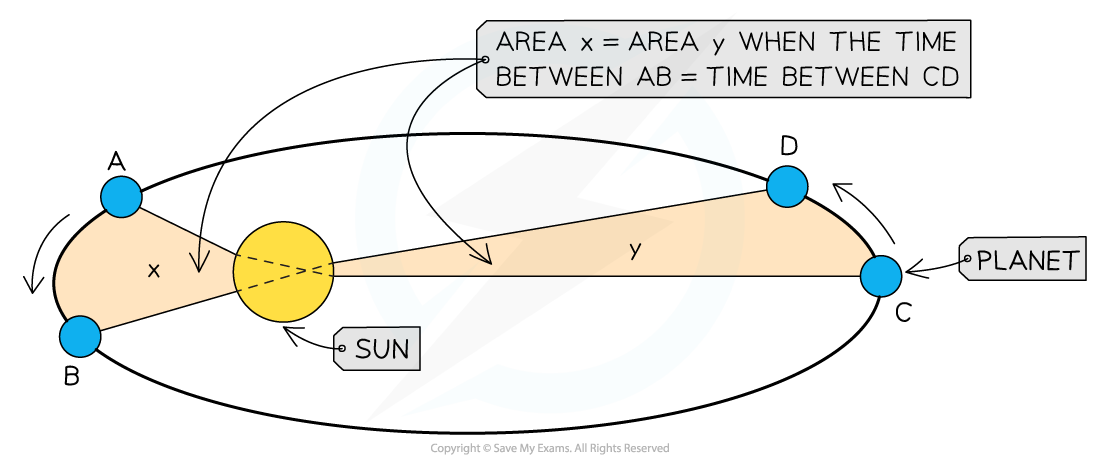
• The consequence of Kepler's Second Law is that planets move faster nearer the Sun and slower further away from it

Kepler's Third Law

• Kepler's Third Law describes the relationship between the time of an orbit and its radius

• It states;

  The square of the orbital time period T is directly proportional to the cube of the orbital radius r

• Kepler's Third Law can be written mathematically as:

T² ∝ r³

• Which becomes:

= k

• Where: 

  • T = orbital time period (s)

  • r = mean orbital radius (m)

  • k = constant (s² m^–3)

• In the case of our solar system, k is constant for all planets orbiting the Sun 

Applications of Kepler's Third Law

• Kepler's Third Law, the fact that T² ∝ r³ applies to any body in orbit about some larger body

• This means that it can be applied to other systems, not just the planets in our Solar System orbiting the sun, for example: 

  • The moons orbiting other planets, like the four moons of Jupiter (Io, Europa, Callisto and Ganymede)

  • Exoplanets in orbit about foreign stars

• This is useful because measuring things like time period and orbital radius are commonplace in modern astrophysics

  • Therefore, useful and interesting data about the mass of orbital systems can be deduced from experimental data