Kinetic Theory of Gases (OCR A Level Physics): Revision Note

Model of the Kinetic Theory of Gases

• Gases consist of atoms or molecules randomly moving around at high speeds

• The kinetic theory of gases models the thermodynamic behaviour of gases by linking:

  • The microscopic properties of particles i.e. mass and speed

  • The macroscopic properties of particles i.e. pressure and volume

• The theory is based on a set of the following assumptions:

  • Molecules of a gas behave as identical (or all have the same mass)

  • Molecules of gas are hard, perfectly elastic spheres

  • The volume of the molecules is negligible compared to the volume of the container

  • The time of a collision is negligible compared to the time between collisions

  • There are no intermolecular forces between the molecules (except during impact)

  • The molecules move in continuous random motion

  • Newton's laws apply

  • There are a very large number of molecules

• The number of molecules of gas in a container is very large, therefore the average behaviour (eg. speed) is usually considered

Pressure in the Model of Kinetic Theory of Gases

• A gas is made of a large number of particles

  • Gas particles have mass and move randomly at high speeds

• Pressure in a gas is due to the collisions of the gas particles with the walls of the container that holds the gas

• When a gas particle hits a wall of the container, it undergoes a change in momentum due to the force exerted by the wall on the particle (as stated by Newton's Second Law)

  • Final momentum = –mv

  • Initial momentum = mv

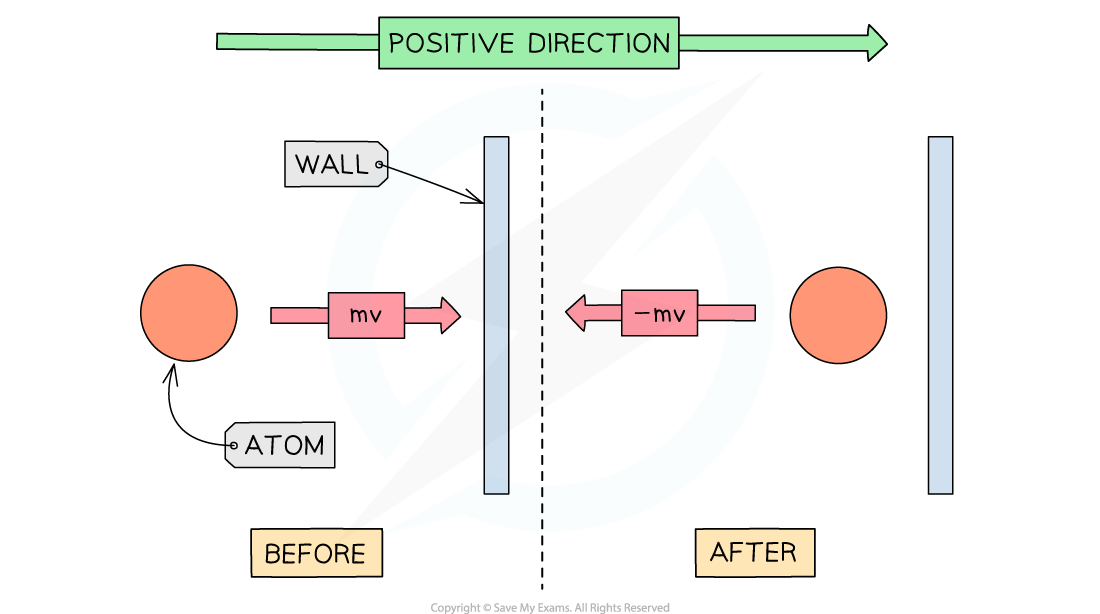
• Therefore, the change in momentum Δp can be written as:

Δp = final momentum – initial momentum

Δp = –mv – mv = –2mv

• According to Newton's Third Law, there is an equal and opposite force exerted by the particle on the wall (i.e. F = )

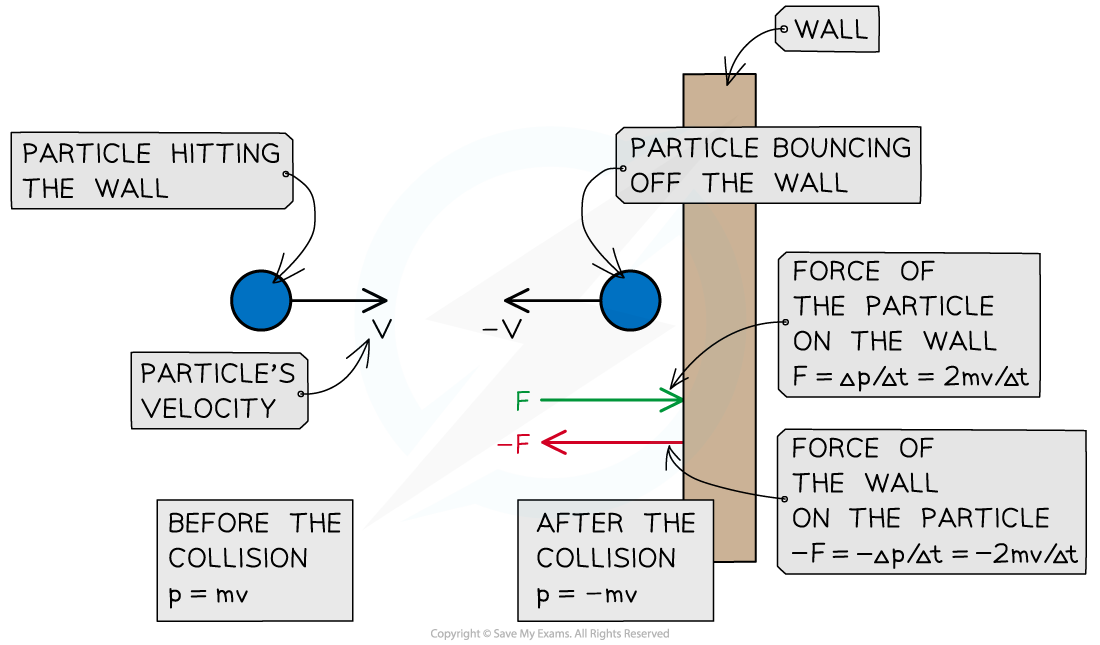
A particle hitting a wall of the container in which the gas is held experiences a force from the wall and a change in momentum. The particle exerts an equal and opposite force on the wall

• Since there is a large number of particles, their collisions with the walls of the container give rise to gas pressure, which is calculated as follows:

• Where:

  • p = pressure in pascals (Pa)

  • F = force in newtons (N)

  • A = area in metres squared (m²)