Specific Latent Heat (OCR A Level Physics)

• Energy is required to change the state of substance
• Examples of changes of state are:
  • Melting = solid to liquid
  • Evaporation/vaporisation/boiling = liquid to gas
  • Sublimation = solid to gas
  • Freezing = liquid to solid
  • Condensation = gas to liquid

 

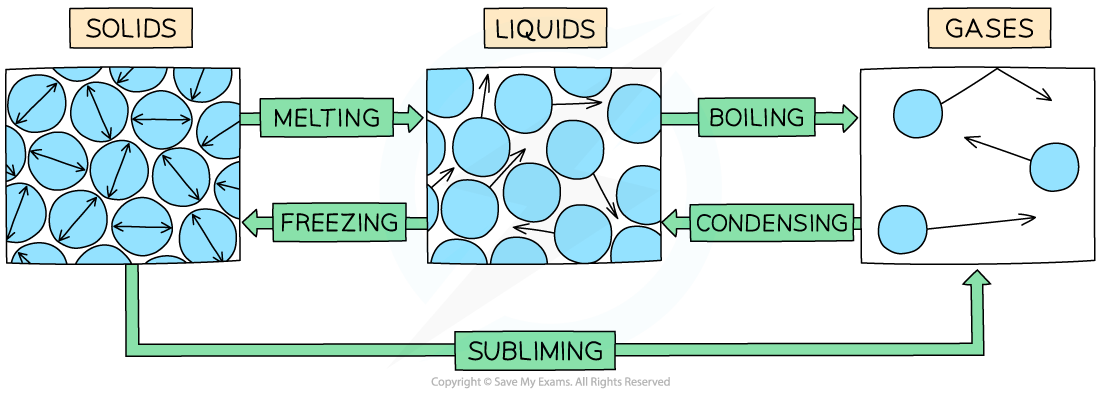
The example of changes of state between solids, liquids and gases

• When a substance changes state, there is no temperature change
• The energy supplied to change the state is called the latent heat and is defined as:

The thermal energy required to change the state of 1 kg of mass of a substance without any change of temperature

• There are two types of latent heat:
  • Specific latent heat of fusion (melting)
  • Specific latent heat of vaporisation (boiling)

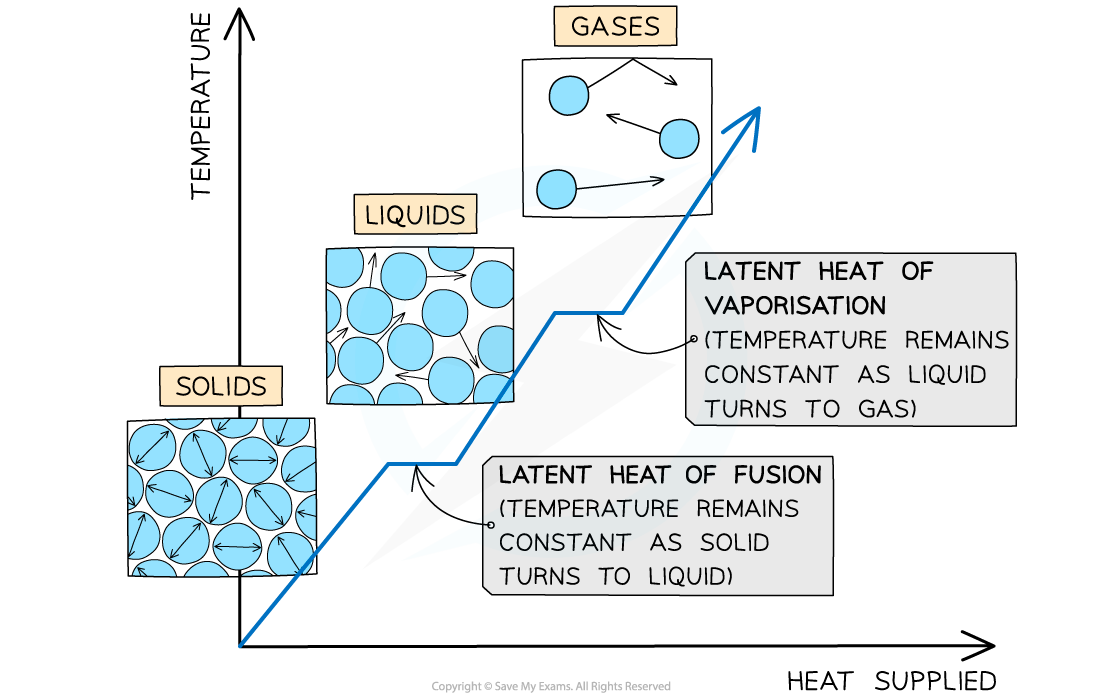
 The changes of state with heat supplied against temperature. There is no change in temperature during changes of state

• The specific latent heat of fusion is used when a solid is melting or a liquid is freezing
  • It is defined as:

The thermal energy required to convert 1 kg of solid to liquid with no change in temperature

• The specific latent heat of vaporisation is used when a liquid is vapourising or a gas is condensing
  • It is defined as:

 The thermal energy required to convert 1 kg of liquid to gas with no change in temperature

• The amount of energy E required to melt or vaporise a mass of m with latent heat L is:

 E = mL

• Where:
  • E = amount of thermal energy to change the state (J)
  • L = latent heat of fusion or vaporisation (J kg⁻¹)
  • m = mass of the substance changing state (kg)

• Specific latent heat of fusion is represented by L_f 
• Specific latent heat of vaporisation is represented by L_v 

• The values of latent heat for water are:
  • Specific latent heat of fusion = 330 kJ kg⁻¹
  • Specific latent heat of vaporisation = 2.26 M J kg⁻¹

• Therefore, evaporating 1 kg of water requires roughly seven times more energy than melting the same amount of ice to form water
• The reason for this is to do with intermolecular forces:
  • When ice melts: energy is required to increase the molecular separation until they can flow freely over each other
  • When water boils: energy is required to completely separate the molecules until there are no longer forces of attraction between the molecules,
    • This requires much more energy

Step 1: State the known values

• • Mass, m = 530 g = 0.53 kg
  • Energy supplied = 0.6 MJ = 0.6 × 10⁶ J

Step 2: State the specific latent heat equation

E = mL 

Step 3: Rearrange for latent heat

Step 4: Substitute in the values

Step 5: State whether the value is the specific latent heat of vaporisation or fusion

• • L is the latent heat of vaporisation because the change in state is from liquid to gas (boiling)

Determining the Specific Latent Heat of Fusion, L_f

Equipment List

• Crushed ice
• Two funnels with filter paper
• Three retort stands
• Two thermometers
• Two electric balances
• An appropriate heater (e.g., an immersion heater)
• A power source
• A voltmeter, ammeter and stop-clock

Method

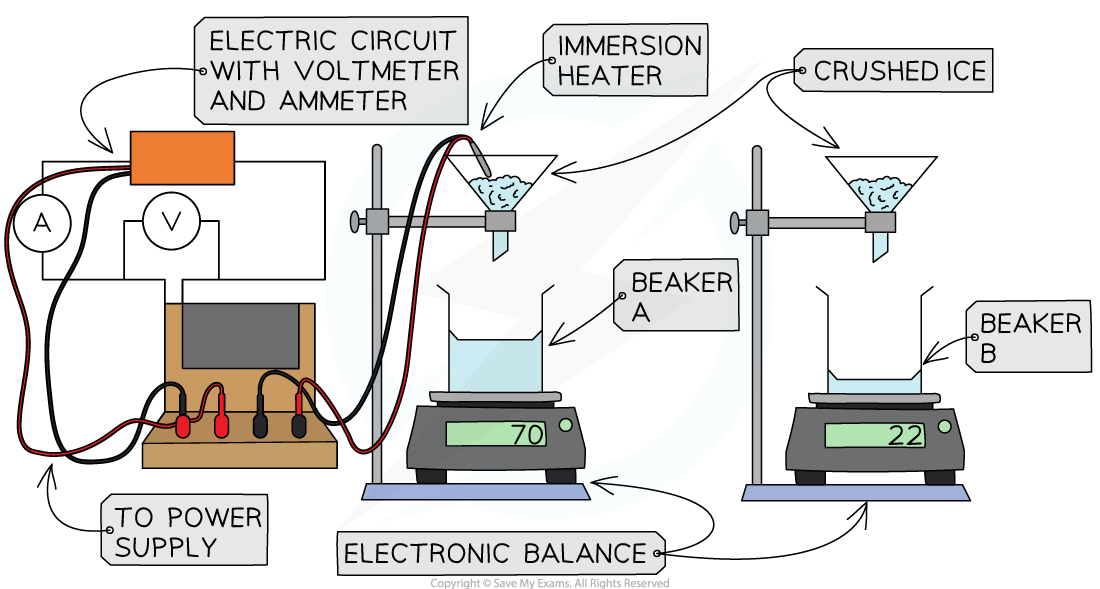
• Place a beaker on each balance
  • Leaving the beaker on the balance, zero the scale
• Arrange a funnel, clamped above each beaker
• Set up an immersion heater
  • Connect to the power source
  • Add an ammeter in series and a voltmeter in parallel
• Place the immersion heater in one of the funnels
• Measure out 50-100g of ice
  • Add the same mass of ice to each beaker
  • Record this value
• Turn on the immersion heater and start the stop watch
• Record the potential difference and current
• After a suitable period of time (around 5-10 minutes) remove the funnels, stop the stop watch and turn off the heater
• Record the mass of water in the beaker

Analysis

• The energy supplied to the ice can be calculated using the equation:

energy = current x potential difference x time

• Using the values for current, potential difference and time, calculate the energy supplied
• The specific latent heat of fusion can be calculated using the equation: 

energy = mass x specific latent heat

• The change mass is equal to the mass of water collected
  • To take into account melting due to heat transfer from the surroundings find the difference in mass between the two beakers of water
  • This gives the change in mass due to the energy supplied by the heater
• Calculate the mass of the melted ice and convert it into kg
  • Δm = m_A - m_B
  • Mass in g ÷ 1000 = Mass in kg
• Calculate the specific latent heat of fusion of ice to water using the equation for specific latent heat

Evaluation

• Errors may be introduced due to precision of the instruments
• Water may be absorbed by the filter paper
  • This will reduce the mass and therefore give a higher value for specific latent heat

Determining the Specific Latent Heat of Vaporisation, L_v

Equipment List

• A double-walled glass vessel with an inner flask containing water
• An appropriate electric heater (e.g., an immersion heater)
• A condenser with a collecting flask
• A power source
• A voltmeter, ammeter and stop-clock
• An electric balance

Method

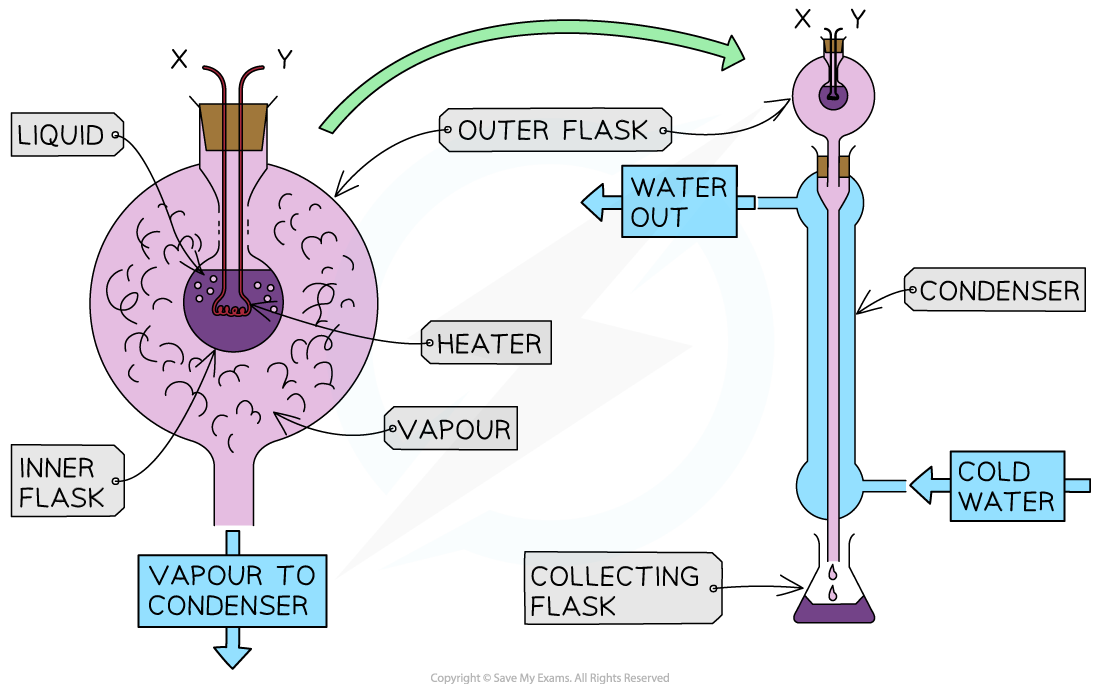
• Connect the double-walled glass vessel to the condenser
  • Place the collecting flask at the end of the condenser
• Set up an immersion heater
  • Connect to the power source
  • Add an ammeter in series and a voltmeter in parallel
• Place the immersion heater in the fluid
• Turn on the immersion heater and start the stop watch
• Record the potential difference and current
• After a suitable period of time (around 5-10 minutes), stop the stop watch and turn off the heater
• Record the mass of water collected in the conical flask

Analysis

• The energy supplied to the water can be calculated using the equation:

energy = current x potential difference x time

• Using the values for current, potential difference and time, calculate the energy supplied
• The specific latent heat of vaporisation can be calculated using
  • The mass of water collected
  • The energy supplied calculated
  • The equation: 

energy = mass x specific latent heat

Evaluation

• Errors may be introduced due to precision of the instruments
• Not all of the vapour which enters the condenser may make it to the beaker