Impulse (OCR A Level Physics) : Revision Note
Force & Momentum
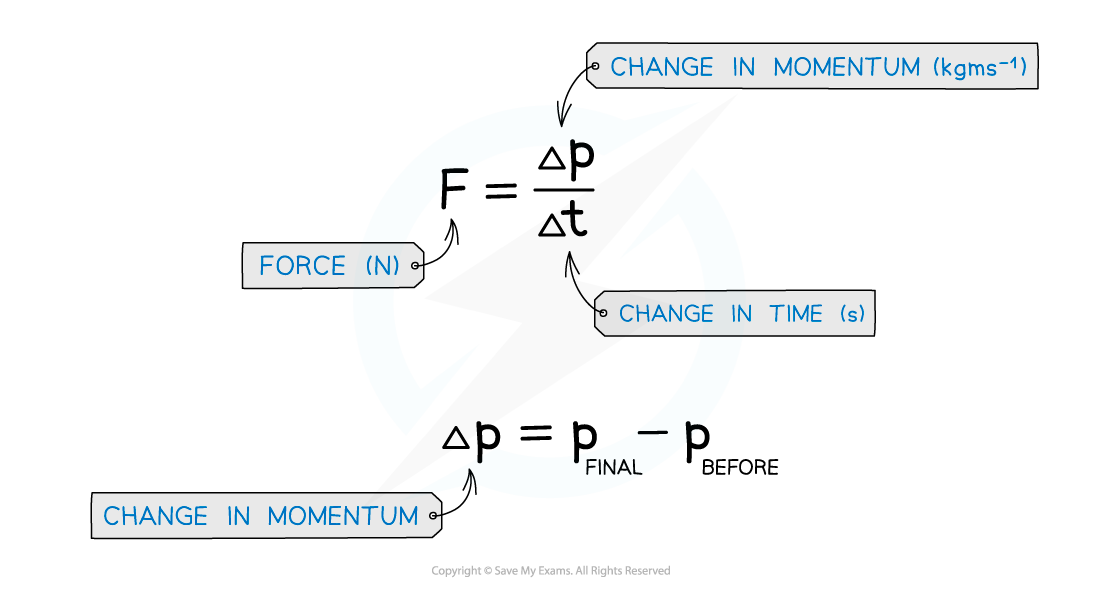
Force is defined as the rate of change of momentum on a body
The change in momentum is defined as the final momentum minus the initial momentum
These can be expressed as follows:
Direction of Forces
Force and momentum are vectors so they can take either positive or negative values
The force that is equal to the rate of change of momentum is still the resultant force
A force on an object will be negative if it is directed in the opposite motion to its initial velocity
This means that the force is produced by the object it has collided with
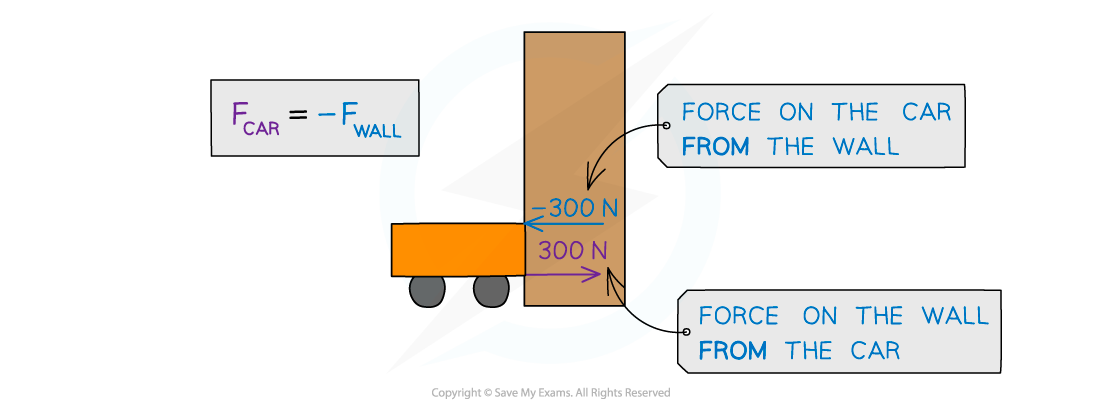
The wall produces a force of -300N on the car and (due to Newton’s Third Law) the car also produces a force of 300 N back onto the wall
Worked Example
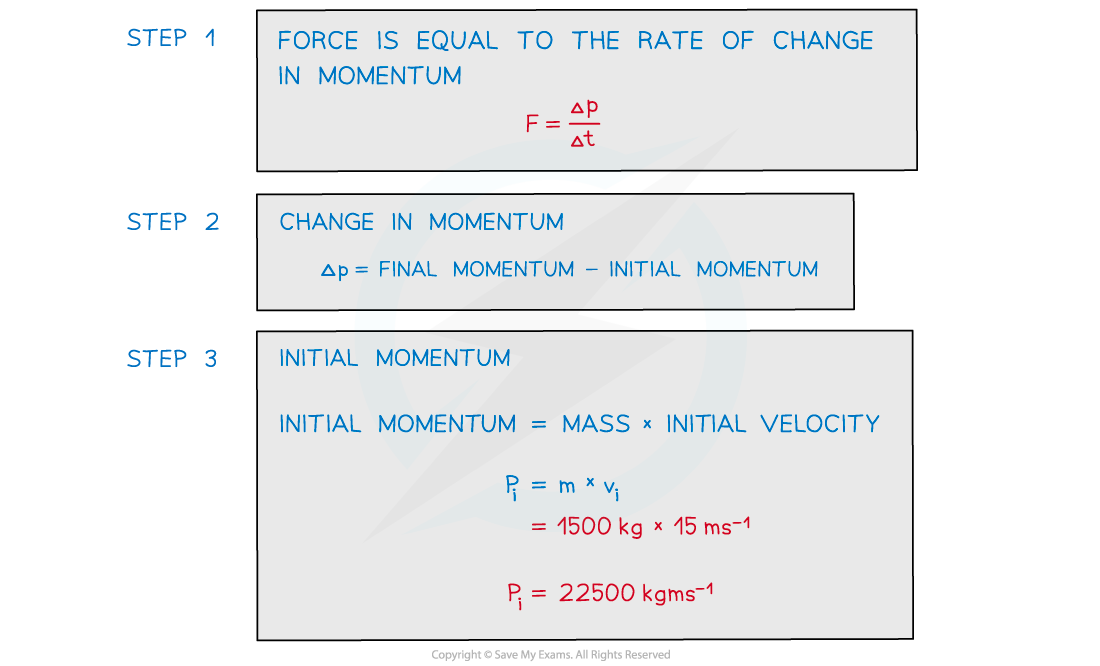
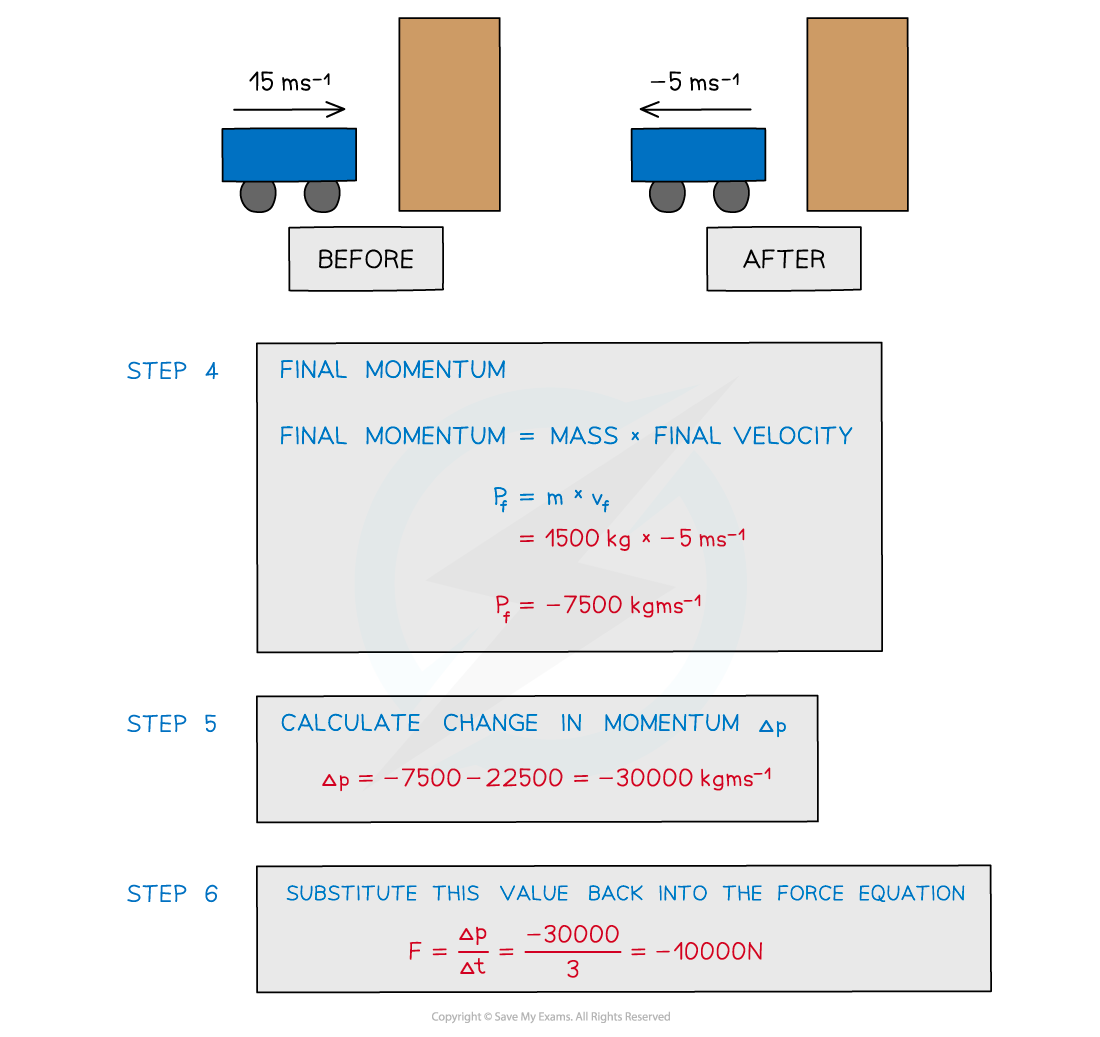
A car of mass 1500 kg hits a wall at an initial velocity of 15 m s-1.
It then rebounds off the wall at 5 m s-1 and comes to rest after 3.0 s.
Calculate the average force experienced by the car.
Answer:
Examiner Tips and Tricks
In an exam question, carefully consider what produces the force(s) acting. Look out for words such as ‘from’ or ‘acting on’ to determine this and don’t be afraid to draw a force diagram to figure out what is going on.
Impulse
The force and momentum equation can be rearranged to find the impulse
Impulse, I, is equal to the change in momentum:
I = FΔt = Δp = mv – mu
Where:
I = impulse (N s)
F = force (N)
t = time (s)
p = momentum (kg m s–1)
m = mass (kg)
v = final velocity (m s–1)
u = initial velocity (m s–1)
This equation is only used when the force is constant
Since the impulse is proportional to the force, it is also a vector
The impulse is in the same direction as the force
The unit of impulse is N s
The impulse quantifies the effect of a force acting over a time interval
This means a small force acting over a long time has the same effect as a large force acting over a short time
Examples of Impulse
An example in everyday life of impulse is when standing under an umbrella when it is raining, compared to hail (frozen water droplets)
When rain hits an umbrella, the water droplets tend to splatter and fall off it and there is only a very small change in momentum
However, hailstones have a larger mass and tend to bounce back off the umbrella, creating a greater change in momentum
Therefore, the impulse on an umbrella is greater in hail than in rain
This means that more force is required to hold an umbrella upright in hail compared to rain
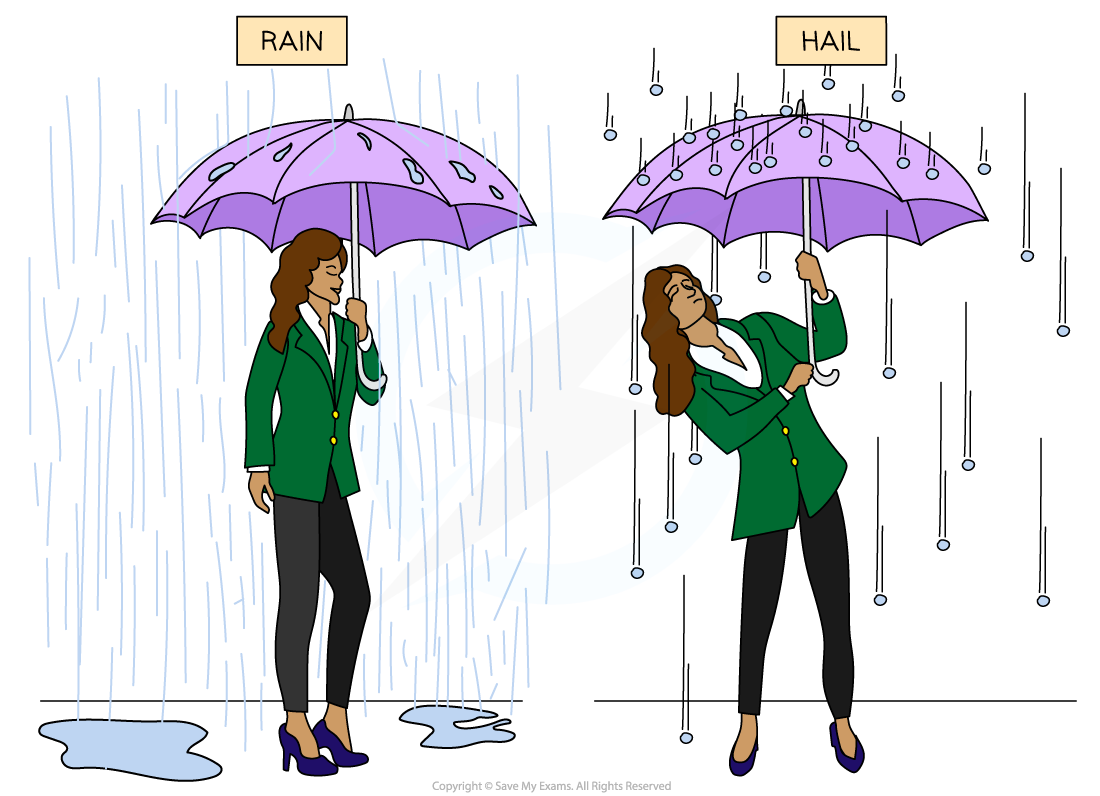
Since hailstones bounce back off an umbrella, compared to water droplets from rain, there is a greater impulse on an umbrella in hail than in rain
Worked Example
A 58 g tennis ball moving horizontally to the left at a speed of 30 m s–1 is struck by a tennis racket which returns the ball back to the right at 20 m s–1.
(i) Calculate the impulse delivered to the ball by the racket
(ii) State which direction the impulse is in
Answer:
Part (i)
Step 1: Write the known quantities
Taking the initial direction of the ball as positive (the left)
Initial velocity, u = 30 m s–1
Final velocity, v = –20 m s–1
Mass, m = 58 g = 58 × 10–3 kg
Step 2: Write down the impulse equation
Impulse I = Δp = m(v – u)
Step 3: Substitute in the values
I = (58 × 10–3) × (–20 – 30) = –2.9 N s
Part (ii)
Direction of the impulse
Since the impulse is negative, it must be in the opposite direction to which the tennis ball was initial travelling (since the left is taken as positive)
Therefore, the direction of the impulse is to the right
Examiner Tips and Tricks
Remember that if an object changes direction, then this must be reflected by the change in sign of the velocity. As long as the magnitude is correct, the final sign for the impulse doesn't matter as long as it is consistent with which way you have considered positive (and negative). For example, if the left is taken as positive and therefore the right as negative, an impulse of 20 N s to the right is equal to -20 N s

You've read 0 of your 5 free revision notes this week
Sign up now. It’s free!
Did this page help you?
