Work (Edexcel A Level Physics)

• Work is defined as

The amount of energy transferred when an external force causes an object to move over a certain distance

• If the force is parallel to the direction of the object's displacement, the work done can be calculated using the equation:

ΔW = FΔs

• Where:
  • ΔW = change in work done (J)
  • F = average force applied in the direction of the motion (N)
  • s = displacement (m)

• Force in the direction of the motion means that when a force is applied at an angle the component in the direction of motion is used to calculate, rather than the whole force

• In the diagram below, the man’s pushing force on the block is doing work as it is transferring energy to the block

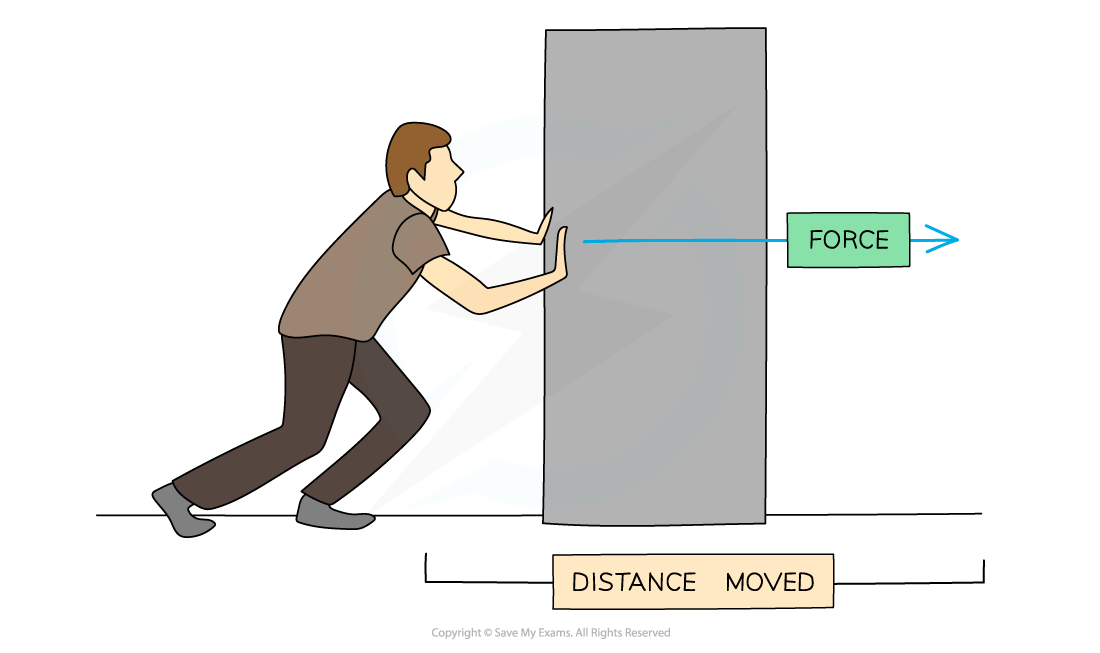
Work is done when a force is used to move an object over a distance

• When pushing a block, work is done against friction to give the box kinetic energy to move
  • The kinetic energy is transferred to other forms of energy such as heat and sound

• Usually, if a force acts in the direction that an object is moving then the object will gain energy
• If the force acts in the opposite direction to the movement then the object will lose energy

Calculating with Force at an Angle

• Sometimes the direction of motion of an object is not parallel to the direction of the force
• If the force is at an angle θ to the object's displacement, the work done is calculated by:

W = Fs cos θ

or

W = Fs sin θ

• Where θ is the angle, in degrees, between the direction of the force and the motion
• When the angle is between the force and the horizontal use cosine
• When the angle is between the force and the vertical, use sine
  • The component needed is the one that is parallel to the displacement

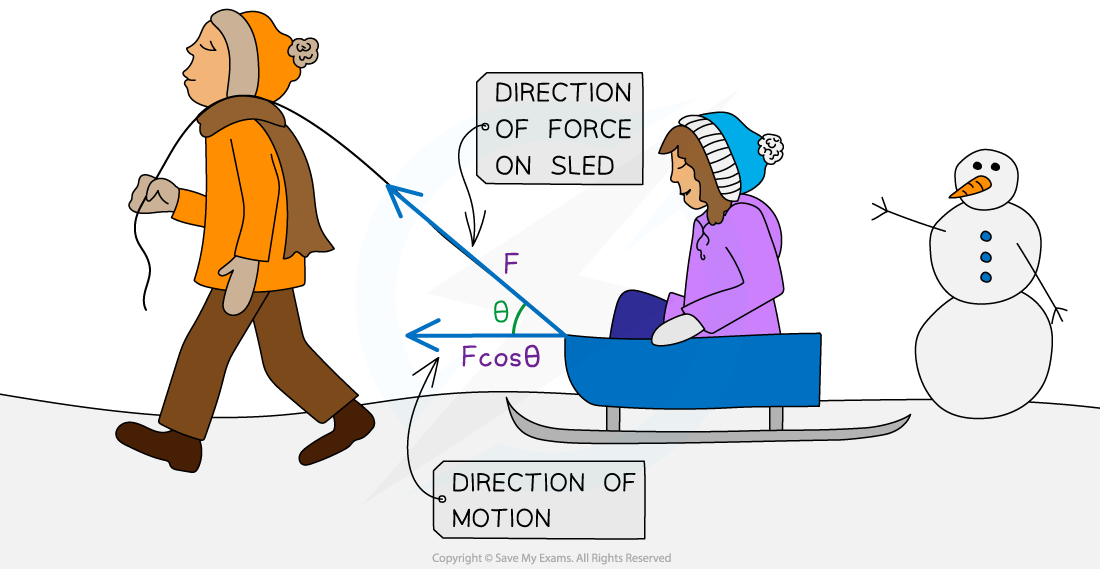
When the force is at an angle, only the component of the force in the direction of motion is considered for the work done

Step 1: Write the known values from the question

• • Force acting downwards, F =  2.5 × 10³ N
  • Angle of the slope, θ = 40^o
  • Displacement, s = 6.0 m

Step 2: Find the force in the direction of motion by resolving the forces 

• • Draw a diagram, showing the weight acting downwards
  • Resolve the weight into two components
  • The first component is parallel to the slope - the same as the direction of the motion; this is W sin θ
  • The second is at right angles to the slope, showing the normal reaction force (this component, W cos θ is not used in this answer)

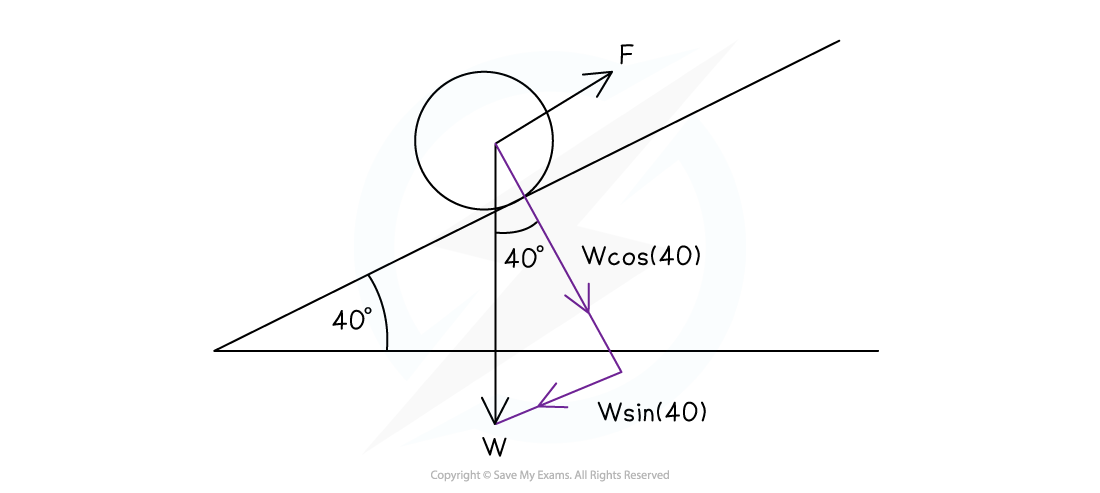
Force acting along the slope, F = W sin (40) = 2.5 × 10³ × sin (40) = 1 607 N

Step 3: Write the equation for work done and substitute in the values

ΔW = FΔs = 1 607 × 6.0 = 9 642 

Step 4: Give the answer to the correct number of significant figures and with units

ΔW = FΔs = 1 607 × 6.0 = 9.6 × 10³ J