Radioactive Decay | CIE A Level Physics Exam Questions 2025

1a

6 marks

Radioactive decay is often described as a spontaneous and random process.

State what is meant by

(i)

radioactive decay

[2]

(ii)

a spontaneous process

[2]

(iii)

a random process

[2]

How did you do?

1b

2 marks

The graph in Fig. 1.1 shows the count rate of a radioactive substance measured by a Geiger-Müller tube.

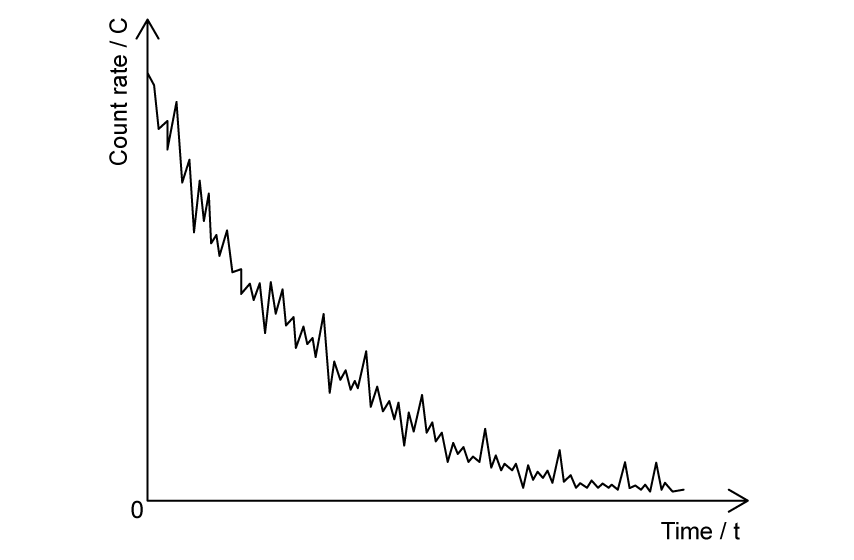
7-1-q2d-question-sl-sq-easy-phy

Fig. 1.1

State the feature of Fig. 1.1 which provides evidence for

(i)

the random nature of radioactive decay

[1]

(ii)

the spontaneous nature of radioactive decay

[1]

How did you do?

1c

6 marks

The element neptunium has at least 24 isotopes. One of the isotopes is neptunium-231 $({}_{93}^{231}{Np})$ , which has a half-life of 49 minutes.

(i)

State what is meant by isotopes and circle the possible isotopes of neptunium

${}_{93}^{239}{Np}$ ${}_{94}^{231}{Np}$ ${}_{93}^{219}{Np}$ ${}_{90}^{231}{Np}$ ${}_{93}^{244}{Np}$

[3]

(ii)

Define half-life and circle the correct expression for calculating the probability per second of decay of a nucleus of neptunium-231.

$\frac{49}{0.693}$ $\frac{0.693}{49}$ $\frac{0.693}{49 \times 60}$ $\frac{49 \times 60}{0.693}$

[3]

How did you do?

1d

3 marks

Fig. 1.2 shows a diagram in which nucleon number A is plotted against proton number Z.

The diagram shows the position of three isotopes of neptunium and their respective decay products.

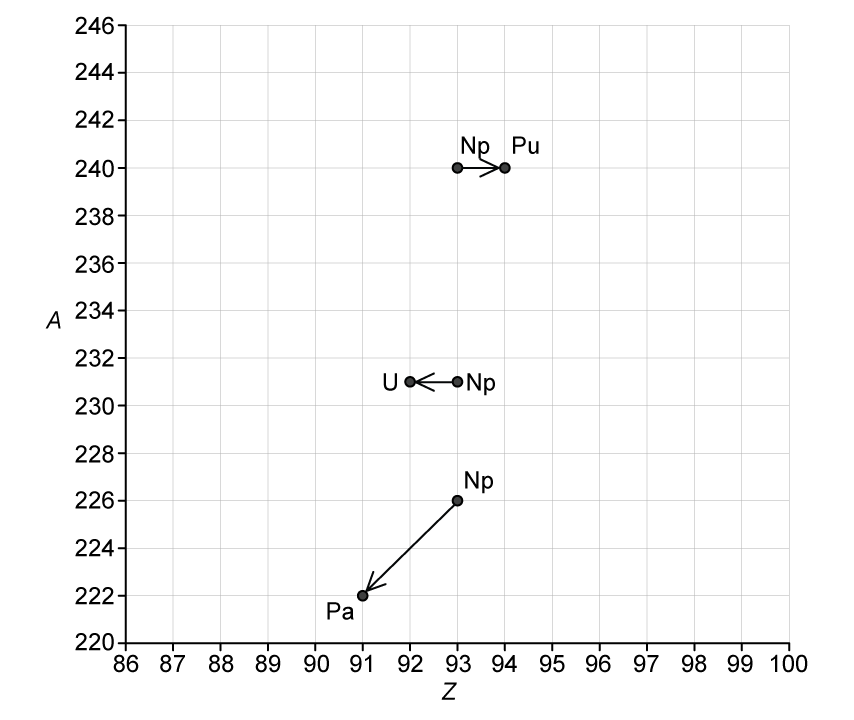
23-2-1d-e--23-2-e-a-z-nucleon-proton-graph-cie-ial-sq

Fig. 1.2

Use Fig. 1.2 to complete the following nuclear decay equations

${}_{. . .}^{. . .}{Np} \to {}_{. . .}^{. . .}{Pu} + . . . . . . . . . . . . . . . . . . . . .$

${}_{. . .}^{. . .}{Np} \to {}_{. . .}^{. . .}U + . . . . . . . . . . . . . . . . . . . . .$

${}_{. . .}^{. . .}{Np} \to {}_{. . .}^{. . .}{Pa} + . . . . . . . . . . . . . . . . . . . . .$

How did you do?

Did this page help you?

Radioactive Decay (CIE A Level Physics)

Exam Questions