When the force, the magnetic field and the current are all mutually perpendicular to each other, the directions of each can be represented by Fleming’s left–hand rule, as shown in Fig. 1.1.
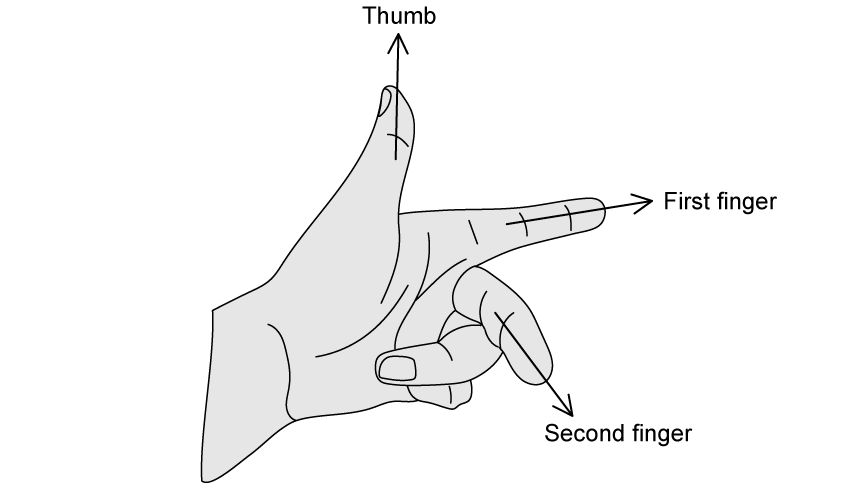
Fig. 1.1
State what is represented by the direction of
(i) the thumb
[1]
(ii) the first finger
[1]
(iii) the second finger
[1]
Fig. 1.2 shows a magnetic field.

Fig. 1.2
(i) State whether the magnetic field is acting into or out of the page.
[1]
(ii) An electron enters the magnetic field at point A, as shown in Fig. 1.3.
On Fig. 1.3, draw
1. an arrow on the electron to show the direction of the force
2. the path of the electron through the magnetic field

Fig. 1.3
[4]
When a moving charge enters a magnetic field the magnetic field produces a force on the charge, which can be calculated using
(i) State the meaning of the quantities B, q and v.
[3]
(ii) When in the field, the charge begins to move in a circular orbit.
State the name and equation of the force that causes the charge to move in a circular orbit.
[3]
When the electron enters the magnetic field, its speed is 3.0 × 106 m s–1. The magnetic field has a magnetic flux density of 3.2 × 10–3 T.
(i) Using the equations in (c), show that the radius of the circular orbit of the charged object inside the magnetic field is equal to
[3]
(ii) Calculate the radius of the circular orbit of the electron
Mass of an electron = 9.11 × 10–31 kg
Charge of an electron = 1.60 × 10–19 C
[2]
Did this page help you?




