Revision NotesExam QuestionsPast Papers
A LevelPhysicsCambridge (CIE)Revision Notes7. WavesProgressive WavesThe Wave Equation

Syllabus Edition

First teaching 2023

First exams 2025

|

The Wave Equation (Cambridge (CIE) A Level Physics)

Revision Note

Katie M

Written by: Katie M

Reviewed by: Caroline Carroll

Updated on

Derivation of v = f λ

  • Using the definitions of speed, frequency and wavelength, the wave equation v = fλ can be derived

  • This is an important relationship between three key properties of a wave

  • The derivation for this is shown below

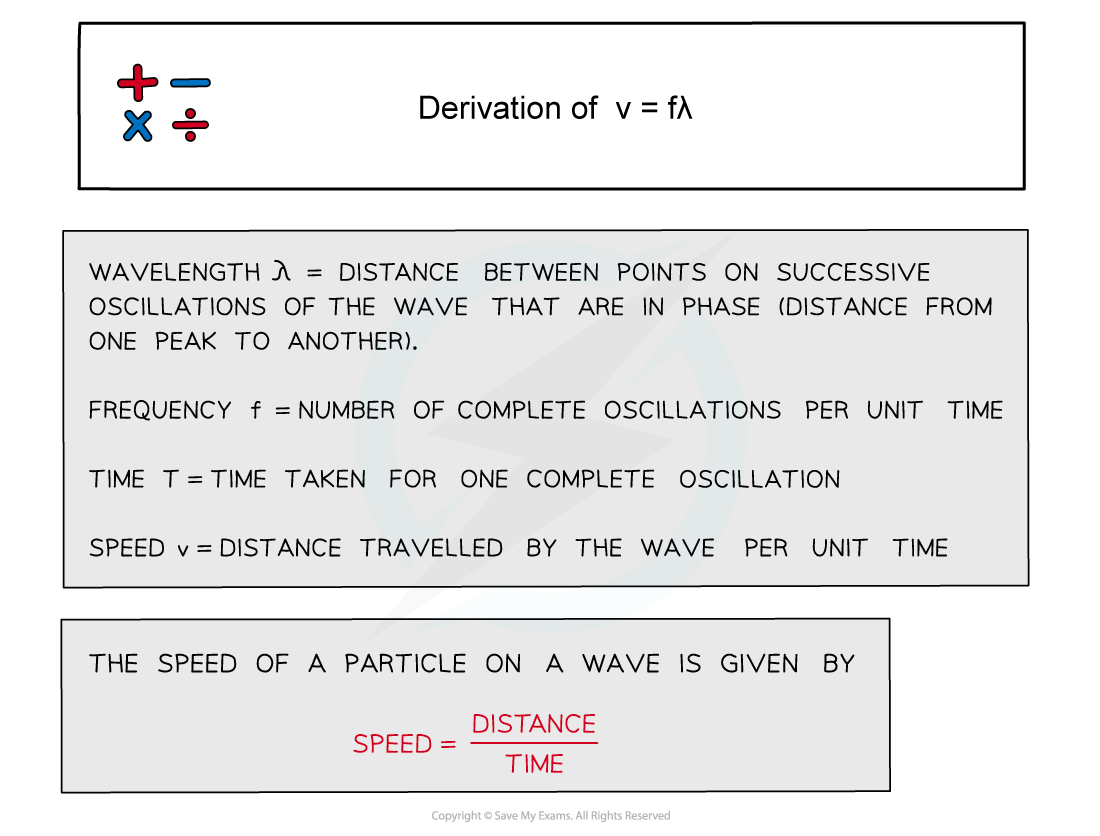
Derivation of v=fλ (1), downloadable AS & A Level Physics revision notes
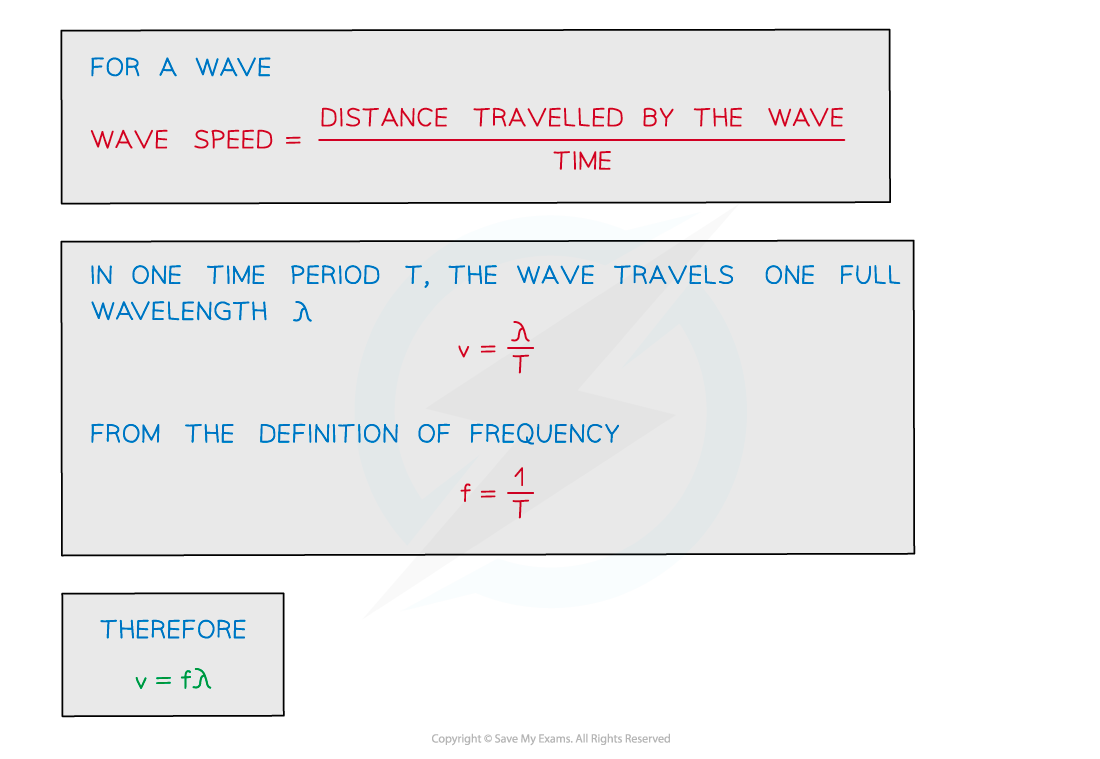
Derivation of v=fλ (2), downloadable AS & A Level Physics revision notes

Derivation of v = fλ

 

Examiner Tips and Tricks

You will be expected to remember all the steps for this derivation (but do not need to write the full definition for each variable). If you are unsure as to where speed = distance/time comes from, make sure to revisit chapter “2. Kinematics”.

The wave equation

  • The wave equation links the speed, frequency and wavelength of a wave

  • This is relevant for both transverse and longitudinal waves

v space equals space f lambda

  • Where

    • v is wave speed in m s−1

    • f is frequency in Hz

    • λ is wavelength in m

  • The wave equation tells us that for a wave at constant speed:

    • As the wavelength increases, the frequency decreases

    • As the wavelength decreases, the frequency increases

  • So wavelength and frequency are inversely proportional to each other

Wavelength and frequency are inversely proportional

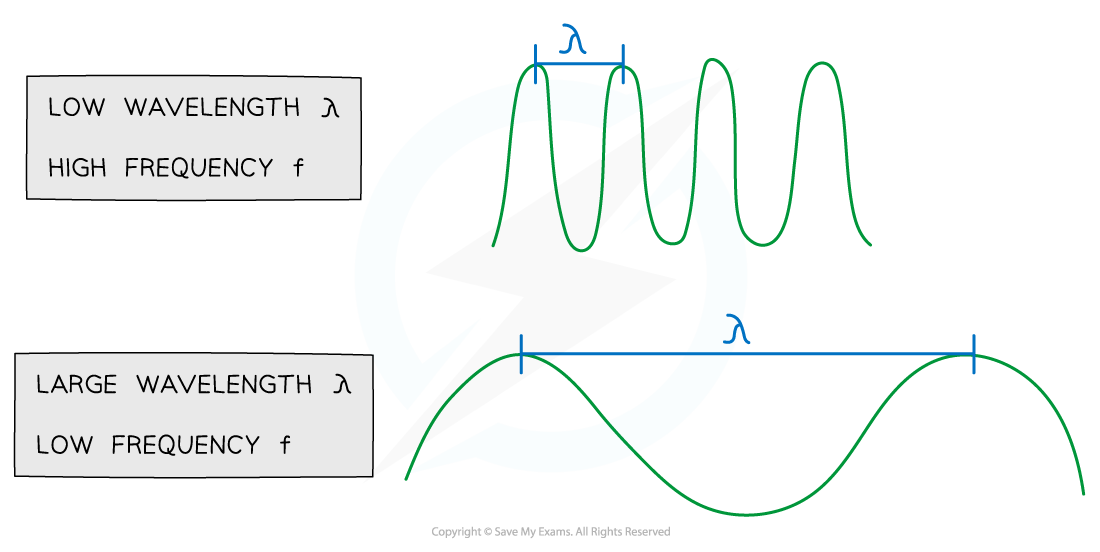
Frequency and wavelength, downloadable AS & A Level Physics revision notes

The relationship between frequency and wavelength of a wave

Worked Example

The wave in the diagram below has a speed of 340 m s–1.

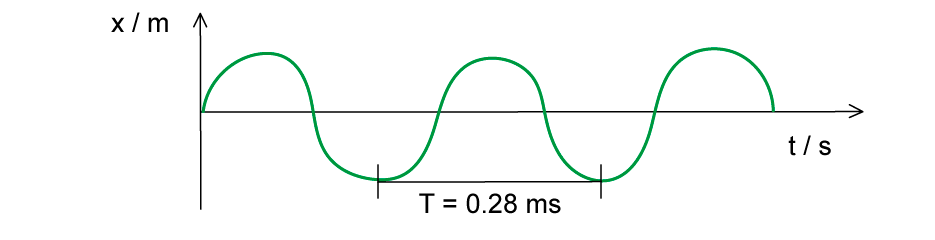
WE - Wave equation question image, downloadable AS & A Level Physics revision notes

What is the wavelength of the wave?

Answer:

Step 1: Determine the frequency of the wave:

  • Convert the period T into seconds and substitute into the frequency equation

f space equals space fraction numerator 1 over denominator 0.28 space cross times space 10 to the power of negative 3 end exponent end fraction space equals space 3571 space Hz

Step 2: Determine wavelength:

  • Rearrange the wavelength equation and substitute speed and frequency

lambda space equals space v over f space equals space 340 over 3571 space equals space 0.095 space straight m

Examiner Tips and Tricks

You may also see the wave equation be written as c = fλ where c is the wave speed. However, c is often used to represent the speed of light (3 x 108 ms-1). Only electromagnetic waves travel at this speed, therefore it’s best practice to use v  for any speed that isn’t the speed of light instead.

You've read 0 of your 5 free revision notes this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

    Test yourself

    Did this page help you?

    Previous:Cathode-Ray OscilloscopeNext:Wave Intensity
    Author
    Reviewer
    Katie M

    Author: Katie M

    Expertise: Physics

    Katie has always been passionate about the sciences, and completed a degree in Astrophysics at Sheffield University. She decided that she wanted to inspire other young people, so moved to Bristol to complete a PGCE in Secondary Science. She particularly loves creating fun and absorbing materials to help students achieve their exam potential.

    Caroline Carroll

    Author: Caroline Carroll

    Expertise: Physics Subject Lead

    Caroline graduated from the University of Nottingham with a degree in Chemistry and Molecular Physics. She spent several years working as an Industrial Chemist in the automotive industry before retraining to teach. Caroline has over 12 years of experience teaching GCSE and A-level chemistry and physics. She is passionate about creating high-quality resources to help students achieve their full potential.