A LevelPhysicsCIERevision Notes6. Deformation of Solids6.2 Elastic & Plastic BehaviourElastic Potential Energy

Syllabus Edition

First teaching 2023

First exams 2025

|

Elastic Potential Energy (CIE A Level Physics)

Revision Note

Test yourself
Katie M

Author

Katie M

Last updated

Area under a force-extension graph

  • The work done in stretching a material is equal to the force applied multiplied by the extension created
  • Therefore, the area under a force-extension graph is equal to the work done to stretch the material

 Area under force-extension graphs

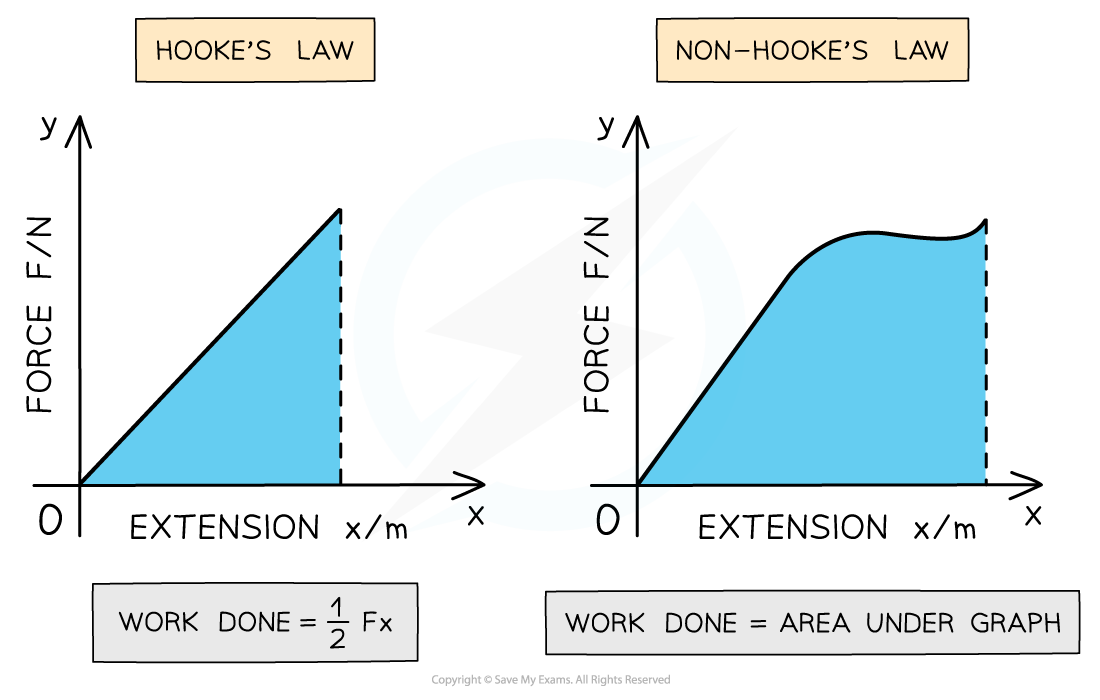
Work done under graphs, downloadable AS & A Level Physics revision notes

Work done is the area under the force - extension graph. This is true for graphs that obey Hooke's law and those which don't. 

  • For a region where a material demonstrates elastic behaviour and obeys Hooke’s law the work done is the area of the right angled triangle under the graph
  • For a region where a material doesn’t obey Hooke’s law, the total area is the sum of the areas of the separate sections under the graph

Worked example

The graph shows the behaviour of a sample of a metal when it is stretched until it starts to undergo plastic deformation. WE - Work done area under graph question image, downloadable AS & A Level Physics revision notes What is the total work done in stretching the sample from zero to an extension of 13.5 mm?

Simplify the calculation by treating the curve XY as a straight line.

Answer:

Step 1: Recall how to determine work done from the graph:

  • Work done is the area underneath the force-extension graph

Step 2: Calculate the area under the graph up to point X:

  • To point X, the area under the graph, AX , is a triangle

A subscript straight X space equals space 1 half space cross times space base space cross times space height

  • Calculate AX , remembering to convert length to metres

A subscript straight X space equals space 1 half space cross times space open parentheses 11.0 space cross times space 10 to the power of negative 3 end exponent close parentheses space cross times space 450 space equals space 2.475 space straight J

Step 3: Calculate the area between X and Y:

  • Assuming the line XY is a straight line, the area under this region of the graph forms a trapezium
  • Recall the equation for a trapezium of width h  and side lengths a  and b 

A subscript XY space equals space open parentheses fraction numerator a space plus space b over denominator 2 end fraction close parentheses h

    • Here, h is the change in extension from X to Y, 2.5 mm
    • a  is the load at point X and b  is the load at point Y

A subscript XY space equals space open parentheses fraction numerator 450 space plus space 600 over denominator 2 end fraction close parentheses space cross times space open parentheses 2.5 space cross times space 10 to the power of negative 3 end exponent close parentheses space equals space 1.313 space straight J

Step 4: Calculate total area:

  • The total area, the total work done, is just the sum of these two areas

work space done space equals space 2.475 space plus space 1.313 space equals space 3.79 space straight J

  • The answer is given to 3 significant figures, as the data has been given to this number of significant figures

Examiner Tip

Make sure to be familiar with the formula for the area of common 2D shapes such as a right angled triangle, trapezium, square and rectangles. If you do forget the equation for a trapezium's area, however, just split the shape up into rectangles and triangles.

Elastic Potential Energy

  • Elastic potential energy is defined as the energy stored within a material (e.g. in a spring) when it is stretched or compressed 
  • Elastic potential energy for a material deformed within its limit of proportionality is found from the area under the force-extension graph 
    • So, the work done is also equal to the elastic potential energy stored in the material when it demonstrates elastic behaviour up to the limit of proportionality

 

Worked example

A spring is extended with varying forces; the graph below shows the results.WE - EPE area under graph question image, downloadable AS & A Level Physics revision notes What is the energy stored in the spring when the extension is 40 mm?

Answer:

Step 1: Recall how to determine energy sto

  • Energy stored in the spring is equal to area under the graph, A
  • This is a triangle, so can be calculated using

A space equals space 1 half space cross times space base space cross times space height

Step 2: Find the area under the graph

  • At 40 mm, the load is approximately 8.3 N
  • Converting length into metres gives an area of

A space equals space 1 half space cross times space open parentheses 40 space cross times space 10 to the power of negative 3 end exponent close parentheses space cross times space 8.3 space equals space 0.166 space straight J

  • This is equal to the energy stored in the spring, which should be given to 2 significant figures

energy space stored space equals space 0.17 space straight J

Examiner Tip

In your exam, a range of values will be allowed for the load at 40 mm. Any value from 8.1 N to 8.5 N will gain a mark. If you are struggling, draw horizontal lines on the graph to show the positions of 9.0 N and 8.5 N.

Calculating elastic potential energy

  • Recall that for a material obeying Hooke’s Law, within its limit of proportionality, elastic potential energy and work done can be calculated using the following equation:

Work space Done space equals space EPE space equals space 1 half F x

  •  Recall the equation for Hooke's law:

F space equals space k x

  • Combining these two equations gives an equation for elastic potential energy in terms of spring constant, k:

EPE space equals space 1 half k x squared

  • Where:
    • k is the spring constant (N m-1)
    • x is the extension (m)

Examiner Tip

The formula for EPE = 1 half k x squared is only the area under the force-extension graph when it is a straight line i.e. when the material obeys Hooke’s law and is within its elastic limit.

You've read 0 of your 10 free revision notes

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

    Test yourselfNext topic

    Did this page help you?

    Katie M

    Author: Katie M

    Expertise: Physics

    Katie has always been passionate about the sciences, and completed a degree in Astrophysics at Sheffield University. She decided that she wanted to inspire other young people, so moved to Bristol to complete a PGCE in Secondary Science. She particularly loves creating fun and absorbing materials to help students achieve their exam potential.