Elastic & Plastic Behaviour (Cambridge (CIE) A Level Physics): Revision Note
Exam code: 9702
Elastic & plastic deformation
Types of deformation
Elastic deformation: when the load is removed, the object will return to its original natural length
Plastic deformation: when the load is removed, the object will not return to its original length
Elastic limit: the point beyond which the object does not return to its original length when the load is removed
Plastic deformation is beyond the elastic limit
These regions can be obtained from a force-extension graph:
Graph showing the elastic limit
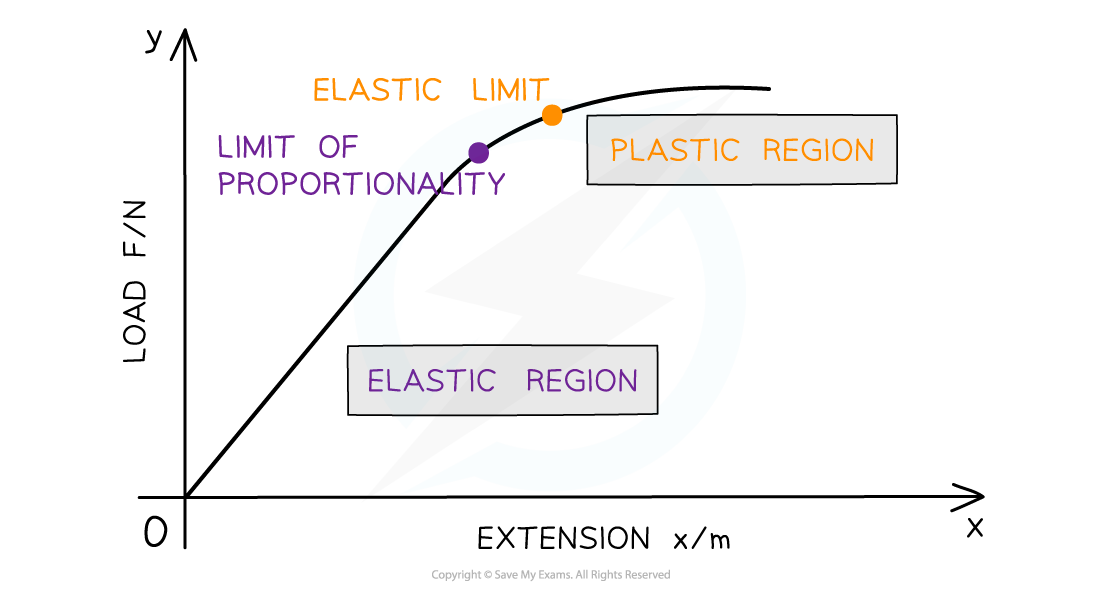
Below the elastic limit, the material exhibits elastic behaviour and returns to its original shape. Above the elastic limit, the material exhibits plastic behaviour
The linear portion of the graph represents the loads at which the force and extension are directly proportional
The limit of proportionality marks the upper end of this proportional relationship
Brittle and ductile materials
Brittle materials have very little to no plastic deformation
Glass and concrete are examples of brittle materials
A brittle material breaks with little elastic and insignificant plastic deformation
Ductile materials have a larger plastic region
Rubber and copper are examples of ductile materials
Ductile material stretches into a new shape before breaking
Brittle and ductile materials stress-strain graph
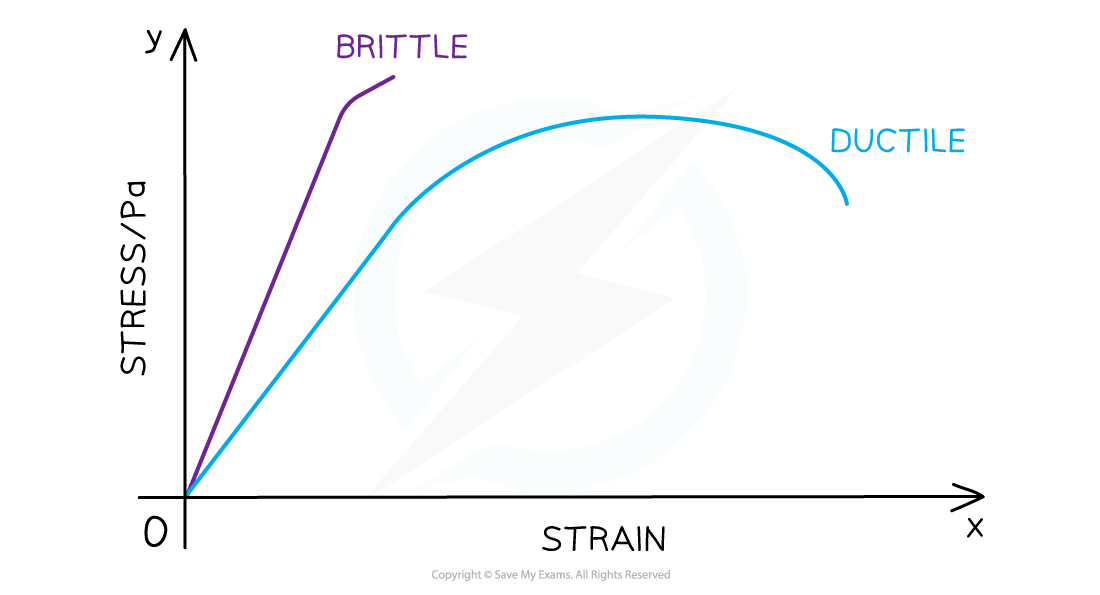
Stress-strain curve for a brittle and ductile material
Brittle and ductile materials can be identified on a stress-strain or force-extension graph:
A brittle material is represented by a straight line through the origin with no or negligible curved region
A ductile material is represented with a straight line through the origin then curving towards the x-axis
Worked Example
A sample of metal is subjected to a force which increases to a maximum value and then fractures. A force-extension graph for the sample is shown.

What is the behaviour of the metal at point X?
A. both elastic and plastic
B. not elastic and not plastic
C. plastic but not elastic
D. elastic but not plastic
Answer: D
The graph is a straight line at point X
Therefore, the force and extension are directly proportional
Point X is not beyond the elastic limit
So the metal is behaving elastically
Examiner Tips and Tricks
Although similar definitions, the elastic limit and limit of proportionality are not the same point on the graph. The limit of proportionality is the point beyond which the material is no longer defined by Hooke’s law. The elastic limit is the furthest point a material can be stretched whilst still able to return to its previous shape. This is at a slightly higher extension than the limit of proportionality. Be sure not to confuse them.

Unlock more, it's free!
Did this page help you?