Revision NotesExam QuestionsPast Papers
A LevelPhysicsCambridge (CIE)Revision Notes5. Work, Energy & PowerEnergy ConservationEfficiency

Syllabus Edition

First teaching 2023

First exams 2025

|

Efficiency (Cambridge (CIE) A Level Physics)

Revision Note

Leander Oates

Written by: Leander Oates

Reviewed by: Caroline Carroll

Updated on

Efficiency of a system

  • The efficiency of a system is the ratio of the useful energy output from the system to the total energy input

    • If a system has high efficiency, this means most of the energy transferred is useful

    • If a system has low efficiency, this means most of the energy transferred is wasted

  • Multiplying this ratio by 100 gives the efficiency as a percentage

  • The efficiency is calculated using the equation:

efficiency space equals fraction numerator space useful space energy space output over denominator total space energy space input end fraction space cross times space 100 percent sign

  • Efficiency can also be written in terms of power (the energy transferred per second):

efficiency space equals fraction numerator space useful space power space output over denominator total space power space input end fraction

Worked Example

An electric motor has an input power, Pin, useful output power, Pout, power lost Plost, and an efficiency η.

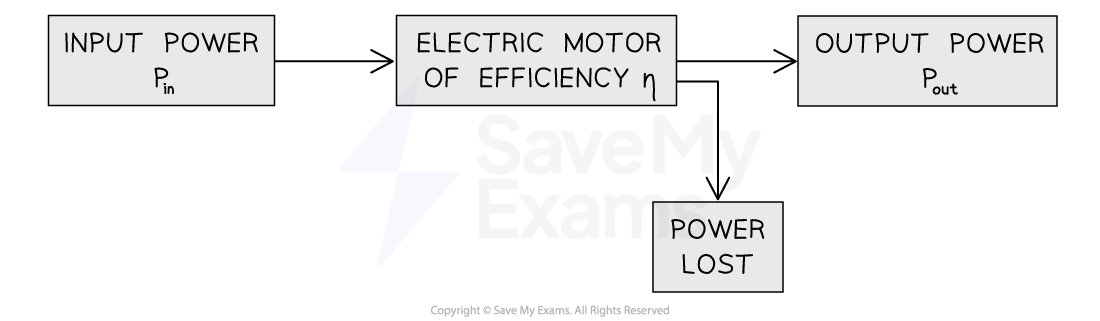
5-1-3-we-efficiency-of-a-system-question-cie-new

What is the output power of the motor? 

A. eta over P subscript i n end subscript      

B. fraction numerator negative eta P subscript l o s t end subscript over denominator eta minus 1 end fraction      

C. eta P subscript l o s t end subscript      

D. negative eta P subscript l o s t end subscript open parentheses eta minus 1 close parentheses

Answer: B

Step 1: State the efficiency equation

efficiency space equals fraction numerator space useful space power space output over denominator total space power space input end fraction

Step 2: Substitute the terms given in the question

eta space equals space P subscript o u t end subscript over P subscript i n end subscript space equals space fraction numerator P subscript o u t end subscript over denominator P subscript o u t space end subscript plus space P subscript l o s t end subscript end fraction

Step 3: Manipulate the equation

  • Multiply by Pout + Plost on both sides

eta open parentheses p subscript o u t end subscript space plus space p subscript l o s t end subscript close parentheses space equals space P subscript o u t end subscript

  • Expand the brackets

eta P subscript o u t end subscript space plus space eta P subscript l o s t end subscript space equals space P subscript o u t end subscript

  • Minus Pout from both sides

    • It is helpful to first minus ηPlost

eta P subscript o u t end subscript space equals space P subscript o u t end subscript space minus space eta P subscript l o s t end subscript

eta P subscript o u t end subscript space minus space P subscript o u t end subscript space equals space minus eta P subscript l o s t end subscript

  • Factor out Pout

P subscript o u t end subscript space open parentheses eta space minus space 1 close parentheses space equals space minus eta P subscript l o s t end subscript

  • Divide by η − 1

P subscript o u t end subscript space equals space fraction numerator negative eta P subscript l o s t end subscript over denominator open parentheses eta space minus space 1 close parentheses end fraction

Examiner Tips and Tricks

Efficiency can be in a ratio or percentage format. If the question asks for an efficiency as a ratio, give your answer as a fraction or decimal. If the answer is required as a percentage, remember to multiply the ratio by 100 to convert it, e.g. Ratio = 0.25, Percentage = 0.25 × 100 = 25 %

Solving problems involving efficiency

  • Efficiency calculations are often part of a larger multi-step problem

  • Since efficiency deals with energy and power, questions will often involve energy or power calculations 

Worked Example

The diagram shows a pump called a hydraulic ram.

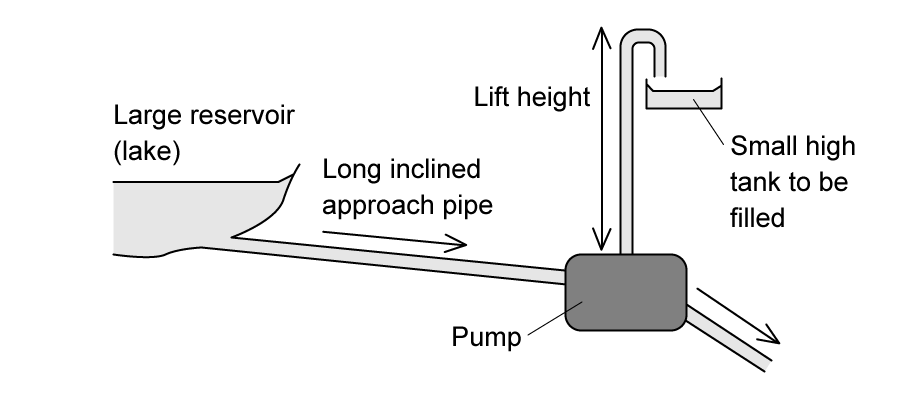
In one such pump, the long approach pipe holds 700 kg of water. A valve shuts when the speed of this water reaches 3.5 m s-1. The kinetic energy of this water is used to lift a small quantity of water by a height of 12m. The efficiency of the pump is 20%.

Which mass of water could be lifted 12 m?

A. 6.2 kg               

B. 4.6 kg               

C. 7.3 kg               

D. 0.24 kg

Answer: C

Step 1: List the known quantities

  • Mass of water in approach pipe = 700 kg

  • Speed of water in approach pipe, v = 3.5 m s-1

  • Height of lifted water, h = 12 m

Step 2: Consider the energy transfer taking place

  • Energy is transferred from the kinetic store of the water to its gravitational potential store

Step 3: Consider the efficiency of the energy transfer

  • The transfer is 20% efficient

  • Therefore, 20% of the kinetic input energy is output as gravitational potential energy

0.2 E subscript k space equals space E subscript p

0.2 space cross times 1 half m v squared space equals space m g h

Step 4: Calculate the mass of water lifted water

0.2 space cross times space 0.5 space cross times 700 space cross times open parentheses 3.5 close parentheses squared space equals space m space cross times space 9.81 space cross times space 12

857.5 space equals space m space cross times space 117.72

m space equals space fraction numerator 857.5 over denominator 117.72 end fraction

m space equals space 7.3 space kg

Examiner Tips and Tricks

Equations for kinetic and potential energies are important for these types of questions. Also, familiarise yourself with the different equations for power depending on what quantities are given.

You've read 0 of your 5 free revision notes this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

    Test yourself

    Did this page help you?

    Previous:The Principle of Conservation of EnergyNext:Power
    Author
    Reviewer
    Leander Oates

    Author: Leander Oates

    Expertise: Physics

    Leander graduated with First-class honours in Science and Education from Sheffield Hallam University. She won the prestigious Lord Robert Winston Solomon Lipson Prize in recognition of her dedication to science and teaching excellence. After teaching and tutoring both science and maths students, Leander now brings this passion for helping young people reach their potential to her work at SME.

    Caroline Carroll

    Author: Caroline Carroll

    Expertise: Physics Subject Lead

    Caroline graduated from the University of Nottingham with a degree in Chemistry and Molecular Physics. She spent several years working as an Industrial Chemist in the automotive industry before retraining to teach. Caroline has over 12 years of experience teaching GCSE and A-level chemistry and physics. She is passionate about creating high-quality resources to help students achieve their full potential.