Principle of Moments (Cambridge (CIE) A Level Physics) : Revision Note
The principle of moments
The principle of moments states:
For a system to be in equilibrium, the sum of clockwise moments about a point must be equal to the sum of the anticlockwise moments (about the same point)
Moments acting on a balanced beam
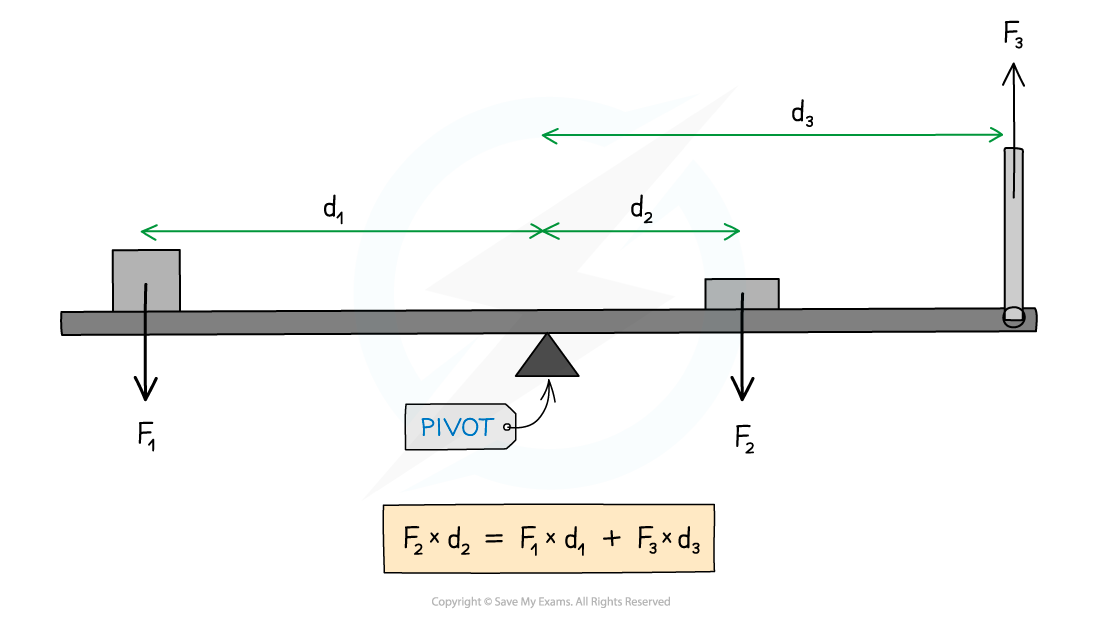
The sum of the anticlockwise moments of forces F1 and F3 are equal to the clockwise moment of force F2
In the above diagram:
Force F2 is supplying a clockwise moment;
Forces F1 and F3 are supplying anticlockwise moments
The clockwise and anticlockwise moments are equal because the beam is in equilibrium
Worked Example
A uniform beam of weight 40 N is 5 m long and is supported by a pivot situated 2 m from one end.
When a load of weight W is hung from that end, the beam is in equilibrium as shown in the diagram.

What is the value of W?
A. 10 N
B. 50 N
C. 25 N
D. 30 N
Answer:
Step 1: List the known quantities
Weight acting on beam, WB = 40 N
Weight acting on mass = W
Length of beam = 5 m
Distance to pivot (from end of beam), dW = 2 m
Step 2: Recall the principle of moments
clockwise moments = anticlockwise moments
Step 3: Calculate the clockwise moment
Because the beam is uniform, the force of weight acting upon it will be exerted from its centre of gravity
This will be the middle of the beam
(from the end of the beam)
The pivot is 2 m from the end of the beam
Therefore, the force acts at a distance of 2.5 − 2 = 0.5 m from the pivot
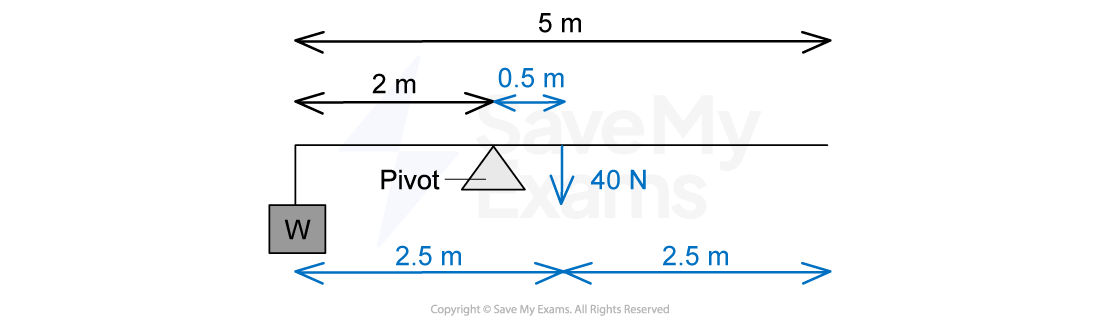
Step 4: Calculate the anticlockwise moment
Step 5: Equate the clockwise and anticlockwise moments to calculate
Therefore, the answer is A
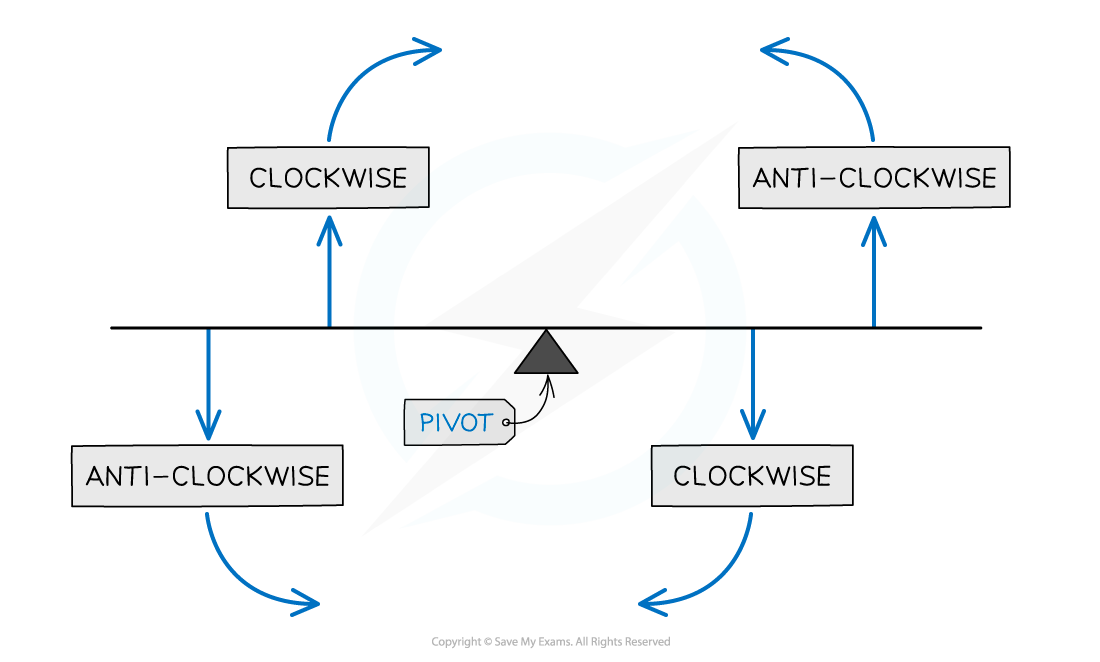
Examiner Tips and Tricks
Make sure that all the distances are in the same units and you’re considering the correct forces as clockwise or anticlockwise, as seen in the diagram below

You've read 0 of your 5 free revision notes this week
Sign up now. It’s free!
Did this page help you?

