Revision NotesExam QuestionsPast Papers
A LevelPhysicsCIERevision Notes24. Medical Physics24.2 Production & Use of X-raysAttenuation of X-rays in Matter

Syllabus Edition

First teaching 2023

First exams 2025

|

Attenuation of X-rays in Matter (CIE A Level Physics)

Revision Note

Ashika

Author

Ashika

Last updated

Attenuation of X-rays in matter

  • Bones absorb X-ray radiation
    • This is why they appear white on the X-ray photograph
  • When the collimated beam of X-rays passes through the patient’s body, some of the photons are absorbed and scattered
  • The attenuation of X-rays can be calculated using the equation:

I space equals space I subscript 0 e to the power of negative mu x end exponent

  • Where:
    • I0 = the intensity of the incident beam (W m-2)
    • I = the intensity of the emergent beam (W m-2)
    • μ = the linear absorption coefficient (m-1)
    • x = distance travelled through the material (m)
  • The attenuation coefficient also depends on the energy of the X-ray photons
  • The intensity of the X-ray decays exponentially
  • The thickness of the material that will reduce the X-ray beam or a particular frequency to half its original value is known as the half thickness

Intensity-distance graph of X-rays for air and body

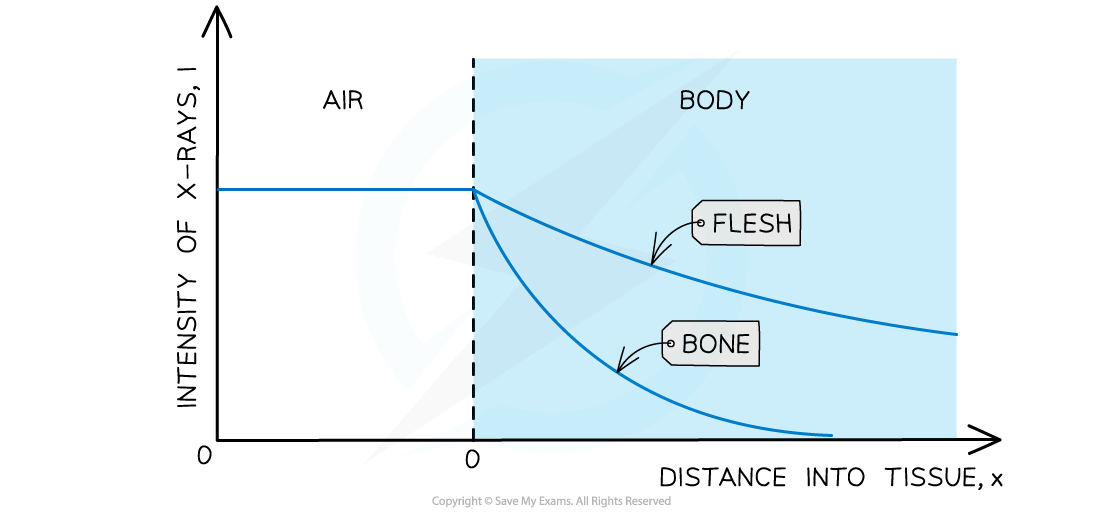
Attenuation of X-rays, downloadable AS & A Level Physics revision notes

Absorption of X-rays by different materials

Worked example

A student investigates the absorption of X-ray radiation in a model arm. A cross-section of the model arm is shown in the diagram.

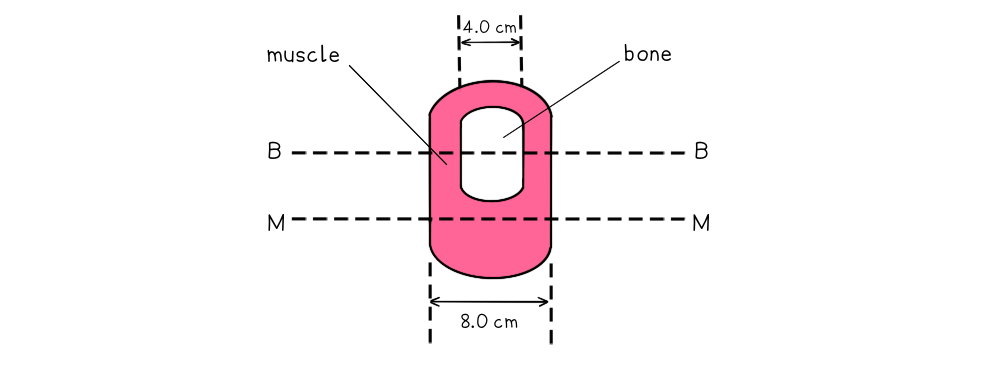
Parallel X-ray beams are directed along the line MM and along the line BB. The linear absorption coefficients of the muscle and the bone are 0.20 cm-1 and 12 cm-1 respectively.

Calculate the ratio:

fraction numerator intensity space of space emergent space straight x minus ray space beam space from space model space over denominator intensity space of space incident space straight x minus ray space beam space on space model end fraction

for a parallel X-ray beam directed along the line

(a) MM

(b) BB

and state whether the X-ray images are sharp, or have good contrast.

Answer:

Part (a)

Step 1: Write out the known quantities

  • Linear absorption coefficient for muscle, μ = 0.20 cm-1
  • Distance travelled through the muscle, x = 8.0 cm

Step 2: Write out the equation for attenuation and rearrange

I space equals space I subscript 0 e to the power of negative mu x end exponent

fraction numerator intensity space of space emergent space straight x minus ray space beam space from space model over denominator intensity space of space incident space straight x minus ray space beam space on space model end fraction space equals fraction numerator space I over denominator I subscript 0 end fraction space equals space e to the power of negative mu x end exponent

Step 3: Substitute in values and calculate the ratio

I over I subscript 0 space equals space e to the power of negative open parentheses 0.20 space cross times space 8 close parentheses end exponent space equals space 0.2

Part (b)

Step 1: Write out the known quantities

  • Linear absorption coefficient for muscle, μm = 0.20 cm-1
  • Linear absorption coefficient for bone, μb = 12 cm-1
  • Distance travelled through the muscle, xm = 4.0 cm
  • Distance travelled through the bone, xb = 4.0 cm

Step 2: Write out the equation for attenuation for two media and rearrange

I over I subscript 0 space equals space e to the power of negative mu subscript m x subscript m end exponent space cross times space e to the power of negative mu subscript b x subscript b end exponent

Step 3: Substitute in values and calculate the ratio

I over I subscript 0 space equals space e to the power of negative open parentheses 0.20 space cross times space 4 close parentheses end exponent space cross times space e to the power of negative open parentheses 12 space cross times space 4 close parentheses end exponent space equals space 6.4 space cross times space 10 to the power of negative 22 end exponent space almost equal to space 0

Step 4: Write a concluding statement

  • Each ratio gives a measure of the amount of transmission of the beam
  • A good contrast is when:
    • there is a large difference between the intensities
    • the ratio is much less than 1.0
  • Therefore, both images have a good contrast

You've read 0 of your 5 free revision notes this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

    Test yourself

    Did this page help you?

    Previous:Production & Use of X-raysNext:Computed Tomography Scanning
    Ashika

    Author: Ashika

    Expertise: Physics Project Lead

    Ashika graduated with a first-class Physics degree from Manchester University and, having worked as a software engineer, focused on Physics education, creating engaging content to help students across all levels. Now an experienced GCSE and A Level Physics and Maths tutor, Ashika helps to grow and improve our Physics resources.