Revision NotesExam QuestionsPast Papers
A LevelPhysicsCIERevision Notes20. Magnetic Fields20.5 Electromagnetic InductionFaraday's & Lenz's Laws

Syllabus Edition

First teaching 2023

First exams 2025

|

Faraday's & Lenz's Laws (CIE A Level Physics)

Revision Note

Ashika

Author

Ashika

Last updated

Faraday's & Lenz's laws

  • Faraday’s law tells us the magnitude of the induced e.m.f in electromagnetic induction and is defined as:

The magnitude of the induced e.m.f is directly proportional to the rate of change in magnetic flux linkage

  • Faraday's law of induction is defined by the equation:

epsilon space equals space N fraction numerator increment capital phi over denominator increment t end fraction

  • Where:
    • ε = induced e.m.f (V)
    • N = number of turns of coil
    • ΔΦ = change in magnetic flux (Wb)
    • Δt = time interval (s)
  • Lenz’s Law gives the direction of the induced e.m.f as defined by Faraday’s law:

The induced e.m.f acts in such a direction to produce effects which oppose the change causing it

  • Lenz’s law combined with Faraday’s law is:

epsilon space equals space minus N fraction numerator increment capital phi over denominator increment t end fraction

  • This equation shows:
    • When a bar magnet goes through a coil, an e.m.f is induced within the coil due to a change in magnetic flux
    • A current is also induced which means the coil now has its own magnetic field
    • The coil’s magnetic field acts in the opposite direction to the magnetic field of the bar magnet
  • If a direct current (d.c) power supply is replaced with an alternating current  (a.c) supply, the e.m.f induced will also be alternating with the same frequency as the supply

Experimental evidence for Lenz’s law

  • To verify Lenz’s law, the only apparatus needed is:
    • a bar magnet
    • a coil of wire
    • a sensitive ammeter
  • Note: a cell is not required
  • A known pole (either north or south) of the bar magnet is pushed into the coil, which induces a magnetic field in the coil
    • Using the right hand grip rule, the curled fingers indicate the direction of the current and the thumb indicates the direction of the induced magnetic field
  • The direction of the current is observed on the ammeter
    • Reversing the magnet direction would give an opposite deflection on the meter
  • The induced field (in the coil) repels the bar magnet
  • This is because of Lenz’s law:
    • The direction of the induced field in the coil pushes against the change creating it, i.e. the bar magnet

Lenz's law

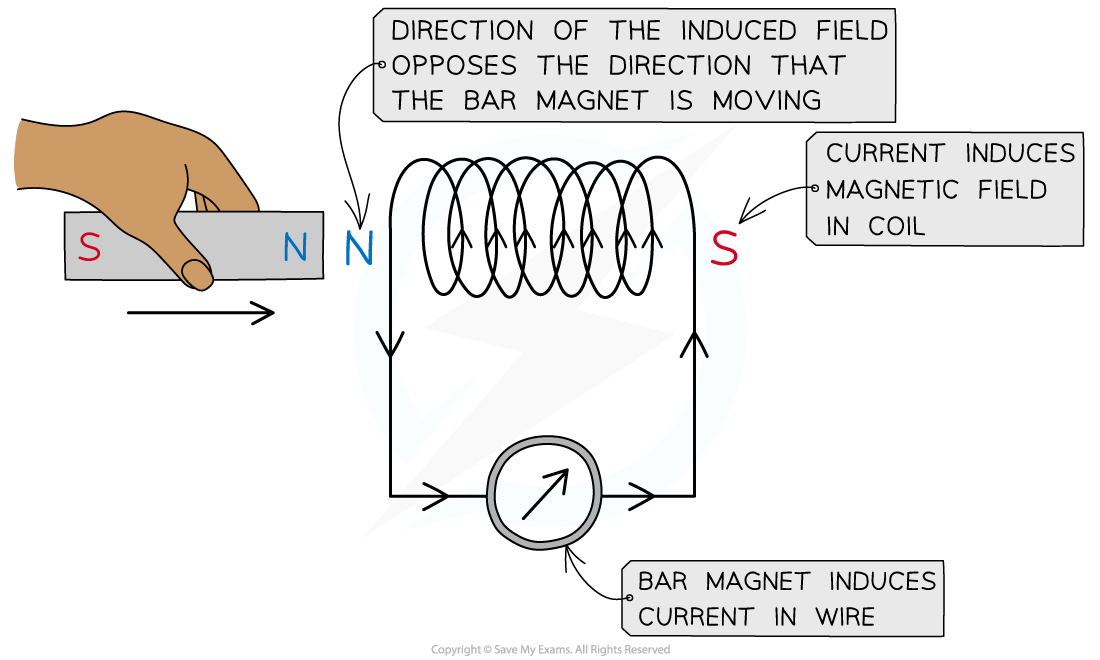
20-2-lenzs-law-experiment-1

Lenz’s law can be verified using a coil connected in series with a sensitive ammeter and a bar magnet

Worked example

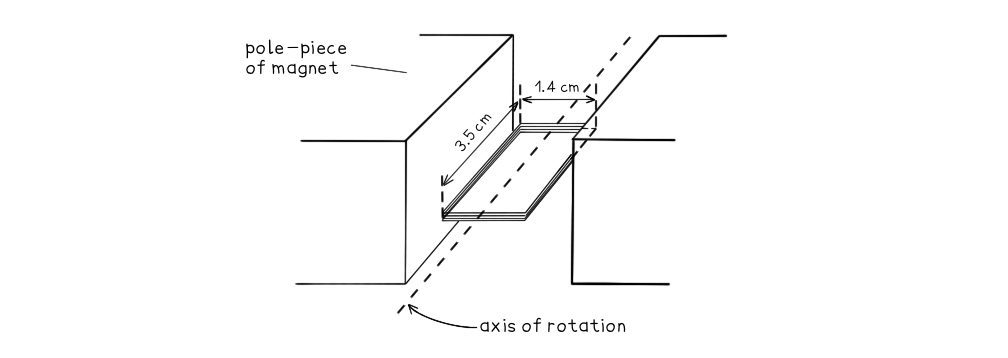
A small rectangular coil contains 350 turns of wire. The longer sides are 3.5 cm and the shorter sides are 1.4 cm.The coil is held between the poles of a large magnet so that the coil can rotate about an axis through its centre.The magnet produces a uniform magnetic field of flux density 80 mT between its poles. The coil is positioned horizontally and then turned through an angle of 40° in a time of 0.18 s.

Calculate the magnitude of the average e.m.f induced in the coil.

Answer:

Step 1: Write down the known quantities

  • Magnetic flux density, B = 80 mT = 80 × 10-3 T
  • Area, A = 3.5 × 1.4 = (3.5 × 10-2) × (1.4 × 10-2) = 4.9 × 10-4 m2
  • Number of turns, N = 350
  • Time interval, Δt = 0.18 s
  • Angle between coil and field lines, θ = 40o

 Step 2: Write out the equation for Faraday’s law:

epsilon space equals space N fraction numerator increment capital phi space over denominator increment t end fraction

Step 3: Write out the equation for flux linkage

N capital phi space equals space B A N cos open parentheses theta close parentheses

Step 4: Substitute values into flux linkage equation:

N capital phi space equals space open parentheses 80 space cross times 10 to the power of negative 3 end exponent close parentheses space cross times space open parentheses 4.9 space cross times space 10 to the power of negative 4 end exponent close parentheses space cross times space 350 space cross times space cos open parentheses 40 close parentheses space equals space 0.0105 space Wb space turns

Step 5: Substitute flux linkage and time into Faraday’s law equation

epsilon space equals space fraction numerator 0.0105 space over denominator 0.18 end fraction space equals space 0.05839 space equals space 58 space mV space open parentheses 2 space straight s. straight f. close parentheses

You've read 0 of your 5 free revision notes this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

    Test yourself

    Did this page help you?

    Previous:Principles of Electromagnetic InductionNext:Alternating Current & Voltage
    Ashika

    Author: Ashika

    Expertise: Physics Project Lead

    Ashika graduated with a first-class Physics degree from Manchester University and, having worked as a software engineer, focused on Physics education, creating engaging content to help students across all levels. Now an experienced GCSE and A Level Physics and Maths tutor, Ashika helps to grow and improve our Physics resources.