Revision NotesExam QuestionsPast Papers
A LevelPhysicsCambridge (CIE)Revision Notes20. Magnetic FieldsForce on a Moving ChargeVelocity Selection

Syllabus Edition

First teaching 2023

First exams 2025

|

Velocity Selection (Cambridge (CIE) A Level Physics)

Revision Note

Ashika

Author

Ashika

Last updated

Velocity selection

  • A velocity selector is defined as:

    A device consisting of perpendicular electric and magnetic fields where charged particles with a specific velocity can be filtered

  • Velocity selectors are used in devices, such as mass spectrometers, to produce a beam of charged particles all travelling at the same velocity

  • A velocity selector consists of two oppositely charged parallel plates situated in a vacuum chamber

    • The plates provide a uniform electric field with strength E between them

  • There is also a uniform magnetic field with flux density B applied perpendicular to the electric field

    • If a beam of charged particles enters between the plates, they may all have the same charge Q but travel at different speeds

Velocity selector

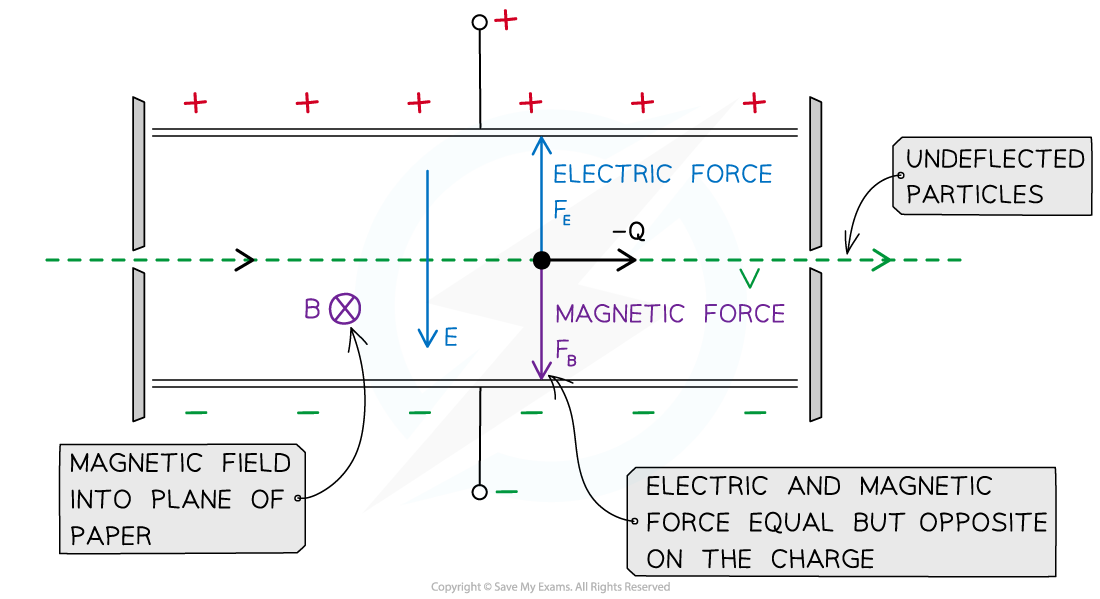
Velocity selection diagram, downloadable AS & A Level Physics revision notes

The particles travelling at the desired speed v will travel through undeflected due to the equal and opposite electric and magnetic forces on them

  • The electric force does not depend on the velocity: F subscript E space equals space E Q

  • However, the magnetic force does depend on the velocity: F subscript B space equals space B Q v

    • The magnetic force will be greater for particles which are travelling faster

  • To select particles travelling at exactly the desired speed v, the electric and magnetic force must therefore be equal, but in opposite directions

F subscript E space equals space F subscript B

  • The resultant force on the particles at speed v will be zero, so they will remain undeflected and pass straight through between the plates

  • By equating the electric and magnetic force equations:

E Q space equals space B Q v

  • The charge Q will cancel out on both sides to give the selected velocity v equation:

v space equals fraction numerator space E over denominator B end fraction

  • Therefore, the speed v in which a particle will remain undeflected is found by the ratio of the electric and magnetic field strength

    • If a particle has a speed greater or less than v, the magnetic force will deflect it and collide with one of the charged plates

    • This would remove the particles in the beam that are not exactly at speed v

  • Note: the gravitational force on the charged particles will be negligible compared to the electric and magnetic forces and therefore can be ignored in these calculations

Worked Example

A positive ion travels between two charged plates towards a slit S

(a) State the direction of the electric and magnetic fields on the ion

(b) Calculate the speed of the ion emerging from slit S when the magnetic flux density is 0.50 T and the electric field strength is 2.8 kV m-1

(c) Which plate will the ion be deflected towards if the speed was greater than the speed in part (b)

Answer:

Part (a)

Step 1: Determine the direction of E field

  • Electric field lines point from the positive to negative to charge

  • Therefore, it must be directed vertically upwards

Step 2: Determine the direction of B field

  • Using Fleming’s left-hand rule:

    • The charge, or current I, is directed to the right

    • B is out of the page

    • Therefore, the force F is vertically downwards

Part (b)

  • Electric field strength, E = 2.8 kV m-1 = 2.8 × 103 V m-1

  • Magnetic flux density, B = 0.50 T

v space equals fraction numerator space E over denominator B end fraction space equals space fraction numerator 2.8 space cross times space 10 cubed space over denominator 0.50 end fraction space equals space 5600 space straight m space straight s to the power of negative 1 end exponent

Part (c)

  • If the speed increases, the magnetic force must be greater because FB ∝ v

  • Since the magnetic force would direct the ion downwards in the direction of the field, the ion will be deflected towards the positive plate

You've read 0 of your 5 free revision notes this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

    Test yourself

    Did this page help you?

    Previous:Motion of a Charged Particle in a Magnetic FieldNext:Magnetic Fields in Wires, Coils & Solenoids
    Ashika

    Author: Ashika

    Expertise: Physics Project Lead

    Ashika graduated with a first-class Physics degree from Manchester University and, having worked as a software engineer, focused on Physics education, creating engaging content to help students across all levels. Now an experienced GCSE and A Level Physics and Maths tutor, Ashika helps to grow and improve our Physics resources.