Revision NotesExam QuestionsPast Papers
A LevelPhysicsCIERevision Notes2. Kinematics2.1 Equations of MotionSolving Problems with Kinematic Equations

Syllabus Edition

First teaching 2023

First exams 2025

|

Solving Problems with Kinematic Equations (CIE A Level Physics)

Revision Note

Ashika

Author

Ashika

Last updated

Solving problems with kinematic equations

  • Solving problems with kinematic equations can be broken down into simple steps

  • Step 1: Write out the variables that are given in the question, both known and unknown, and use the context of the question to deduce any quantities that aren’t explicitly given
    • e.g. for vertical motion a = ± 9.81 m s–2, an object which starts or finishes at rest will have u = 0 or v = 0

  • Step 2: Choose the equation which contains the quantities you have listed
    • e.g. the equation that links s, u, a and t is s = ut + ½at2

  • Step 3: Convert any units to SI units and then insert the quantities into the equation and rearrange algebraically to determine the answer

Worked example

The diagram shows an arrangement to stop trains that are travelling too fast.

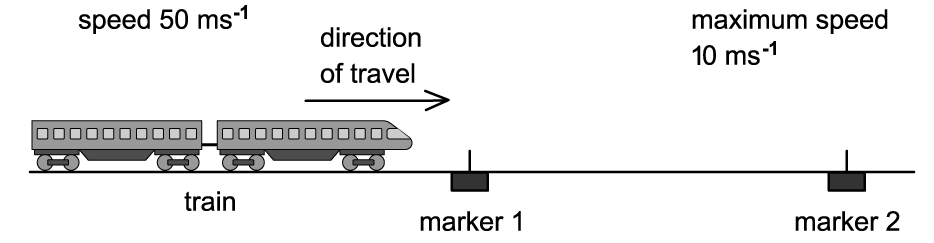
SUVAT Problems Worked Example (1), downloadable AS & A Level Physics revision notes

Trains coming from the left travel at a speed of 50 ms-1.

At marker 1, the driver must apply the brakes so that the train decelerates uniformly in order to pass marker 2 at no more than 10 ms-1. The train carries a detector that notes the times when the train passes each marker and will apply an emergency brake if the time between passing marker 1 and marker 2 is less than 20 s.

Suggest how far from marker 2 that marker 1 should be placed.

Answer:
Step 1: List the known quantities

  • u = 50 m s–1
  • v = 10 m s–1
  • t = 20 s
  • s = ?

Step 2: State the appropriate equation

  • The equation that links u, v, t and s are:

s space equals fraction numerator space open parentheses u space plus thin space v close parentheses over denominator 2 end fraction t

Step 3: Substitute in the values

s space equals space fraction numerator open parentheses 50 space plus space 10 close parentheses space over denominator 2 end fraction space cross times space 20 space equals space 600 space straight m

Examiner Tip

This is arguably the most important section of this topic, you can always be sure there will be one, or more, questions in the exam about solving problems with the kinematic equations. Make sure you've correctly selected the final and initial velocity.

You've read 0 of your 5 free revision notes this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

    Test yourself

    Did this page help you?

    Previous:Deriving Kinematic EquationsNext:Acceleration of Free Fall Experiment
    Ashika

    Author: Ashika

    Expertise: Physics Project Lead

    Ashika graduated with a first-class Physics degree from Manchester University and, having worked as a software engineer, focused on Physics education, creating engaging content to help students across all levels. Now an experienced GCSE and A Level Physics and Maths tutor, Ashika helps to grow and improve our Physics resources.