Electric Potential Gradient (Cambridge (CIE) A Level Physics)

Potential gradient

• An electric field can be described in terms of the variation of electric potential at different points in the field

  • This is known as the potential gradient

• The potential gradient of an electric field is defined as:

  The rate of change of electric potential with respect to displacement in the direction of the field

• A graph of potential V against distance r can be drawn for a positive or negative charge Q

• This is a graphical representation of the equation:

• The electric field strength at a point is equivalent to the negative electric potential gradient at that point

• This can be written mathematically as:

• Where:

  • E = electric field strength (V m⁻¹)

  • ΔV = potential difference between two points (V)

  • Δr = displacement in the direction of the field (m)

• Hence,  = electric potential gradient, i.e. the change in potential with distance

• The negative sign is included to indicate that the potential gradient acts in the opposite direction to the field strength and electric force

• Therefore, as distance from the point charge increases:

  • field strength and force decreases

  • potential gradient increases

Graph of electric potential and distance

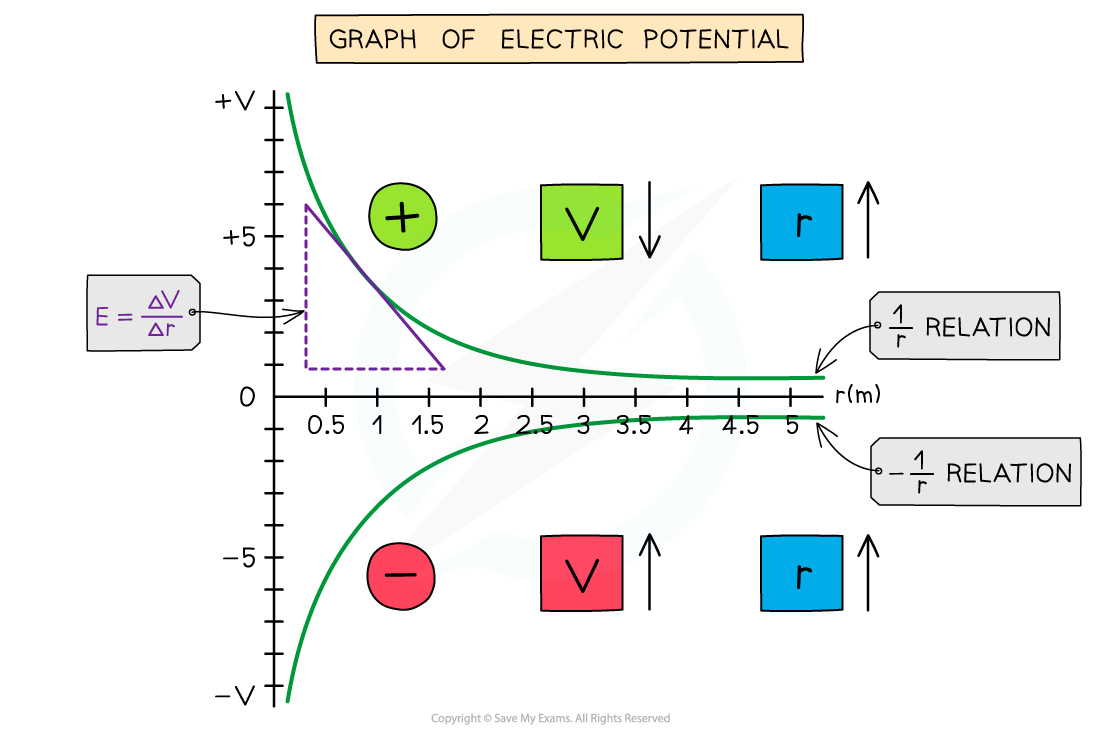
The electric potential around a positive charge decreases with distance and increases with distance around a negative charge

• The key features of this graph are:

  • All values of potential are negative for a negative charge

  • All values of potential are positive for a positive charge

  • As r increases, V against r follows a 1/r relation for a positive charge and a -1/r relation for a negative charge

  • The gradient of the graph at any particular point is equal to the field strength E at that point

  • The curve is shallower than the corresponding E-r graph

Determining potential difference from a field-distance graph

• The potential difference due to a charge can also be determined from the area under a field-distance graph

• A graph of field strength E against distance r can be drawn for a positive or negative charge Q

• This is a graphical representation of the equation:

• The area under the E-r graph between two points is equal to the potential difference ΔV between those points

Graph of electric field strength and distance

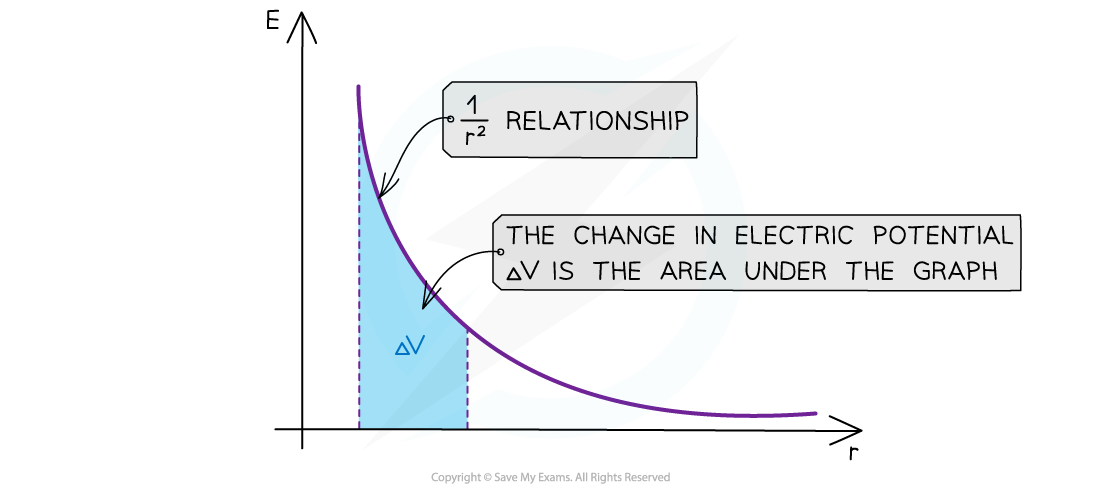
The electric field strength E has a 1/r² relationship, and the area under the graph represents change in electric potential

• The key features of this graph are:

  • All values of field strength are negative for a negative charge

  • All values of field strength are positive for a positive charge

  • As r increases, E against r follows a 1/r² relation (inverse square law)

  • The area under this graph is the change in electric potential ΔV

  • The curve is steeper than the corresponding V-r graph