Electric Potential

The electric potential at a point is defined as:

The work done per unit charge in taking a small positive test charge from infinity to a defined point

Electric potential is measured in J C⁻¹ or V
It is a scalar quantity but has a positive or negative sign to indicate the sign of the charge

In a similar way to gravitational potential, electric potential also has a value of zero at infinity

The electric potential at a point depends on:
- the magnitude of the point charge
- the distance between the charge and the point

Electric potential for a positive charge

Around an isolated positive charge, electric potential:
- has a positive value
- increases when a test charge moves closer
- decreases when a test charge moves away

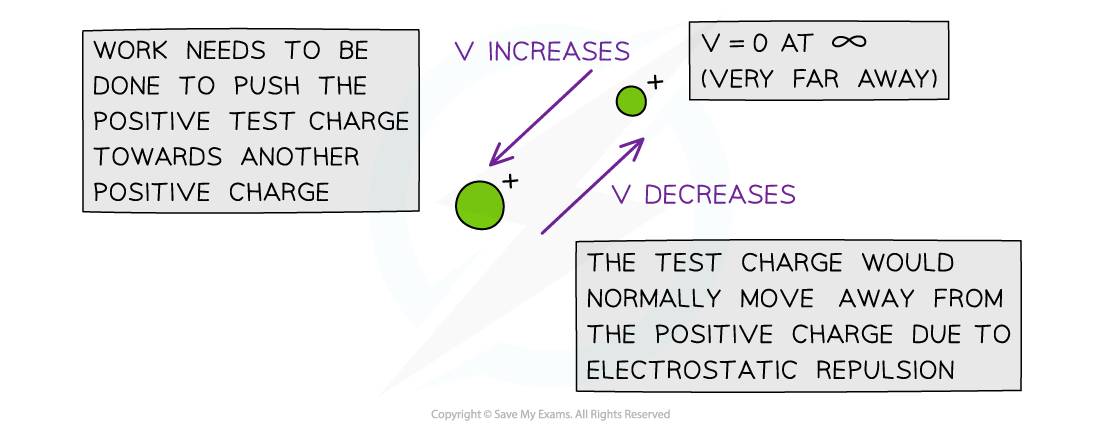
Electric Potential around Positive & Negative Charges 1, downloadable AS & A Level Physics revision notes

For a positive charge, the electric potential decreases in the direction a positive test charge would move in due to the electrostatic repulsion

Electric potential for a negative charge

Around an isolated negative charge, electric potential:
- has a negative value
- decreases when a test charge moves closer
- increases when a test charge moves away

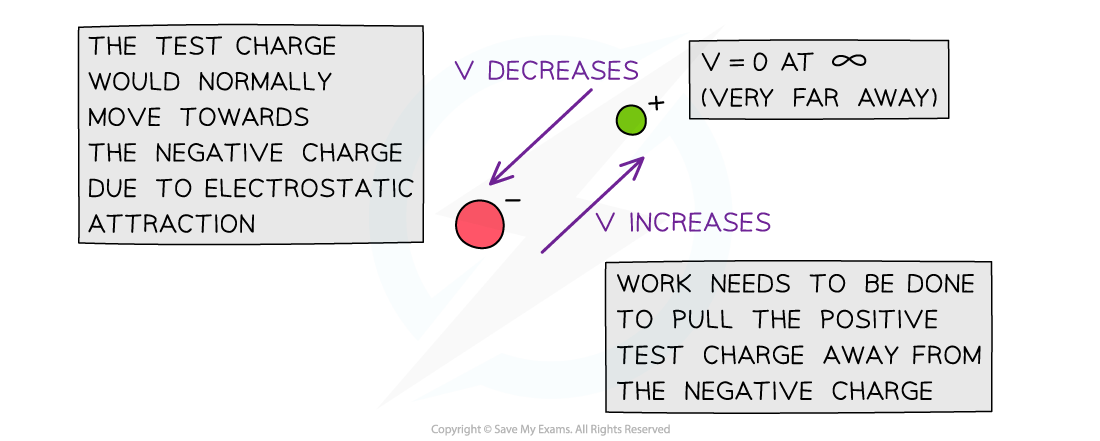
Electric Potential around Positive & Negative Charges 2, downloadable AS & A Level Physics revision notes

For a negative charge, the electric potential decreases in the direction a positive test charge would move in due to the electrostatic attraction

Examiner Tip

One way to remember whether the electric potential increases or decreases with respect to the distance from the charge is by the direction of the electric field lines. The potential always decreases in the same direction as the field lines and vice versa.

Electric potential due to a point charge

The electric potential due to a point charge can be calculated using:

$V = \frac{Q}{4 π ε_{0} r}$

Where:
- V = electric potential (V)
- Q = magnitude of the charge producing the potential (C)
- r = distance from the centre of the point charge (m)
- ε₀ = permittivity of free space (F m⁻¹)

For a positive (+) charge:
- potential V_e increases as the separation r decreases
- energy must be supplied to a positive test charge to overcome the repulsive force

For a negative (−) charge:
- potential V_e decreases as the separation r increases
- energy is released as a positive test charge moves in the direction of the attraction force

The electric potential has an inversely proportional relationship with distance
Unlike gravitational potential which is always negative, the sign of the charge corresponds to the sign of the electric potential

Note: this equation also applies to a conducting sphere. The charge on the sphere is treated as if it is concentrated at the centre of the sphere, i.e. like a point charge

Graph of potential against distance for a positive charge

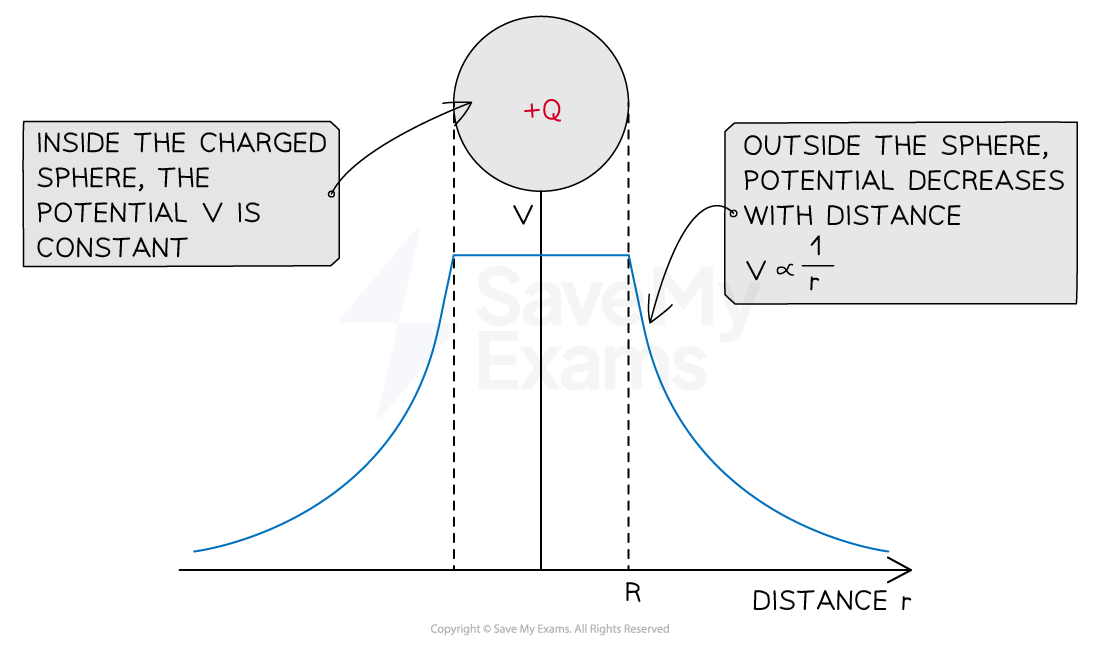
electric-potential-around-charged-sphere

Electric potential is constant inside a charged sphere and decreases with distance outside the sphere

Combining electric potentials

To find the potential at a point caused by multiple charges, each potential can be combined by addition
For example, the combined potential of two point charges at a point is:

$V = \frac{Q_{1}}{4 π ε_{0} r_{1}} + \frac{Q_{2}}{4 π ε_{0} r_{2}}$

Where:
- Q₁, Q₂ = magnitude of the charges (C)
- r₁, r₂ = distance between each charge and the point (m)

How to calculate total electric potential

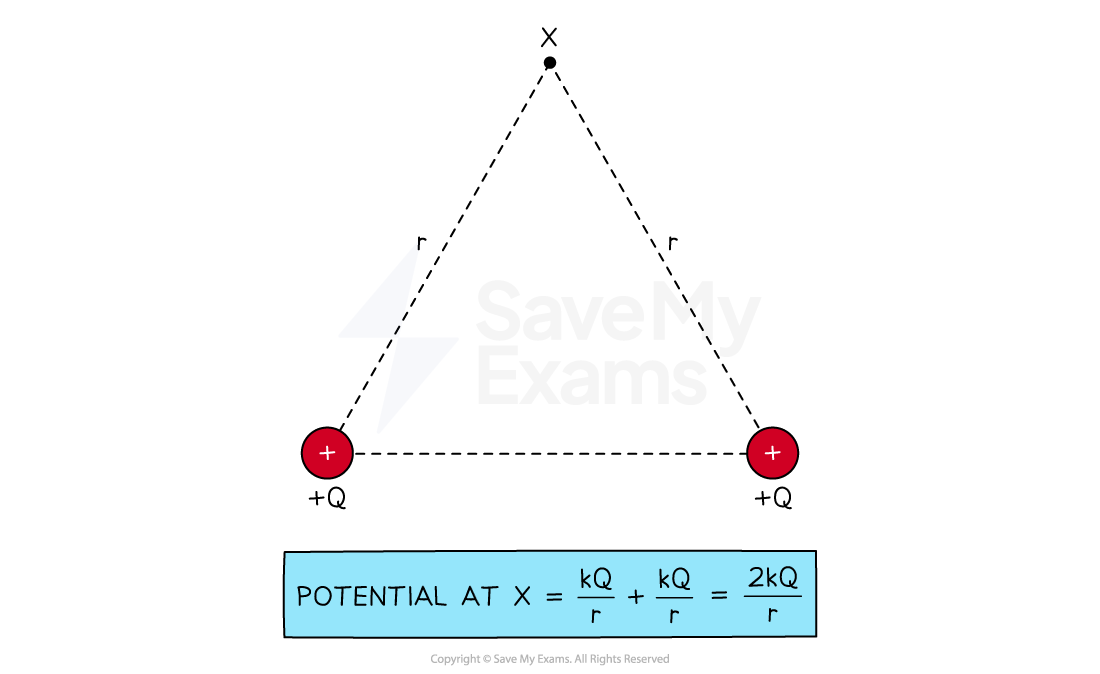
4-2-7-combined-potential-due-to-two-charges

Point X makes an equilateral triangle of length r with two equal positive charges Q. The combined potential of both charges at X is double the potential due to one of the charges

Worked example

A Van de Graaff generator has a spherical dome of radius 15 cm. It is charged up to a potential of 240 kV.

Calculate

(a)

the charge stored on the dome

(b)

the potential at a distance of 30 cm from the dome's surface

Answer:

Part (a)

Step 1: List down the known quantities

Radius of the dome, r = 15 cm = 15 × 10⁻² m
Potential difference, V = 240 kV = 240 × 10³ V
Permittivity of free space, ε₀ = 8.85 × 10⁻¹² F m⁻¹

Step 2: Write down the equation for the electric potential due to a point charge

$V = \frac{Q}{4 π ε_{0} r}$

Step 3: Rearrange for charge Q

$Q = 4 π ε_{0} r V$

Step 4: Substitute in values

Q = (240 × 10³) × (4π × 8.85 × 10⁻¹²) × (15 × 10⁻²) = 4.0 × 10⁻⁶ C = 4.0 μC

Part (b)

Step 1: Write down the known quantities

Charge stored in the dome, Q = 4.0 × 10⁻⁶ C
Distance, r = radius of the dome + distance from the dome = 15 + 30 = 45 cm = 0.45 m
- Note: we are treating the Van de Graaff as a point charge, so we take the distance from the centre of the dome

Step 2: Write down the equation for electric potential due to a point charge

$V = \frac{Q}{4 π ε_{0} r}$

Step 3: Substitute in values

$V = \frac{4.0 \times 10^{- 6}}{4 π \times (8.85 \times 10^{- 12}) \times 0.45} = 79.9 \times 10^{3} = 80$ kV (2 s.f.)

Electric Potential (CIE A Level Physics)

Revision Note

Electric potential

Electric potential for a positive charge

Electric potential for a negative charge

Examiner Tip

Electric potential due to a point charge

Graph of potential against distance for a positive charge

Combining electric potentials

How to calculate total electric potential

Worked example

You've read 0 of your 10 free revision notes

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

Author: Ann H