Electric Force Between Two Point Charges | CIE A Level Physics Revision Notes 2025

Point charge approximation

When calculating the force between two charges, air is treated as a vacuum
- This is why the permittivity of free space ε₀ is used

For a point outside a spherical conductor, the charge of the sphere may be considered to be a point charge at its centre
- A uniform spherical conductor is one where its charge is distributed evenly

The electric field lines around a spherical conductor are therefore identical to those around a point charge
- An example of a spherical conductor is a charged sphere

The field lines are radial and their direction depends on the charge of the sphere
- If the spherical conductor is positively charged, the field lines are directed away from the centre of the sphere
- If the spherical conductor is negatively charged, the field lines are directed towards the centre of the sphere

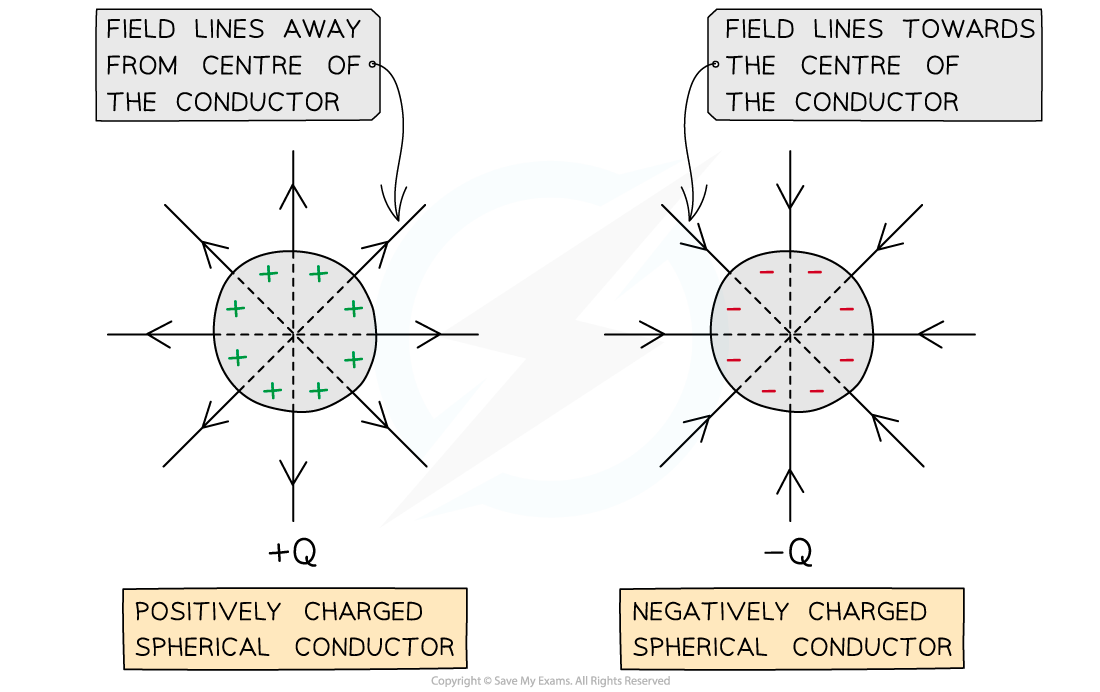
Point charge field lines, downloadable AS & A Level Physics revision notes

Electric field lines around a uniform spherical conductor are identical to those on a point charge

Examiner Tip

You might have noticed that the electric fields share many similarities to the gravitational fields. The main difference being the gravitational force is always attractive, whilst electrostatic forces can be attractive or repulsive.

You should make a list of all the similarities and differences you can find, as this could come up in an exam question.

Coulomb's law

All charged particles generate an electric field
- This field exerts a force on charged particles which are nearby

The electric force between two charges is defined by Coulomb’s law, which states that:

The force between two point charges is directly proportional to the product of the charges and inversely proportional to the square of the distance between them

This electric force can be calculated using the expression:

$F = \frac{Q_{1} Q_{2}}{4 π ε_{0} r^{2}}$

Where:
- F = electric force between two charges (N)
- Q₁, Q₂ = magnitudes of the charges (C)
- r = distance between the centres of the two charges (m)
- ε₀ = permittivity of free space

Electrostatic attraction between two charges

Coulombs Law diagram, downloadable AS & A Level Physics revision notes

The attractive electric force F_E between two point charges +Q and −Q with a separation of r is defined by Coulomb’s law

The constant ε₀ is the permittivity of free space
- ε₀ = 8.85 × 10^–12 C² N^–1 m^–2 and refers to charges in a vacuum
- The value of the permittivity of air is taken to be the same as ε₀
- All other materials have a higher permittivity ε > ε₀
- ε is a measure of the resistance offered by a material in creating an electric field within it

Sometimes Coulomb's law may be written as

$F = k \frac{Q_{1} Q_{2}}{r^{2}}$

Where $k = \frac{1}{4 π ε_{0}}$ is the Coulomb constant
The value of k depends on the material between the charges
- In a vacuum, k = 8.99 × 10⁹ N m² C^–2

Repulsive & attractive forces

Unlike the gravitational force between two masses which is only attractive, electric forces can be:
- attractive (the charges move closer together)
- repulsive (the charges move further apart)

Attraction and Repulsion

Opposite charges attract, like charges repel

Between two charges of the same type:
- The product Q₁Q₂ is positive, so the electrostatic force is positive
- A positive force means the charges experience repulsion

For two opposite charges:
- The product Q₁Q₂ is negative, so the electrostatic force is negative
- A negative force means the charges experience attraction

Worked example

An alpha particle is placed 2.0 mm from a gold nucleus in a vacuum.

Taking them as point charges, calculate the magnitude of the electric force acting between the nuclei.

Proton number of helium = 2
Proton number of gold = 79

Answer:

Step 1: Write down the known quantities

Separation between charges, r = 2.0 mm = 2.0 × 10^–3 m
Elementary charge, e = 1.60 × 10^–19 C (from the data booklet)
Permittivity of free space, ε₀ = 8.85 × 10^–12 F m^–2 (from the data booklet)

Step 2: Calculate the charges of the alpha particle and gold nucleus

An alpha particle (helium nucleus) has 2 protons, hence it has a charge of:

Q₁ = 2e = 2 × (1.60 × 10^–19)

A gold nucleus has 79 protons, hence it has a charge of:

Q₂ = 79e = 79 × (1.60 × 10^–19)

Step 3: Write down Coulomb's law

$F = \frac{Q_{1} Q_{2}}{4 π ε_{0} r^{2}}$

Step 4: Substitute the values and calculate the magnitude of the electric force

$F = \frac{2 \times 79 \times {(1.60 \times 10^{- 19})}^{2}}{4 π \times (8.85 \times 10^{- 12}) \times {(2.0 \times 10^{- 3})}^{2}} = 9.1 \times 10^{- 21}$ N (2 s.f.)

Examiner Tip

You do not need to memorise the value of the permittivity of free space ε₀, nor the Coulomb constant 1/4πε₀, as these are both given on the data sheet.

Unless specified in the question, you should assume that charges are located in a vacuum.

You should note that Coulomb's law can only be applied to charged spheres whose size is much smaller than their separation. Only in this case, the point charge approximation is valid. You must remember that the separation r must be taken from the centres of the spheres.

You cannot use Coulomb's law to calculate the force between charges distributed on irregularly shaped objects.

Electric Force Between Two Point Charges (CIE A Level Physics)

Revision Note

Point charge approximation

Examiner Tip

Coulomb's law

Electrostatic attraction between two charges

Repulsive & attractive forces

Worked example

Examiner Tip

You've read 0 of your 10 free revision notes

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

Author: Ann H