Energy in SHM (Cambridge (CIE) A Level Physics): Revision Note
Exam code: 9702
Kinetic & potential energies
Simple harmonic motion also involves an interchange between potential and kinetic energy
The swinging of a pendulum is an interplay between gravitational potential energy and kinetic energy
The horizontal oscillation of a mass on a spring is an interplay between elastic potential energy and kinetic energy
The vertical oscillation of a mass on a spring is an interplay between elastic potential energy, gravitational potential energy and kinetic energy
Energy of a horizontal mass-spring system
The system has the maximum amount of elastic potential energy when the spring is stretched or compressed to its maximum displacement from the equilibrium position (amplitude)
When the mass is released, it moves towards the equilibrium position, accelerating as it goes, causing the kinetic energy to increase
At the equilibrium position, the velocity of the mass is at its maximum, therefore the kinetic energy is at its maximum and elastic potential energy is at its minimum
Once past the equilibrium position the kinetic energy decreases and elastic potential energy increases
Energy interchange for horizontal mass-spring system
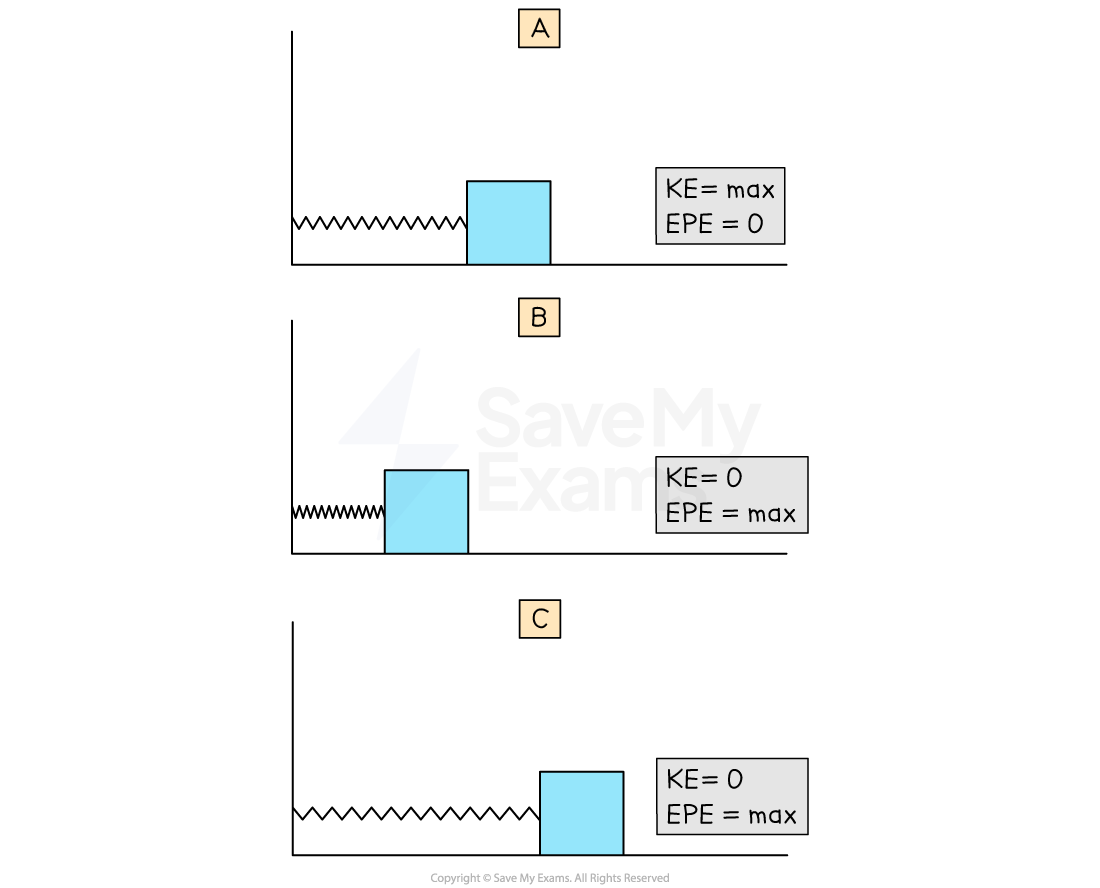
In a horizontal mass-spring system the kinetic energy is maximum in the equilibrium position and the elastic potential energy is maximum in the amplitude position
Energy of a simple pendulum
The pendulum has its maximum gravitational potential energy at the point of maximum displacement from the equilibrium position (amplitude)
When the pendulum is released, it moves towards the equilibrium position, accelerating as it goes and the kinetic energy increases
As the pendulum swings through the equilibrium position, its velocity is at a maximum, and therefore kinetic energy is at a maximum and gravitational potential energy is at a minimum
Once the mass has passed the equilibrium position, kinetic energy decreases and gravitational potential energy increases
Energy interchange for a simple pendulum
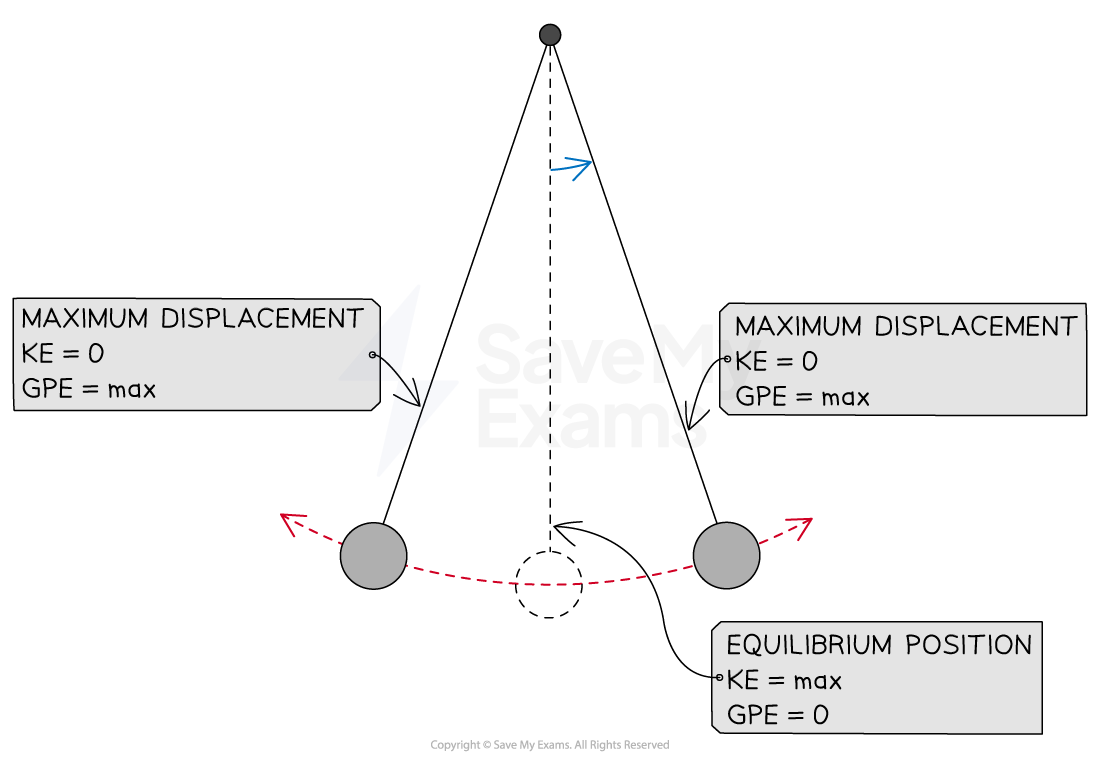
In a simple pendulum, the kinetic energy is maximum in the equilibrium position and the gravitational potential energy is maximum in the amplitude position
Examiner Tips and Tricks
You may be expected to draw as well as interpret energy graphs against time or displacement in exam questions. Make sure the sketches of the curves are as even as possible and use a ruler to draw straight lines, for example, to represent the total energy.
Calculating total energy of a simple harmonic system
Total energy equation
The total energy of a system undergoing simple harmonic motion is defined by:
Where:
E = total energy of a simple harmonic system (J)
m = mass of the oscillator (kg)
⍵ = angular frequency (rad s-1)
x0 = amplitude (m)
Sum of potential and kinetic energies
The total energy in the system remains constant, but the amount of kinetic and potential energy varies throughout the oscillation
Total energy is the sum of the potential and kinetic energies:
E = EP + EK
Where:
E = total energy in joules (J)
EP = potential energy in joules (J)
EK = kinetic energy in joules (J)
Remember the equations for potential and kinetic energy:
Gravitational potential energy: Ep = mgh
Elastic potential energy, Ep =
Kinetic energy, Ek =
Energy-displacement graph
The kinetic and potential energy transfers go through two complete cycles during one period of oscillation
One complete oscillation reaches the maximum displacement twice (once on the positive side and once on the negative side of the equilibrium position)
You need to be familiar with the graph showing the total, potential and kinetic energy transfers in half an SHM oscillation (half a cycle)
Energy displacement graph for half an oscillation
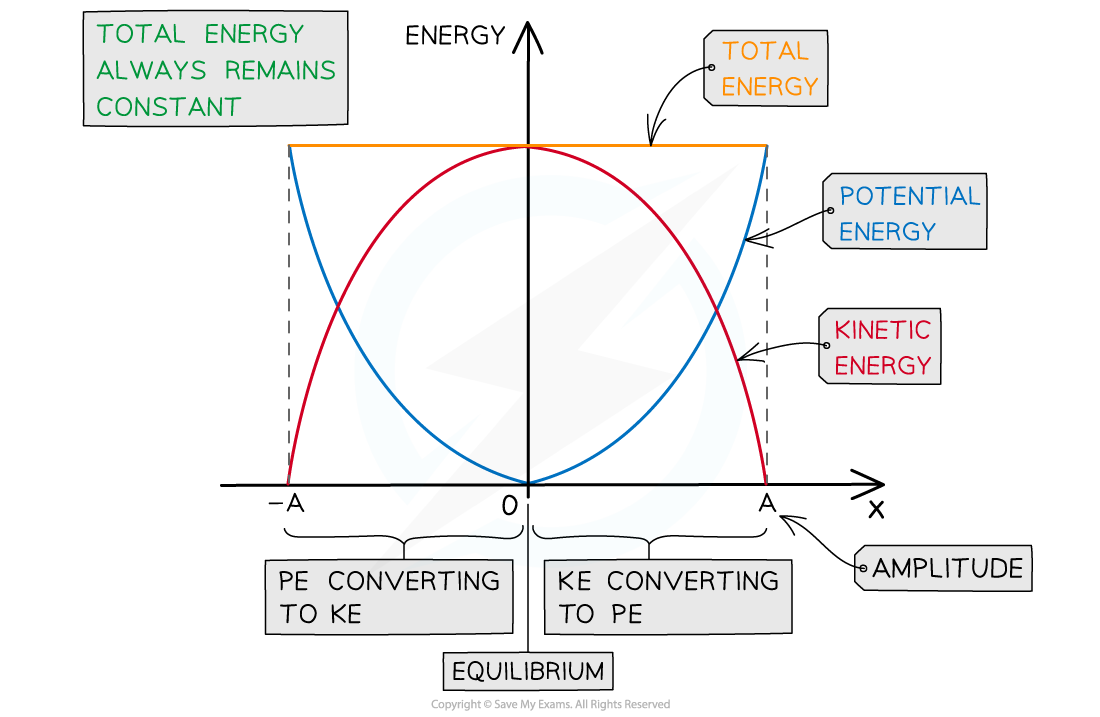
Graph showing the potential and kinetic energy against displacement in half a period of an SHM oscillation
The key features of the energy-displacement graph are:
Displacement occurs in both directions, so the graph has both positive and negative x values
The potential energy is always maximum at the amplitude positions x = A
The potential energy is always at a minimum or zero at the equilibrium position x = 0
This is represented by a ‘U’ shaped curve
The kinetic energy is the opposite:
Kinetic energy is at a minimum or zero at the amplitude positions x = A
Kinetic energy is at a maximum at the equilibrium position x = 0
This is represented by an ‘n’ shaped curve
The total energy is represented by a horizontal straight line above the curves
Worked Example
A ball of mass 23 g is held between two fixed points A and B by two stretch helical springs, as shown in the figure below.
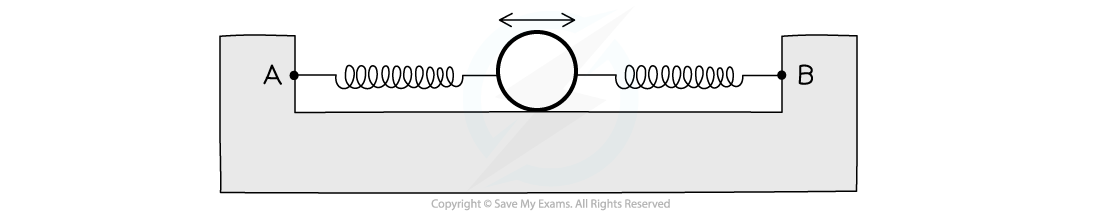
The ball oscillates along the line AB with simple harmonic motion of frequency 4.8 Hz and amplitude 1.5 cm.
Calculate the total energy of the oscillations.
Answer:
Step 1: Write down all known quantities
Mass, m = 23 g = 23 × 10–3 kg
Amplitude, x0 = 1.5 cm = 0.015 m
Frequency, f = 4.8 Hz
Step 2: Write down the equation for the total energy of SHM oscillations:
Step 3: Write an expression for the angular frequency
Step 4: Substitute values into the energy equation

You've read 0 of your 5 free revision notes this week
Unlock more, it's free!
Did this page help you?