Radians & Angular Displacement

The angle subtended at the centre of a circle by an arc equal in length to the radius of the circle

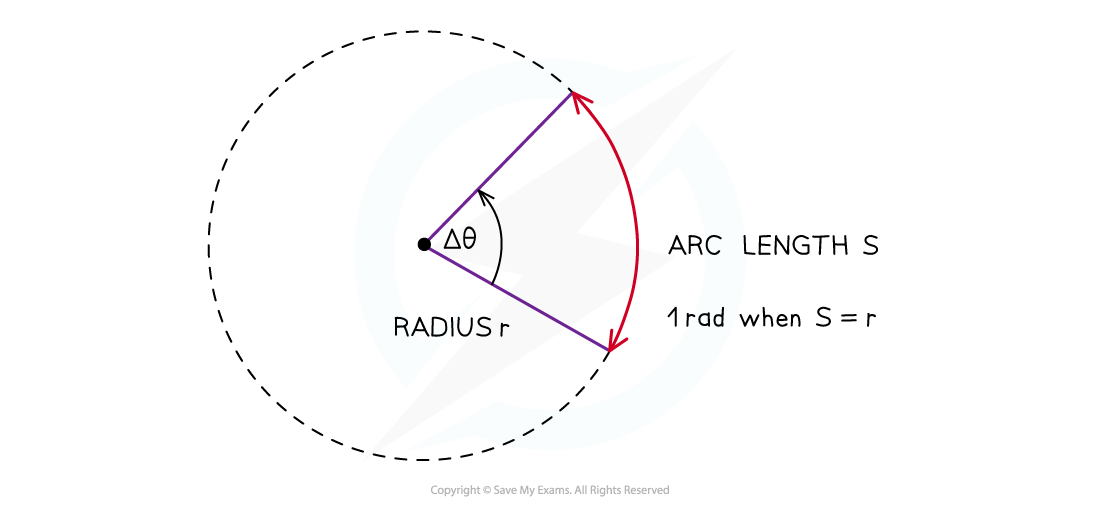
When the angle is equal to one radian, the length of the arc (S) is equal to the radius (r) of the circle

$\frac{circumference of circle}{radius} = \frac{2 π r}{r} = 2 π$

$θ ° \times (\frac{π}{180}) = θ rad$

Table of common degrees to radians conversions

In circular motion, it is more convenient to measure angular displacement in units of radians rather than units of degrees
Angular displacement is defined as:

The change in angle, in radians, of a body as it rotates around a circle

$∆ θ = \frac{distance travelled around the circle}{radius of the circle}$

Where:
- Δθ = angular displacement, or angle of rotation (radians)
- S = length of the arc, or the distance travelled around the circle (m)
- r = radius of the circle (m)

6-1-1-angle-in-radians_sl-physics-rn

An angle in radians, subtended at the centre of a circle, is the arc length divided by the radius of the circle

Convert the following angular displacement into degrees: WE - Radians conversion question image, downloadable AS & A Level Physics revision notes

Answer:

Step 1: Rearrange the degrees to radians conversion equation

$degrees \to radians \Rightarrow θ ° \times \frac{π}{180} = θ rad$

$radians \to degrees \Rightarrow θ rad \times \frac{180}{π} = θ °$

Step 2: Substitute the values to calculate

$\frac{π}{3} rad \times \frac{180}{π} = \frac{180 °}{3} = 60 °$

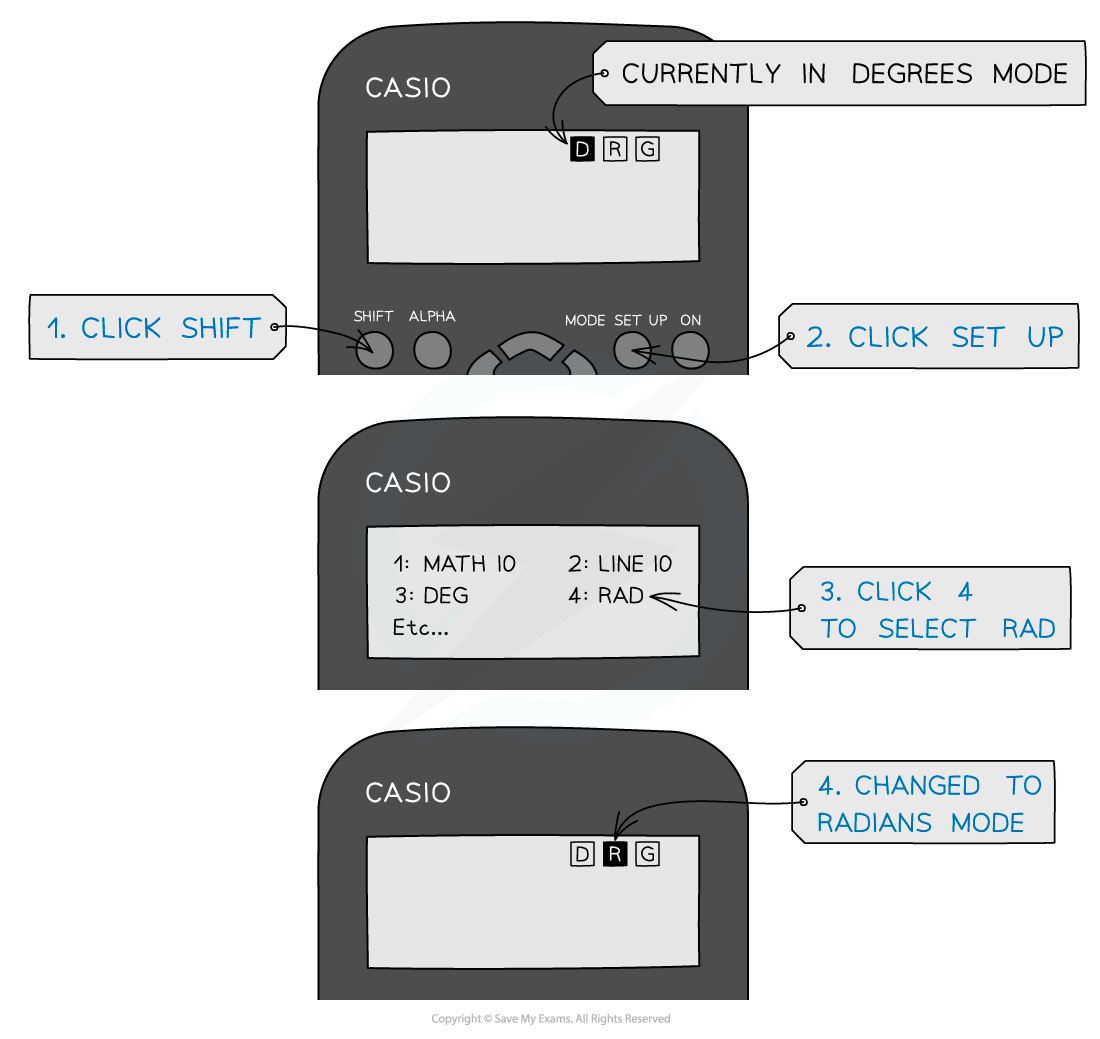
You will notice your calculator has a degree (Deg) and radians (Rad) mode
This is shown by the “D” or “R” highlighted at the top of the screen
Remember to make sure it’s in the right mode when using trigonometric functions (sin, cos, tan) depending on whether the answer is required in degrees or radians
It is extremely common for students to get the wrong answer (and lose marks) because their calculator is in the wrong mode - make sure this doesn’t happen to you!

Radians & Angular Displacement (CIE A Level Physics)