Scalars & Vectors (CIE A Level Physics)

• All quantities can be one of two types:
  • a scalar
  • a vector

Scalars

• Scalars are quantities that have magnitude but not direction
  • For example, mass is a scalar quantity because it has magnitude but no direction 

Vectors

• Vectors are quantities that have both magnitude and direction
  • For example, weight is a vector quantity because it is a force and has both magnitude and direction

Distance and displacement

• Distance is a measure of how far an object has travelled, regardless of direction
  
  • Distance is the total length of the path taken
  • Distance, therefore, has a magnitude but no direction
  • So, distance is a scalar quantity

• Displacement is a measure of how far it is between two points in space, including the direction
  
  • Displacement is the length and direction of a straight line drawn from the starting point to the finishing point
  • Displacement, therefore, has a magnitude and a direction
  • So, displacement is a vector quantity

What is the difference between distance and displacement?

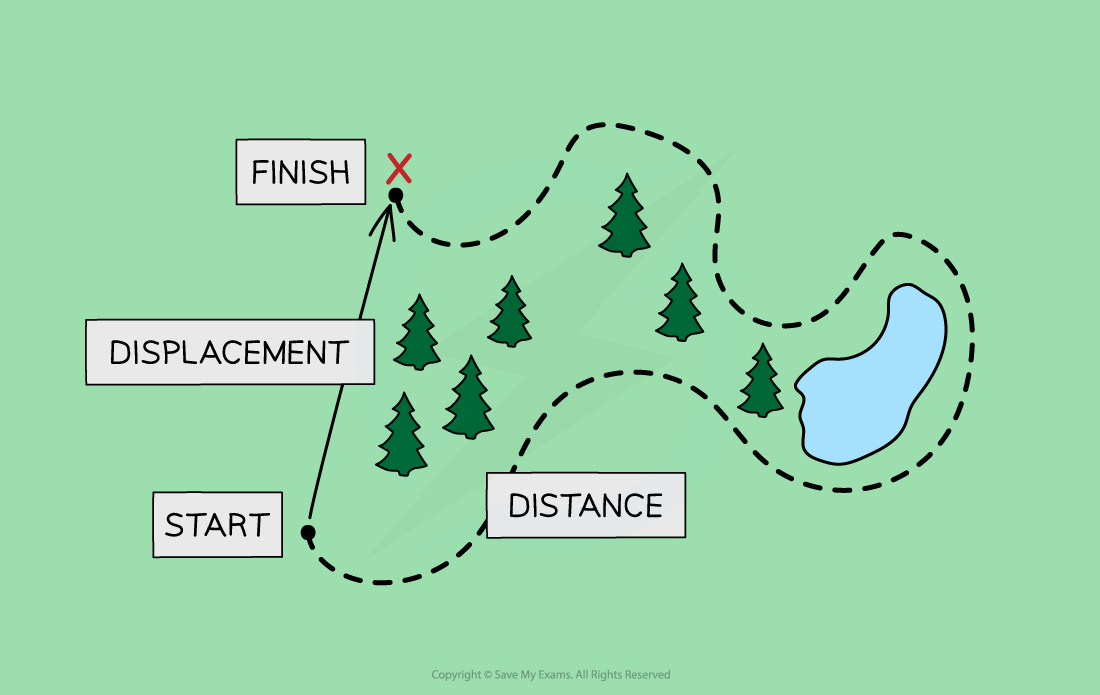
Displacement is a vector quantity while distance is a scalar quantity

• When a student travels to school, there will probably be a difference in the distance they travel and their displacement
  • The overall distance they travel includes the total lengths of all the roads, including any twists and turns
  • The overall displacement of the student would be a straight line between their home and school, regardless of any obstacles, such as buildings, lakes or motorways, along the way

Speed and velocity

• Speed is a measure of the distance travelled by an object per unit time, regardless of the direction
  • The speed of an object describes how fast it is moving, but not the direction it is travelling in
  • Speed, therefore, has magnitude but no direction
  • So, speed is a scalar quantity

• Velocity is a measure of the displacement of an object per unit time, including the direction
  • The velocity of an object describes how fast it is moving and which direction it is travelling in
  • An object can have a constant speed but a changing velocity if the object is changing direction
  • Velocity, therefore, has magnitude and direction
  • So, velocity is a vector quantity

Examples of scalars & vectors

• The table below lists some common examples of scalar and vector quantities

Table of scalars and vectors

• Vectors are represented by an arrow
  • The arrowhead indicates the direction of the vector
  • The length of the arrow represents the magnitude

• Vectors can be combined by adding or subtracting them from each other
• There are two methods that can be used to combine vectors: the triangle method and the parallelogram method

Triangle Method

• To combine vectors using the triangle method:
  • Step 1: link the vectors head-to-tail
  • Step 2: the resultant vector is formed by connecting the tail of the first vector to the head of the second vector
  • To subtract vectors, change the direction of the vector from positive to negative and add them in the same way

Triangle method for adding and subtracting vectors

The triangle method links vectors tip to tail to find the resultant vector

Parallelogram method

• To combine vectors using the parallelogram method:
  • Step 1: link the vectors tail-to-tail
  • Step 2: complete the resulting parallelogram
  • Step 3: the resultant vector is the diagonal of the parallelogram

Parallelogram method for adding and subtracting vectors

The parallelogram method links vectors tail to tail to find the resultant vector 

• When two or more vectors are added together (or one is subtracted from the other), a single vector is formed, known as the resultant vector
• The magnitude of the resultant vector can be found using Pythagoras' theorem or trigonometry

Condition for equilibrium

• Coplanar forces can be represented by vector triangles
• In equilibrium, these are closed vector triangles.
  • The vectors, when joined together, form a closed path

Forces in equilibrium

If three forces acting on an object are in equilibrium; they form a closed triangle

• Two vectors can be represented by a single resultant vector that has the same effect
• A single resultant vector can be resolved and represented by two vectors, which in combination have the same effect as the original one
• When a single resultant vector is broken down into its parts, those parts are called components
• For example, a force vector of magnitude F and an angle of θ to the horizontal is shown below

Resultant vector diagram

A resultant vector, F

• It is possible to resolve this vector into its horizontal and vertical components using trigonometry

Horizontal and vertical vector components

Horizontal and vertical components of F

• For the horizontal component, F_x = Fcosθ
• For the vertical component, F_y = Fsinθ