Calculating Uncertainties (Cambridge (CIE) A Level Physics)

Calculating uncertainty

What is uncertainty?

• There is always a degree of uncertainty when measurements are taken; the uncertainty can be thought of as the difference between the actual reading taken (caused by the equipment or techniques used) and the true value

• Uncertainties are not the same as errors

  • Errors can be thought of as issues with equipment or methodology that cause a reading to be different from the true value

  • The uncertainty is a range of values around a measurement within which the true value is expected to lie, and is an estimate

• For example, if the length of a box is measured multiple times as 12.55 cm, 12.45 cm and 12.51 cm, we can say the length is 12.50 cm with an uncertainty of 0.05 cm,

  • This is often written as 12.50 ± 0.05 cm

Calculating Uncertainty

• Uncertainties can be represented in a number of ways:

  • Absolute Uncertainty: where uncertainty is given as a fixed quantity (as above)

  • Fractional Uncertainty: where uncertainty is given as a fraction of the measurement

  • Percentage Uncertainty: where uncertainty is given as a percentage of the measurement

• To find uncertainties in different situations:

  • The uncertainty in a reading (e.g. from a voltmeter): ± half the smallest division

  • The uncertainty in a measurement (e.g. from a ruler): at least ±1 smallest division

  • The uncertainty in repeated data: half the range i.e. ± ½ (largest - smallest value)

  • The uncertainty in digital readings: ± the last significant digit unless otherwise quoted

Diagram showing a reading from an ammeter

Combining uncertainties

• When combining two measurements that both have uncertainties, the uncertainties have to be combined too

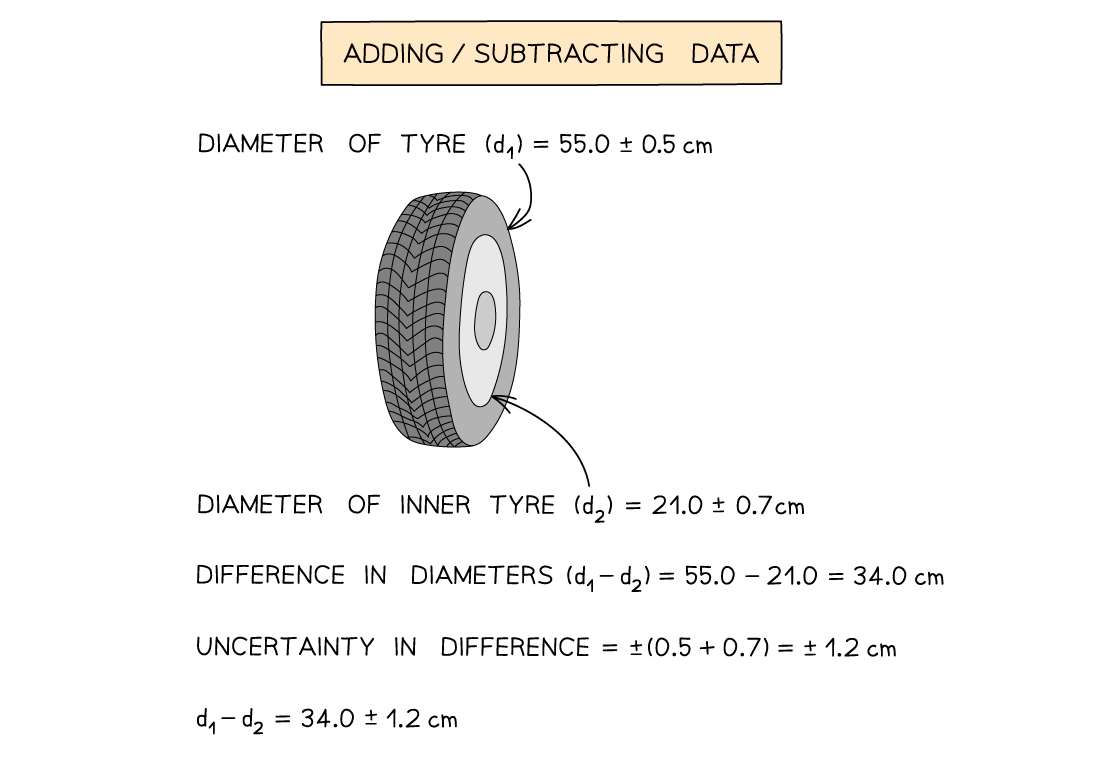
Adding or subtracting data

• When adding or subtracting two values with uncertainties, just add the absolute uncertainties

Adding or subtracting data example

Multiplying or dividing data

• When multiplying or dividing measurements with uncertainties, add their percentage uncertainties

Multiplying or dividing data example

• When the measurement is raised to a power, multiply the fractional or percentage uncertainty by the power

Raising to a power example