Deformation: Stress & Strain (CIE A Level Physics)

[1]

[1]

A block of mass 0.40 kg slides in a straight line with a constant speed of 0.30 m s^–1 along a horizontal surface, as shown in Fig. 3.1.

Fig. 3.1

Assume that there are no resistive forces opposing the motion of the block.

The block hits a spring and decelerates. The speed of the block becomes zero when the compression of the spring is 8.0 cm.

kinetic energy = ....................................................... J [2]

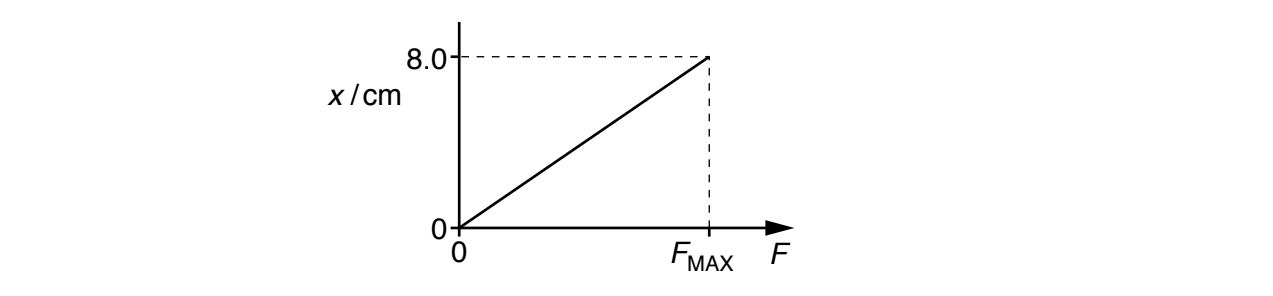
Fig. 3.2

Assume that the elastic potential energy of the spring when its compression is 8.0 cm is equal to the initial kinetic energy of the block.

Use your answer in (b)(i) to calculate the maximum force F_max exerted on the spring by the block.

F_max = ...................................................... N [2]

deceleration = ................................................. m s^–2 [2]

•   before it hits the spring

...........................................................................................................................................

•   when its speed becomes zero.

...........................................................................................................................................

[2]

A short time later, the block has a speed of 0.15 m s^–1 as it loses contact with the spring and moves back along its original path.

Calculate the magnitude of the change in momentum of the block.

change in momentum = .................................................... N s [2]

Define work done by a force.

A heavy suitcase is placed on a weighing machine consisting of a platform mounted on a spring as shown in Fig. 1.1.

Describe and explain how work is done in this situation.

The diagram in Fig.1.2 shows a suitcase of mass 22.6 kg positioned on the weighing platform.

The platform sinks down 3 cm when the suitcase is placed on it, and then returns to its original position when the suitcase is removed.

Calculate the spring constant of the spring.

For the weighing platform described in part (c) sketch a graph to show the known properties of the spring.

Fig. 1.1 is a graph of stress against strain for two wires, X and Y. The wires are made from different materials but have the same dimensions.

State and explain which wire would be more suitable for use as cables and structural beams.

A group of physics students are told that they will be performing the investigation which leads to the graph in part (a).  

State two safety precautions which they must take and explain your answer.

Fitness equipment often uses springs as a method of creating resistance. One example is the Push Down Bar, which the manufacturer says can help a person who uses it to 'increase strength and burn calories'.

To use a Push Down Bar a person applies force by pushing the handles towards each other as shown in Fig. 1.1.

Fig. 1.2 shows the construction of the Push Down Bar.

The relationship between applied force and change in length of the springs was measured for a range of values of force.

The results are plotted on the graph in Fig. 1.3.

Derive the formula for the elastic potential energy stored by the spring from the graph of force against extension.

State and explain whether the material chosen for the spring for the equipment in Fig. 1.1 should exhibit elastic or plastic behaviour.

Nichrome is a common alloy used to make coins and heating elements in electrical appliances. It consists of 80% by volume of nickel and 20% by volume of chromium.

Determine the mass of nickel and the mass of chromium required to make a wire of nichrome of volume 0.50 × 10⁻³ m³. Density of nickel = 8.9 × 10³ kgm³

Density of chromium = 7.1 × 10³ kgm³

To calculate the breaking stress of the nichrome wire both the cross-sectional area of the wire and the applied load must be known.

For each value, briefly describe an experimental method to determine the value, mentioning any equipment required.

The radius of the nichrome wire is 5.7 mm. The wire breaks under an applied force of 72 kN.

Calculate the breaking stress of nichrome.

The breaking stresses of nickel is 59 MPa. The breaking stress of chromium is 131 MPa.

Use your answer to part (c) to suggest why nichrome is a suitable material for making coins.