Attenuation of Ultrasound in Matter (Cambridge (CIE) A Level Physics): Revision Note
Exam code: 9702
Attenuation of ultrasound in matter
Attenuation of ultrasound is defined as:
The reduction of energy due to the absorption of ultrasound as it travels through a material
The attenuation coefficient of the ultrasound is expressed in decibels per centimetre lost for every incremental increase in megahertz frequency
Generally, 0.5 dB cm–1 is lost for every 1 MHz
The intensity I of the ultrasound decreases with distance x, according to the equation:
Where:
I0 = the intensity of the incident beam (W m-2)
I = the intensity of the reflected beam (W m-2)
μ = the absorption coefficient (m-1)
x = distance travelled through the material (m)
The absorption coefficient μ, will vary from material to material
Attenuation is not a major problem in ultrasound scanning as the scan relies on the reflection of the ultrasounds at the boundaries of materials
Intensity-depth graph showing attenuation
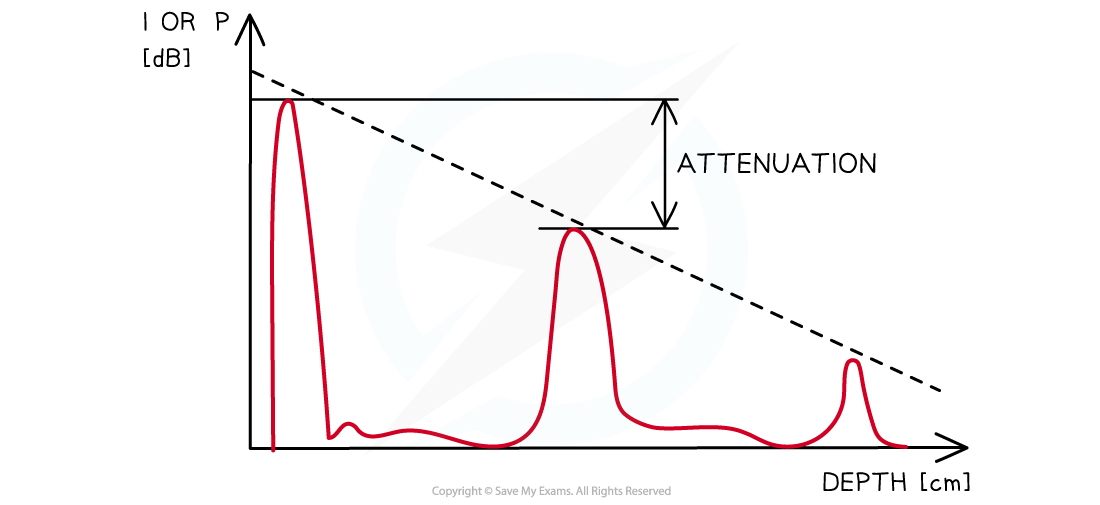
When the intensity is expressed in decibels, the amplitudes of the echoes can be seen to decrease linearly
Worked Example
The thickness x of the layer of fat on an animal, as shown in the diagram, is to be investigated using ultrasound.
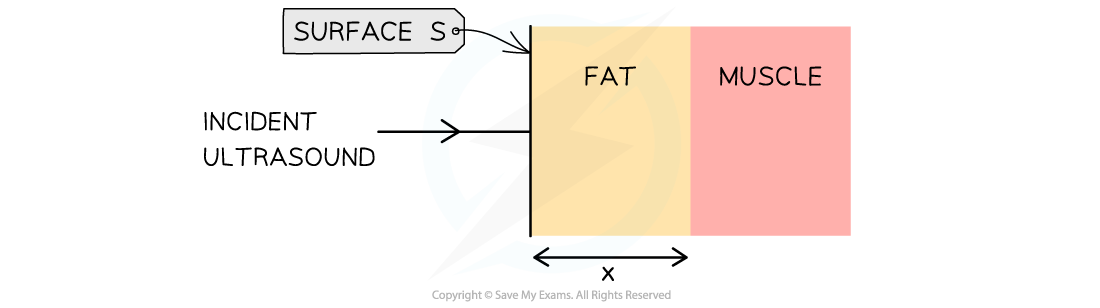
The intensity of the parallel ultrasound beam entering the surface S of the layer of fat is .
The beam is reflected from the boundary between fat and muscle.
The intensity of the reflected ultrasound detected at the surface S of the fat is .
Medium | Z / kg m–2 s–1 | μ / m–1 |
Fat | 1.3 × 106 | 48 |
Muscle | 1.7 × 106 | 23 |
Using the information in the table, calculate:
(a) The intensity reflection coefficient at the boundary between the fat and the muscle.
(b) The thickness of the layer of fat.
Answer:
Part (a)
Step 1: Write down the equation for intensity reflection coefficient α
Step 2: Calculate the intensity reflection coefficient
This means that 0.018 of the intensity is reflected at the interface between fat and muscle
This reflected intensity will move back through the fat towards surface S
Part (b)
Step 1: Write out the known quantities
The intensity of the ultrasound pulse is affected 3 times:
Attenuation from the surface S to the fat-muscle boundary
Reflection at the boundary
Attenuation from the boundary back to the surface S
After being transmitted in the fat, the intensity at surface S is given to be
.
Therefore, the intensity is
at the fat-muscle boundary, and as the ultrasound moves through the fat, it gets attenuated and the new intensity at the surface S is now
incident intensity = intensity of the reflected pulse
Transmitted intensity =
Absorption coefficient,
= 48 m-1
Thickness of fat =
Step 2: Write out the equation for attenuation
Step 3: Substitute in values for intensity and simplify
Step 4: Rearrange and take the natural log of both sides
Step 5: Rearrange and calculate the thickness x
Examiner Tips and Tricks
The intensity equation will not be provided for you on your exam datasheet, so make sure you remember this!

Unlock more, it's free!
Did this page help you?