Figure 1 shows a current-carrying conductor at an angle θ to an external magnetic field.
Figure 1
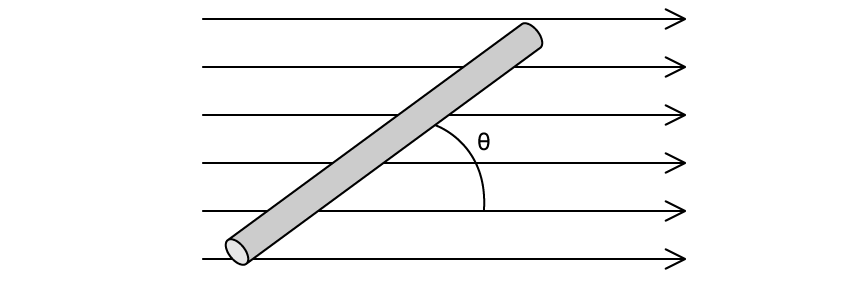
The equation for the force acting on the current-carrying conductor at different angles, θ, to the magnetic field is given by
State the meaning of the symbols B, I and L.
State the angle, θ, between the conductor and the magnetic field which would result in the largest force being exerted on the conductor.
State the angle, θ, between the conductor and the magnetic field which would result in no force being exerted on the conductor.
The conductor in Figure 1 has a length of 1.2 m and has a current of 0.85 A flowing through it. The conductor is placed at 30o to the B field, which has a magnetic flux density of 70 mT.
Calculate the force acting on the conductor.
Did this page help you?



















