State the definition for electric potential.
State whether electric potential is a scalar or vector quantity.
Give a reason for your answer.
State what happens to the magnitude of the electric potential the further a positive test charge is moved from:
(i) A positive charge
(ii) A negative charge
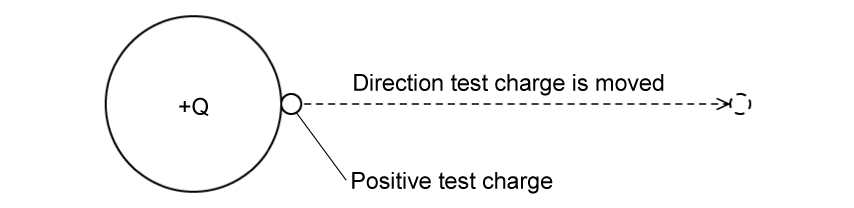
A positive test charge, +q, is moved away from the surface of an object with charge +Q, as shown in Figure 1.
Figure 1
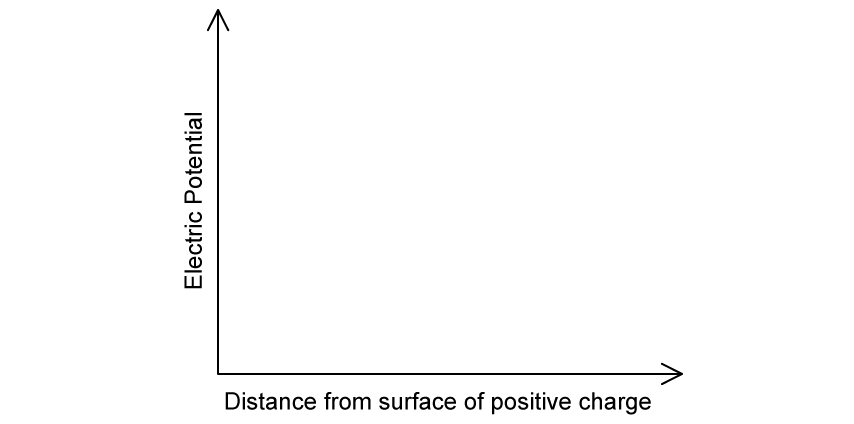
On Figure 2 below, sketch a graph to show how the electric potential varies as the test charge is moved from the surface of the positive charge.
Figure 2
Did this page help you?
















