Define the density of a material.
A student takes an empty measuring cylinder and places it on an electronic balance. She records the mass and then adds an unknown liquid to the measuring cylinder. She records the volume of liquid in the measuring cylinder and the reading on the electronic balance. She repeats this process and plots her results on a graph as shown in Figure 1 below.
Figure 1
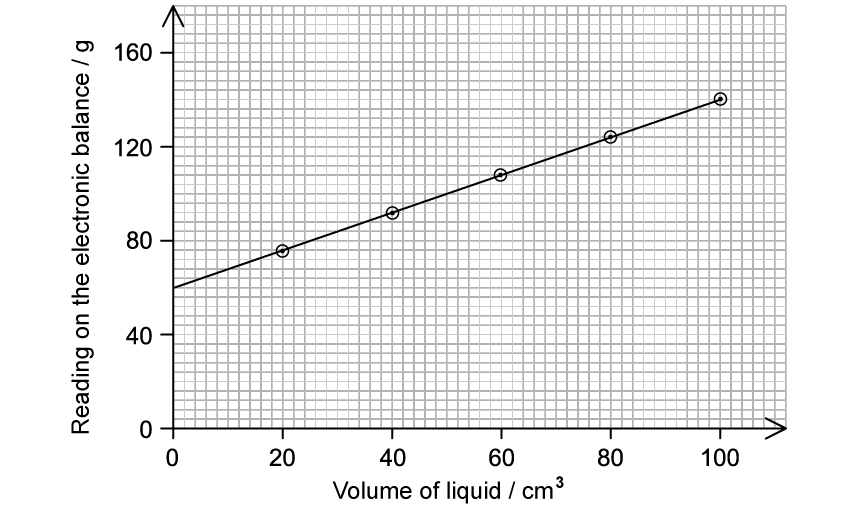
Determine the mass of the empty measuring cylinder.
Table 1 shows four different liquids and their densities.
Table 1
Liquid | Density /kg m–3 |
Ethanol | 800 |
Water | 1000 |
Petrol | 700 |
Castor oil | 900 |
Using the data from the graph and your answer to part (b) identify the liquid which the student used. You must show full working out.
You may use the conversion: 1 m3 = 1 × 106 cm3
The student repeats the experiment with a liquid which has a lower density than the one plotted in Figure 1.The same measuring cylinder was used for both experiments.
Draw a line on Figure 1 to show the results she would expect to obtain from this second liquid.
Did this page help you?




















