Nuclear Radius Equation (AQA A Level Physics) : Revision Note
Nuclear Radius Values
The radius of some nuclei are shown in the table below:
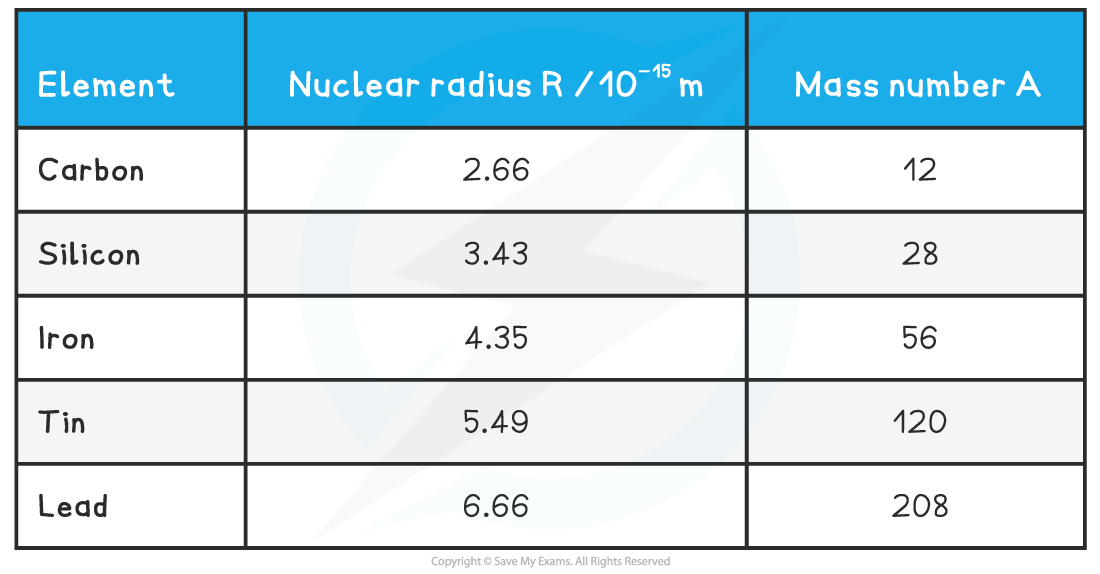
In general, nuclear radii are of the order 10–15 m or 1 fm
The nuclear radius, R, varies with nucleon number, A as follows:

The key features of this graph are:
The graph starts with a steep gradient at the origin
Then the gradient gradually decreases to almost horizontal
This means that
As more nucleons are added to a nucleus, the nucleus gets bigger
However, the number of nucleons A is not proportional to its size r
Radius v Nucleon Number
The radius of nuclei depends on the nucleon number, A of the atom
This makes sense because as more nucleons are added to a nucleus, more space is occupied by the nucleus, hence giving it a larger radius
The exact relationship between the radius and nucleon number can be determined from experimental data
By doing this, physicists were able to deduce the following relationship:
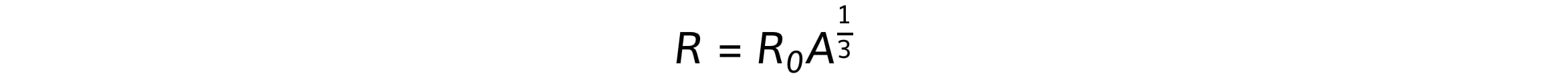
Where:
R = nuclear radius (m)
A = nucleon / mass number
R0 = constant of proportionality = 1.05 fm
Plotting a graph of R against A1 / 3 gives a straight line through the origin with the gradient equal to R0

It is also possible to plot a logarithmic graph of the relationship which can be derived as follows:
ln R = ln (R0 A1 / 3)
ln R = ln R0 + ln (A1 / 3)
ln R = ln R0 + 1/3 ln A
Therefore, a graph of ln R against ln A yields a straight line
Comparing this to the straight-line equation: y = mx + c
y = lnR
x = lnA
m (the gradient) = 1/3
c (y-intercept) = ln R0

Worked Example
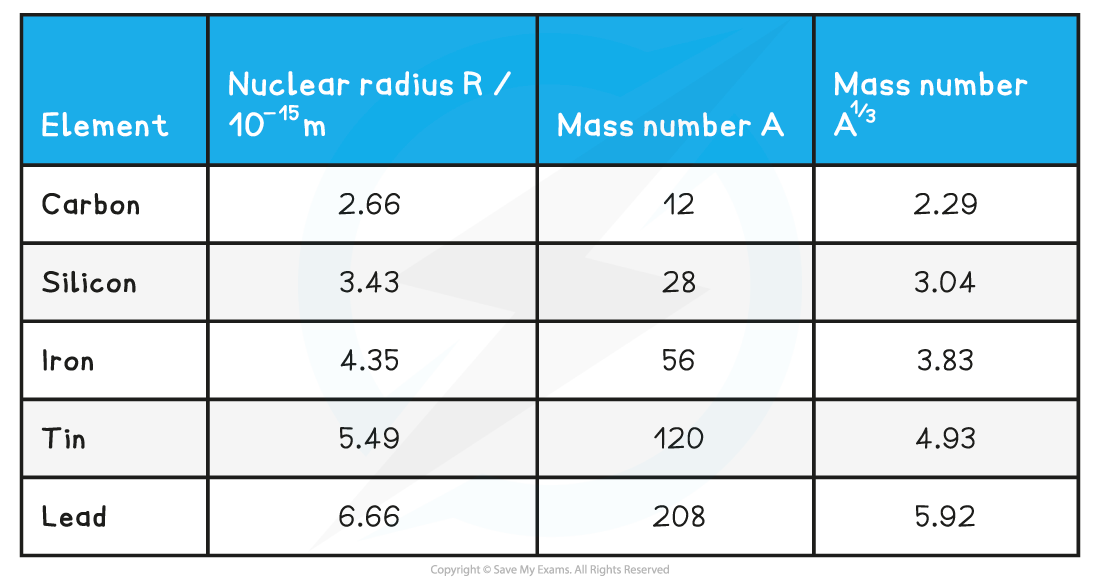
Verify the experimental relationship between R and A using the data from the table above and estimate a value of R0.
Answer:
Step 1: Add a column to the table to determine the values for A1 / 3
Step 2: Plot a graph of R against A1 / 3 and draw a line of best fit

Step 3: Calculate the gradient
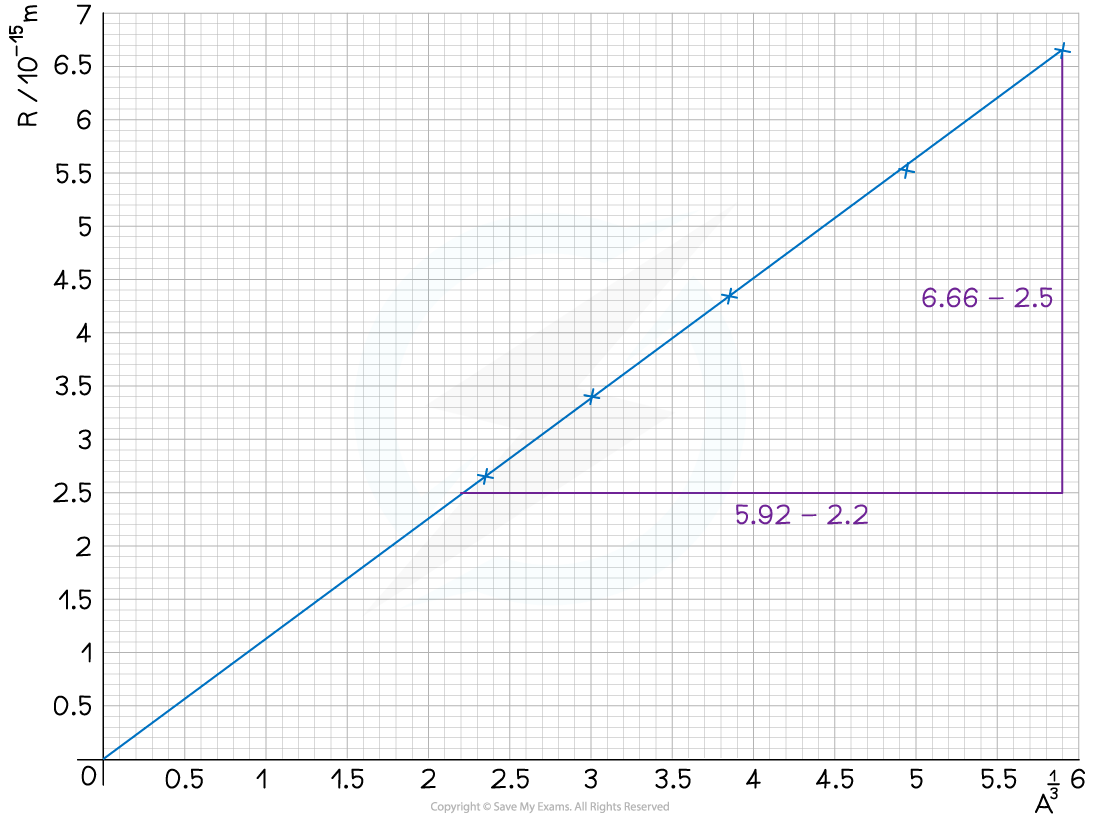
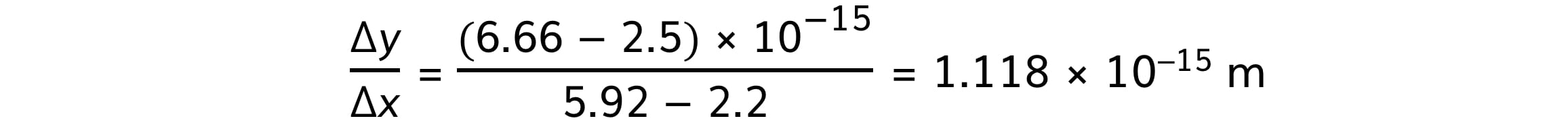
R0 = 1.12 fm

You've read 0 of your 5 free revision notes this week
Sign up now. It’s free!
Did this page help you?
