A LevelPhysicsAQARevision NotesFields & Their ConsequencesOrbits of Planets & SatellitesGeostationary Orbits

Geostationary Orbits (AQA A Level Physics): Revision Note

Exam code: 7408

Written by: Katie M

Updated on 2 July 2026

Synchronous Orbits

A synchronous orbit is:

An orbit in which the orbital period of the satellite is equal to the rotational period (or length of day) of the planet or body it is orbiting

The following characteristics are implied:
- Equal Period
  - The time taken for the satellite to complete one full orbit is exactly the same as the time taken for the planet to rotate once on its axis
- Same Angular Speed
  - The satellite has the same angular speed (or angular velocity) as the planet it is orbiting
Synchronous orbits usually refer to satellites (the orbiting body) around planets (the body being orbited)
A synchronous orbit can be in any plane and at any longitude
- It does not have to be above the equator or above a fixed point on the surface

Geosynchronous Orbits

A geosynchronous orbit is an Earth-centred synchronous orbit
- The orbital period is 24 hours (matching Earth's rotation)
- They may have inclined orbits
- They may have slightly elliptical orbits
- The object in orbit does not stay above one fixed point on the Earth's surface

Geostationary Orbits

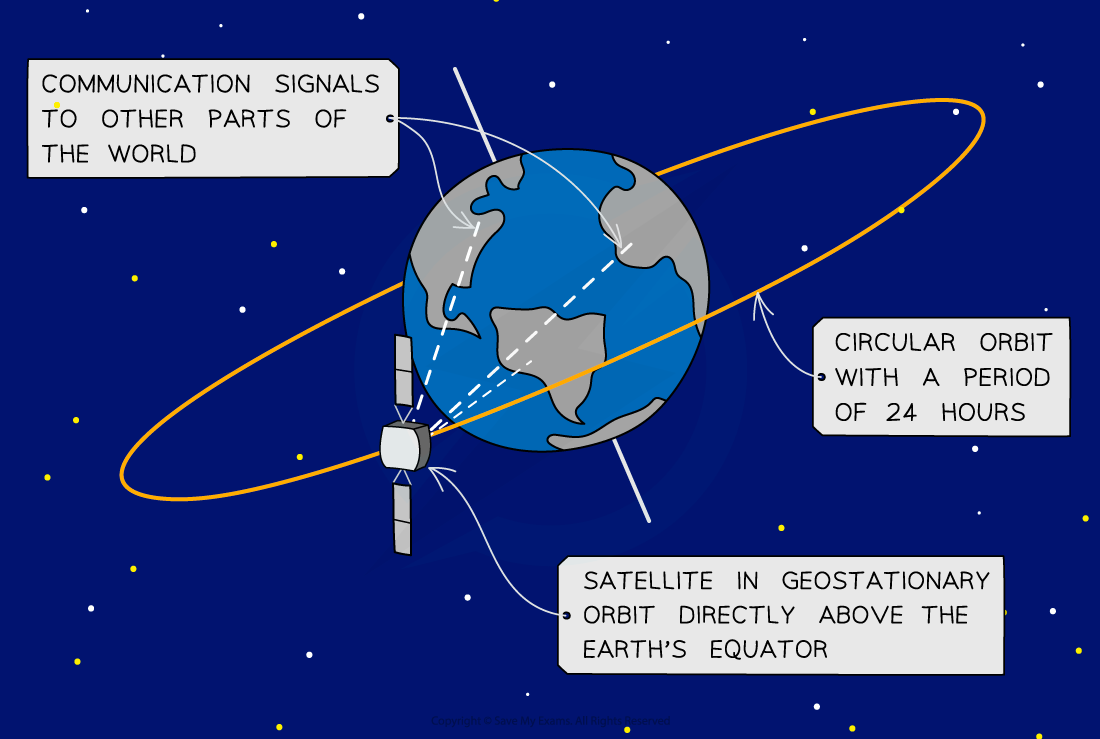
Geostationary Orbit

A geostationary orbit is a specific type of geosynchronous orbit around the Earth
A geostationary orbit must meet the following criteria:
- The orbital period is 24 hours (matching Earth's rotation)
- The orbit must be in the plane of the equator
- The object in orbit does stay above one fixed point on the Earth's surface
Geostationary satellites are used for telecommunication transmissions (e.g. radio) and television broadcasts
A base station on Earth sends the TV signal up to the satellite, where it is amplified and broadcast back to the ground to the desired locations
The satellite receiver dishes on the surface must point towards the same point in the sky
- Since the geostationary orbits of the satellites are fixed, the receiver dishes can be fixed too

Low Orbits

Some satellites are in low orbits, which means their altitude is closer to the Earth's surface
One example of this is a polar orbit, where the satellite orbits around the north and south poles of the Earth
Low orbits are useful for taking high-quality photographs of the Earth's surface. This could be used for:
- Weather
- Military applications

Geostationary orbit satellite, downloadable AS & A Level Physics revision notes

Geostationary satellite in orbit

Worked Example

The table gives data for two types of satellite, a low-Earth orbit (LEO) and a geostationary orbit

Orbit type	T / min	h / km
LEO	89	250
Geostationary	X	Y

For the geostationary orbit, calculate

(i) the orbital period X in minutes.

(ii) the height Y above the Earth's surface that a geostationary satellite will orbit in km.

Answer:

Part (i)

Step 1: Convert the time period from seconds to minutes

The period of a geostationary orbit is X = 24 hrs
- The period of a geostationary orbit is X = 24 × 60 = 1440 minutes

Part (ii)

Step 1: List the known quantities

Period of the LEO, T_L = 89 min
- Period of a geostationary orbit, T_G = 1440 min
- Height above Earth of the LEO, h_L = 250 km
- Radius of the Earth, R = 6.37 × 10⁶ m (from the data sheet)

Step 2: Recall the relationship between orbital period and radius

Orbital period T is related to the radius r of the orbit by $T^{2} \propto r^{3}$

Step 3: Convert the proportional relationship into an equation

$\frac{T_{G}^{2}}{T_{L}^{2}} = \frac{r_{G}^{3}}{r_{L}^{3}}$

$r_{G}^{3} = r_{L}^{3} {(\frac{T_{G}}{T_{L}})}^{2}$

$r_{G} = \sqrt[3]{r_{L}^{3} {(\frac{T_{G}}{T_{L}})}^{2}} = r_{L} {(\frac{T_{G}}{T_{L}})}^{\frac{2}{3}}$

Step 4: Evaluate a final value for Y

Orbital radius of LEO:

$r_{L} = R + h_{L} = (6.37 \times 10^{6}) + (250 \times 10^{3}) = 6.62 \times 10^{6} m$

Orbital radius of geostationary:

$r_{G} = (6.62 \times 10^{6}) {(\frac{1440}{89})}^{\frac{2}{3}} = 4.235 \times 10^{7} m$

Height above the Earth's surface:

Y = $(4.235 \times 10^{7}) - (6.37 \times 10^{6}) = 3.6 \times 10^{7} m$

Height above the Earth's surface: Y = 36 000 km

Examiner Tips and Tricks

Make sure to memorise the key features of a geostationary orbit, since this is a common exam question. Remember:

Equatorial orbit
Moves west to east
Period of 24 hours

You will also be expected to remember that the time period of the orbit is 24 hours for calculations on a geostationary satellite.

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

I would just like to say a massive thank you for putting together such a brilliant, easy to use website.I really think using this site helped me secure my top gradesin science and maths. You really did save my exams! Thank you.

Beth
IGCSE Student

This website is soooo useful and I can’t ever thank you enough for organising questions by topic like this. Furthermore, the name of the website could not have been more appropriate as it literally did SAVE MY EXAMS!

Fathima
A Level Student

Incredible! SO worth my money, the revision notes have everything I need to know and are so easy to understand. I actually enjoy revising! It makes me feel a lot more confident for my GCSEs in a few months.

Kate
GCSE Student

Absolutely brilliant, both my girls used it for A levels and GCSE. It's saves on paper copies, also beneficial exam questions ranked from easy to hard. It's removed a lot of stress from the exams.

Sameera
Parent

Just to say that your resources are the best I have seen and I have been teaching chemistry at different levels for about 40 years

Mark
Chemistry Teacher

Excellent