Period of Mass-Spring System (AQA A Level Physics) : Revision Note
Period of Mass-Spring System
A mass-spring system consists of a mass attached to the end of a spring
The equation for the restoring force (the force responsible for the SHM) is FH = - kx
This is the same as the equation for Hooke's Law
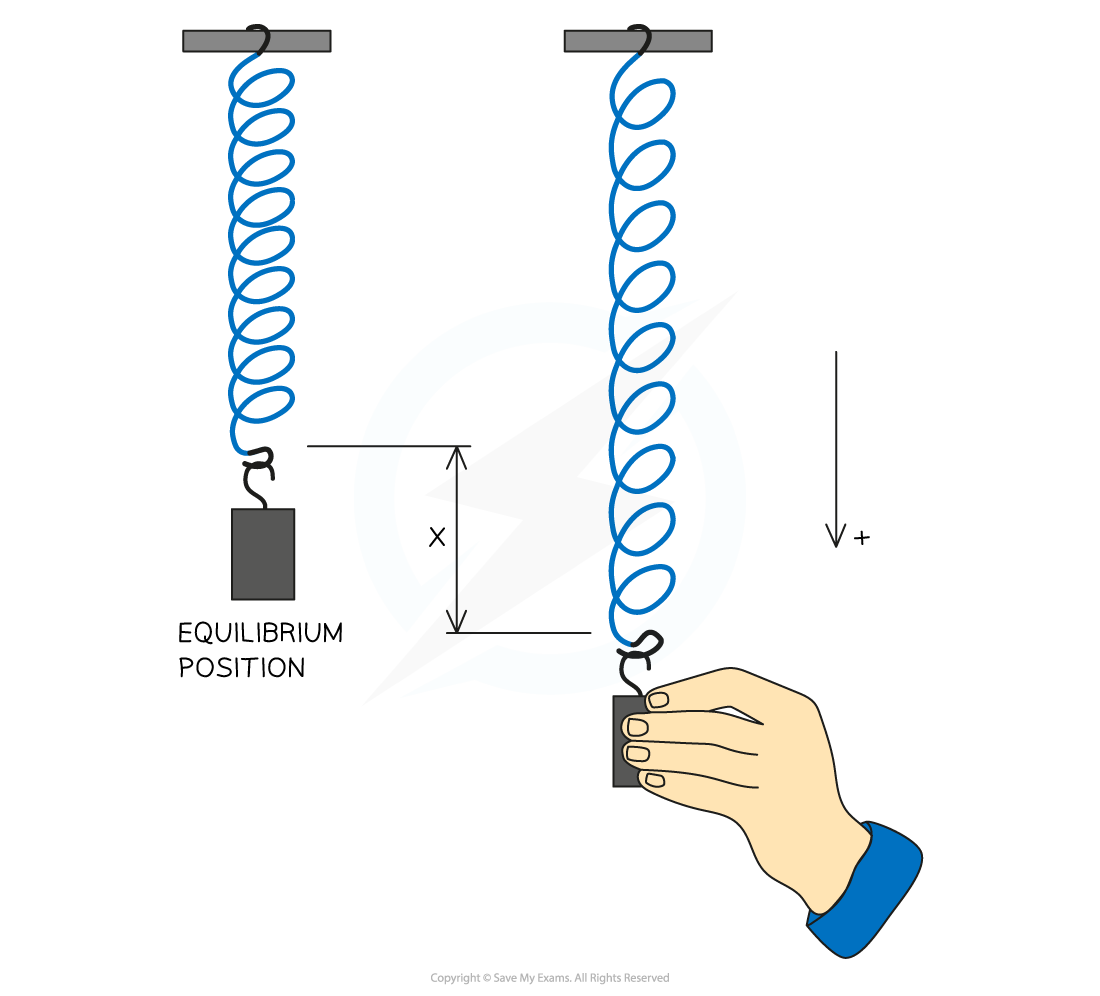
The time period of a mass-spring system is given by:
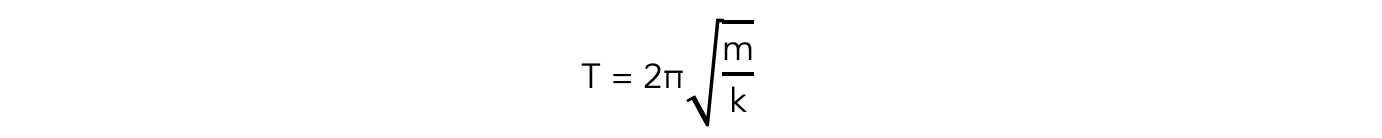
Where:
T = time period (s)
m = mass on the end of the spring (kg)
k = spring constant (N m-1)
This equation applies to both horizontal and vertical mass-spring systems:
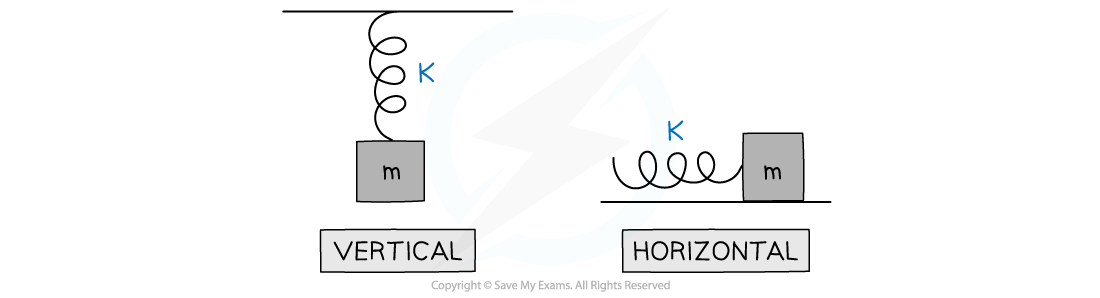
A mass-spring system can be either vertical or horizontal. The time period equation applies to both
The equation shows that the time period and frequency, of a mass-spring system, does not depend on the force of gravity
Therefore, the oscillations would have the same time period on Earth and the Moon
The higher the spring constant k, the stiffer the spring and the shorter the time period of the oscillation
Worked Example
Calculate the frequency of a mass of 2.0 kg attached to a spring of spring constant 0.9 N m–1 oscillating in simple harmonic motion.
Answer:
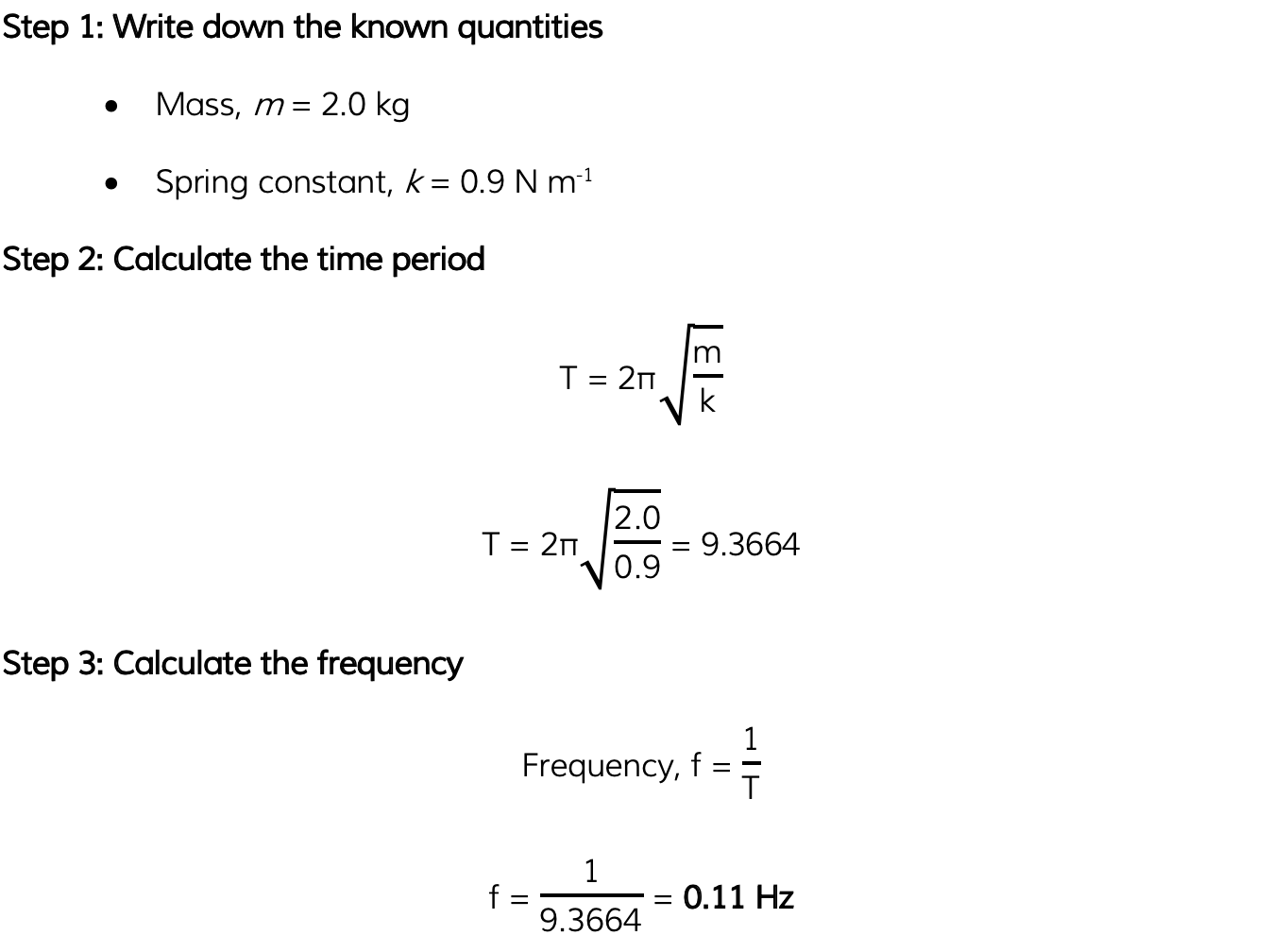
Examiner Tips and Tricks
Another area of physics where you may have seen the spring constant k is from Hooke's Law. Exam questions commonly merge these two topics together, so make sure you're familiar with the Hooke's Law equation too.

You've read 0 of your 5 free revision notes this week
Sign up now. It’s free!
Did this page help you?
