Period of Simple Pendulum (AQA A Level Physics) : Revision Note
Period of Simple Pendulum
A simple pendulum consists of a string and a bob at the end
The bob is a weight, generally spherical and considered a point mass
The bob moves from side to side
The string is light and inextensible remaining in tension throughout the oscillations
The string is attached to a fixed point above the equilibrium position
The time period of a simple pendulum for small angles of oscillation is given by:
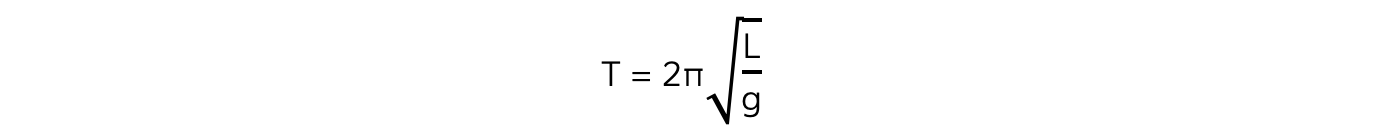
Where:
T = time period (s)
L = length of string (from the pivot to the centre of mass of the bob) (m)
g = gravitational field strength (N kg-1)
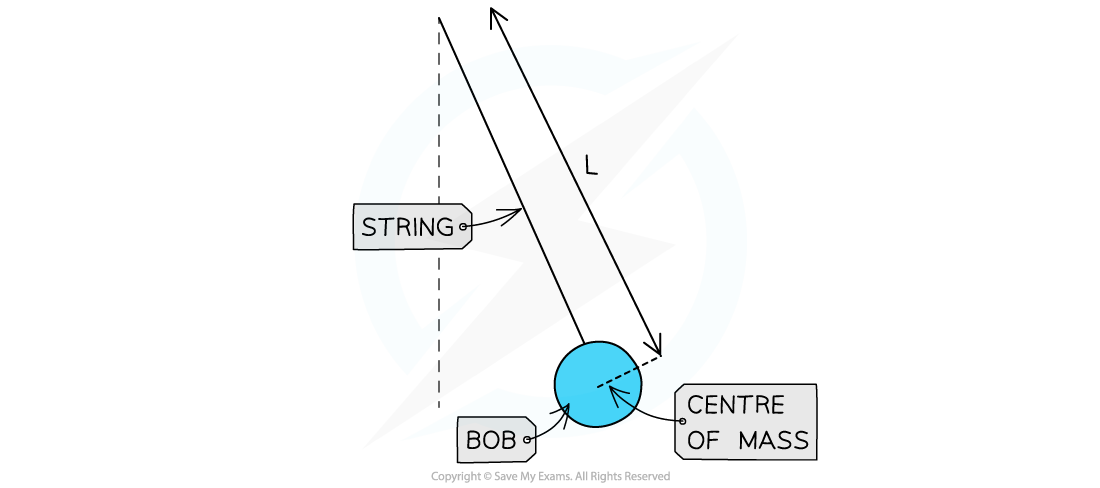
A simple pendulum
The time period of a pendulum does depend on the gravitational field strength, meaning its period would be different on the Earth and the Moon
Small Angle Approximation
This formula is limited to small angles (θ < 10°) and therefore small amplitudes of oscillation from the equilibrium point
The restoring force of the pendulum is the weight component acting along the arc of the circle towards the equilibrium position
It is resolved to act at an angle θ to the horizontal x
When considering SHM because of small angle approximation it is assumed the restoring force acts along the horizontal
So sin θ ≅ θ

Forces on a pendulum when it is displaced. Assuming θ < 10°, the small angle approximation can be used to describe the time period of a simple pendulum such as this.
Worked Example
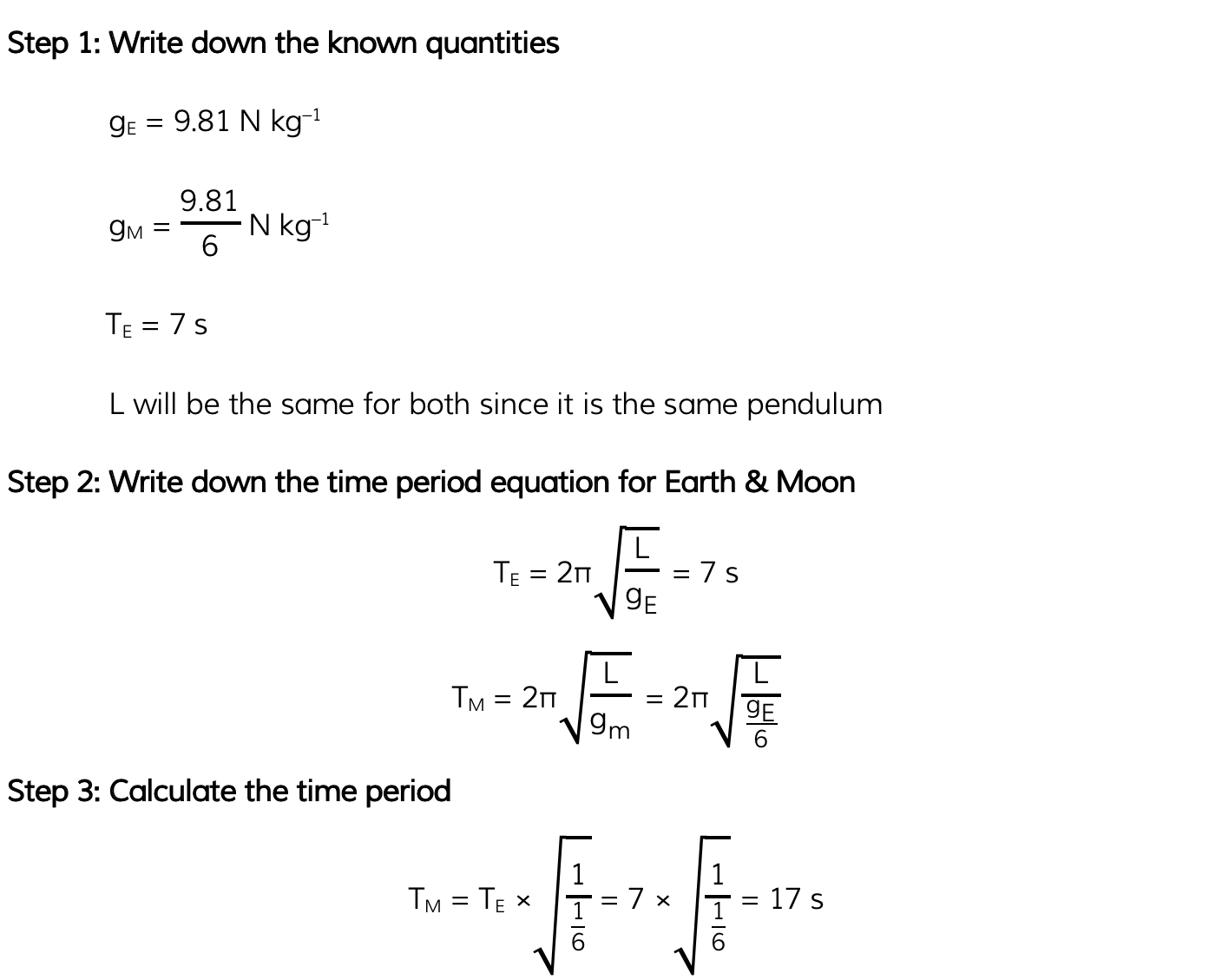
Calculate the time period of a simple pendulum on the Moon, if on Earth it has a time period of 7 s. g on the moon is 1/6 of that on Earth.
Answer:

You've read 0 of your 5 free revision notes this week
Sign up now. It’s free!
Did this page help you?
