Electromotive Force & Internal Resistance (AQA A Level Physics) : Revision Note
Electromotive Force
When charge passes through a power supply such as a battery, it gains electrical energy
The electromotive force (e.m.f) is defined as:
The amount of chemical energy converted to electrical energy per coulomb of charge (C) when passing through a power supply

This can also be written as:
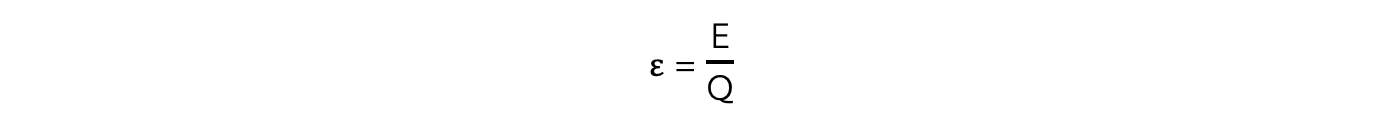
E.m.f can be represented by the symbol ε (greek letter epsilon)
It is not actually a force, and is measured in volts (V)
E.m.f is equal to the potential difference across the cell when no current is flowing
E.m.f can be measured by connecting a high-resistance voltmeter around the terminals of the cell in an open circuit, as so:

e.m.f is measured using a voltmeter connected in parallel with the cell
The terminal potential difference (p.d) is the potential difference across the terminals of a cell
If there was no internal resistance, the terminal p.d would be equal to the e.m.f
It is defined as:
V = IR
Where:
V = terminal p.d (V)
I = current (A)
R = resistance (Ω)
Since a cell has internal resistance, the terminal p.d is always lower than the e.m.f
In a closed circuit, current flows through a cell and a potential difference develops across the internal resistance
Since resistance opposes current, this reduces the energy per unit charge (voltage) available to the rest of the external circuit
This difference is called the ‘lost volts'
Lost volts is usually represented by little v
It is defined as
The work done per unit charge / coulomb to overcome the internal resistance / resistance inside the battery (when current flows)
In other words, this is the voltage lost in the cell due to internal resistance
So, from conservation of energy: v = e.m.f − terminal p.d
v = ε – V = Ir (Ohm’s law)
Where:
v = lost volts (V)
I = current (A)
r = internal resistance of the battery (Ω)
ε = e.m.f (V)
V = terminal p.d (V)
The e.m.f is the sum of these potential differences, giving the equation below:
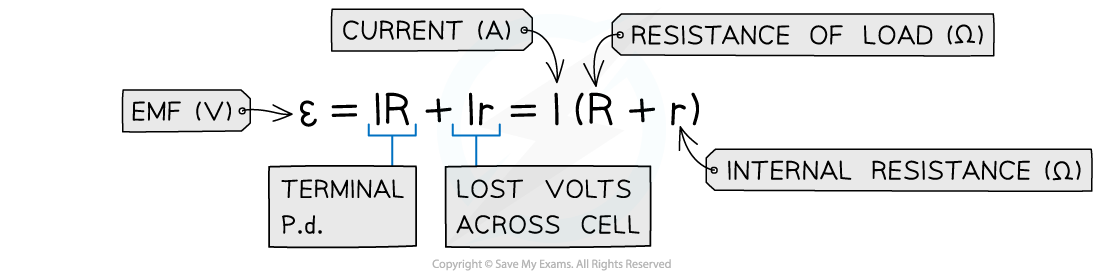
E.m.f can therefore be defined as the total, or maximum, voltage available to the circuit
Internal Resistance
All power supplies have some resistance between their terminals
This is called internal resistance (r)
Internal resistance is defined as:
The resistance of the materials within the battery
It is internal resistance that causes the charge circulating to dissipate some electrical energy from the power supply itself
This is why the cell becomes warm after a period of time
Therefore, over time the internal resistance causes loss of voltage or energy loss in a power supply
A cell can be thought of as a source of e.m.f with an internal resistance connected in series. This is shown in the circuit diagram below:
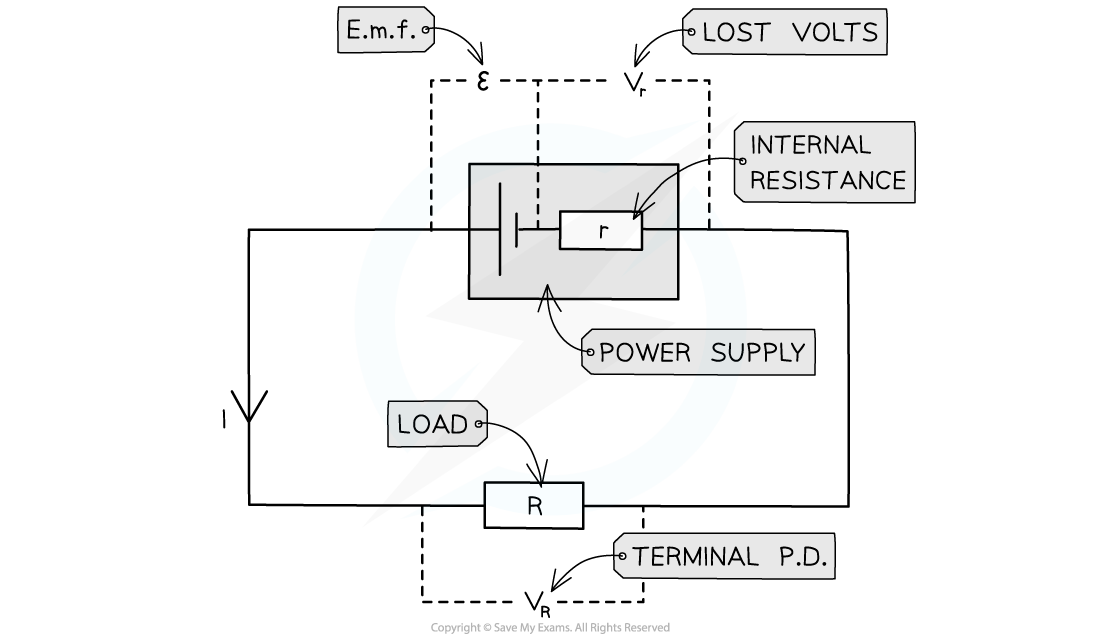
Circuit showing the e.m.f and internal resistance of a power supply
Where:
Resistor R is the 'load resistor'
r is the internal resistance
ε is the e.m.f
Vr is the lost volts
VR is the p.d across the load resistor, which is the same as the terminal p.d
Worked Example
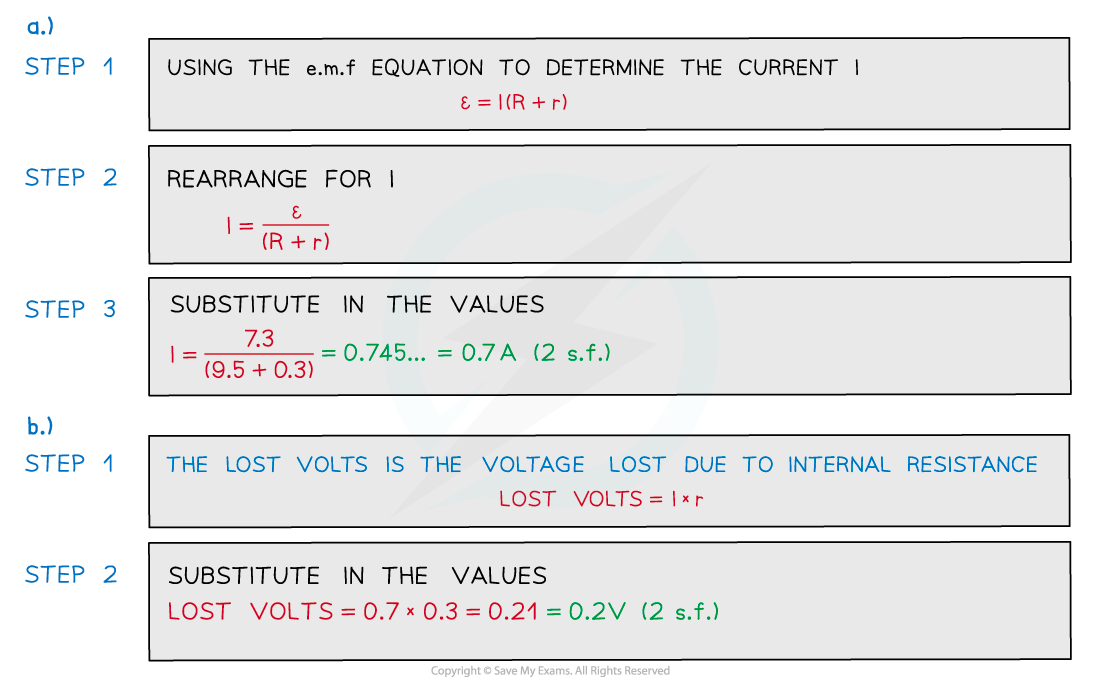
A battery of e.m.f 7.3 V and internal resistance r of 0.3 Ω is connected in series with a resistor of resistance 9.5 Ω.

Determine:
a) The current in the circuit
b) Lost volts from the battery
Answer:
Examiner Tips and Tricks
If the exam question states 'a battery of negligible internal resistance', this assumes that e.m.f of the battery is equal to its voltage. Internal resistance calculations will not be needed here.If the battery in the circuit diagram includes internal resistance (like that in the worked example), then the e.m.f equations must be used.

You've read 0 of your 5 free revision notes this week
Sign up now. It’s free!
Did this page help you?
