Impulse on a Force-Time Graph (AQA A Level Physics)

Impulse on a Force-Time Graph

• In real life, forces are often not constant and will vary over time

• If the force is plotted against time, the impulse is equal to the area under the force-time graph

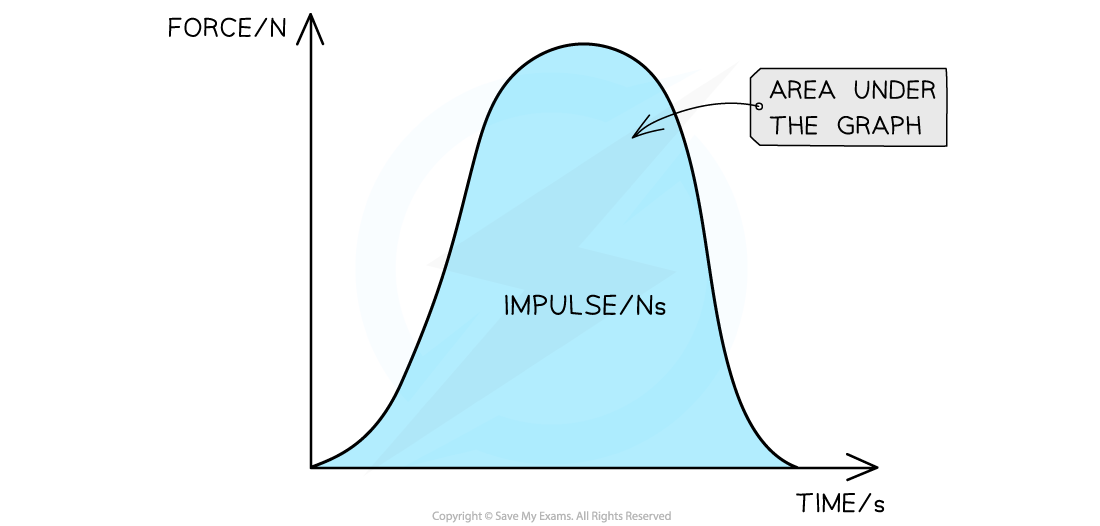
When the force is not constant, the impulse is the area under a force–time graph

• This is because

Impulse = Force × Change in time

• The impulse is therefore equal whether there is a small force over a long period of time or a large force over a small period of time

• The force-time graph may be a curve or a straight line

  • If the graph is a curve, the area can be found by counting the squares underneath

  • If the graph is made up of straight lines, split the graph into sections. The total area is the sum of the areas of each section

Impact Forces

• Impact forces are reduced by increasing the contact time

• This fact is used in everyday life to lower the risk of injury

• Some example of where reducing impact force is important:

  • In sport

  • In packaging

In Sports

• For example, in cricket, when a fielder relaxes their hands and pulls them back when catching a ball

  • A cricket ball travels at very high speeds and therefore has a high momentum

  • When a fielder catches the ball, it exerts a force onto their hands

  • Stopping a ball with high momentum instantly will cause a large force on their hands

  • This is because a change in momentum (impulse) acts over a short period of time which creates a large force on the fielder's hands and could cause serious injury

  • A fielder moves their hands back when they catch the ball, which increases the time for its change in momentum to reduce

  • This means there will be less force exerted on the fielder's hands and, therefore, less chance of injury

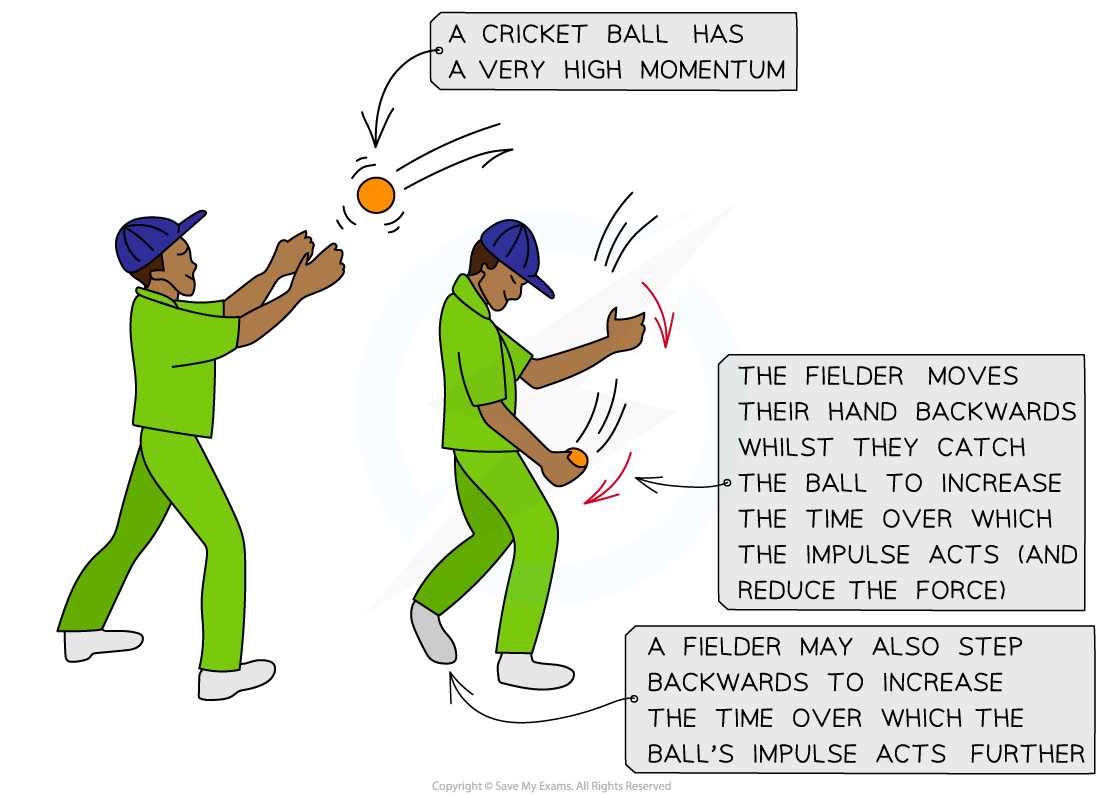
A cricket fielder moves their hands backwards when catching a cricket ball to reduce the force it will exert on their hands

• In football:

  • Increasing the contact time is sometimes used to advantage, as the longer the contact time, the larger change in momentum

  • When kicking a football, after a strong kick the motion is followed through

  • The momentum from the foot is transferred to the ball

  • This creates a large impulse and the ball then has a higher velocity

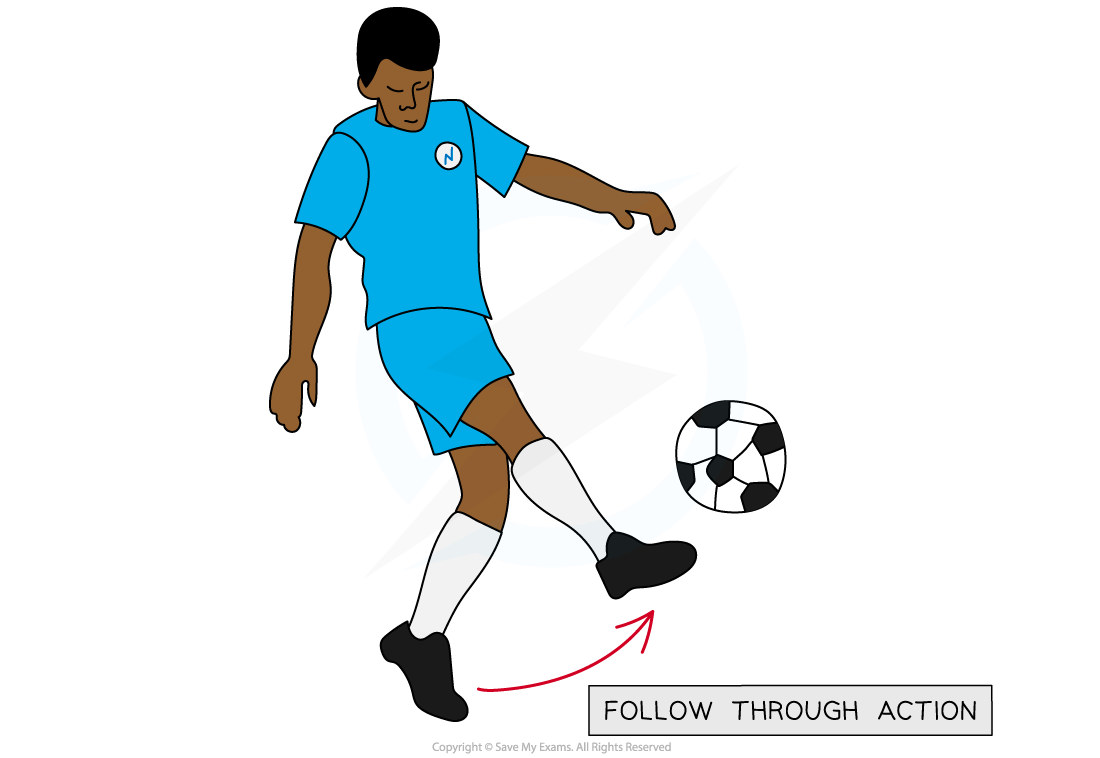
The follow through action of a football kick increases the change in momentum of the ball

In Packaging

• Packaging, especially for fragile items, uses bubble wrap or polyester packaging to reduce the impact forces that items experience in transit

• These help cushion the items by increasing the time over which they experience a force, which reduces the risk of damage