Motion Along a Straight Line (AQA A Level Physics): Revision Note
Exam code: 7408
Displacement, Speed, Velocity & Acceleration
Scalar quantities
Scalar quantities only have a magnitude (size)
Distance: the total length between two points
Speed: the total distance travelled per unit of time
Vector quantities
Vector quantities have both magnitude and direction
Displacement: the distance of an object from a fixed point in a specified direction
Velocity: the rate of change of displacement of an object
Acceleration: the rate of change of velocity of an object
Equations for Velocity & Acceleration
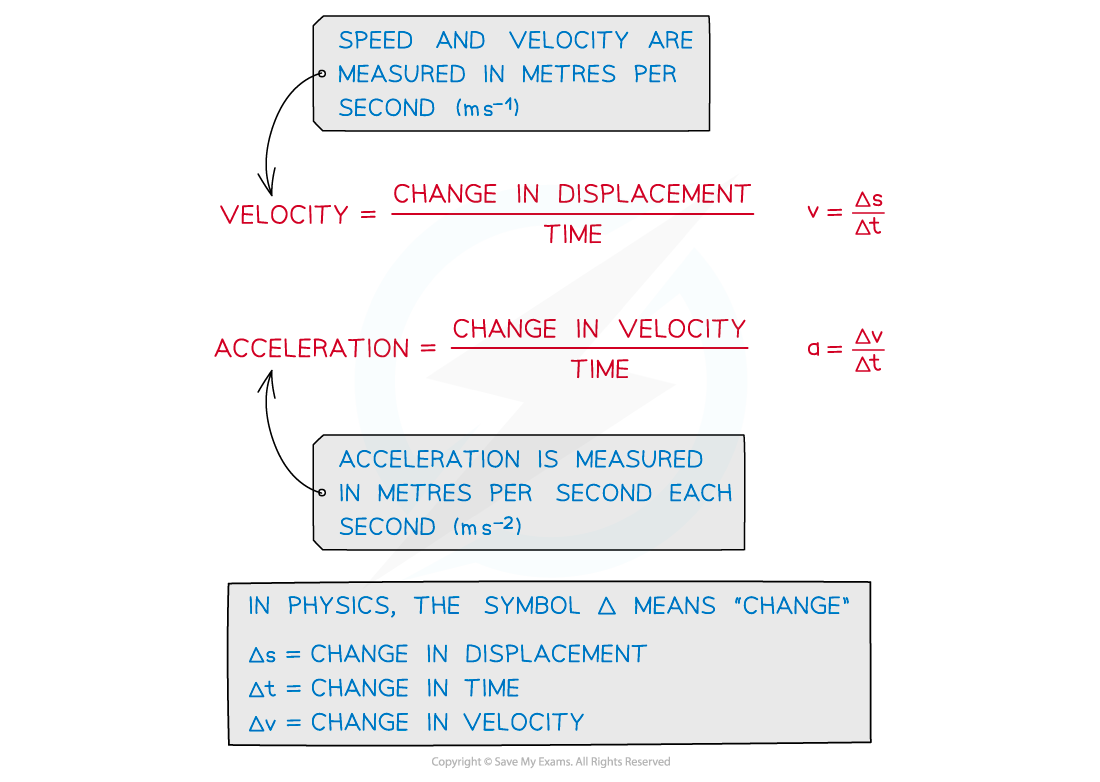
Equations linking displacement, velocity and acceleration
Worked Example
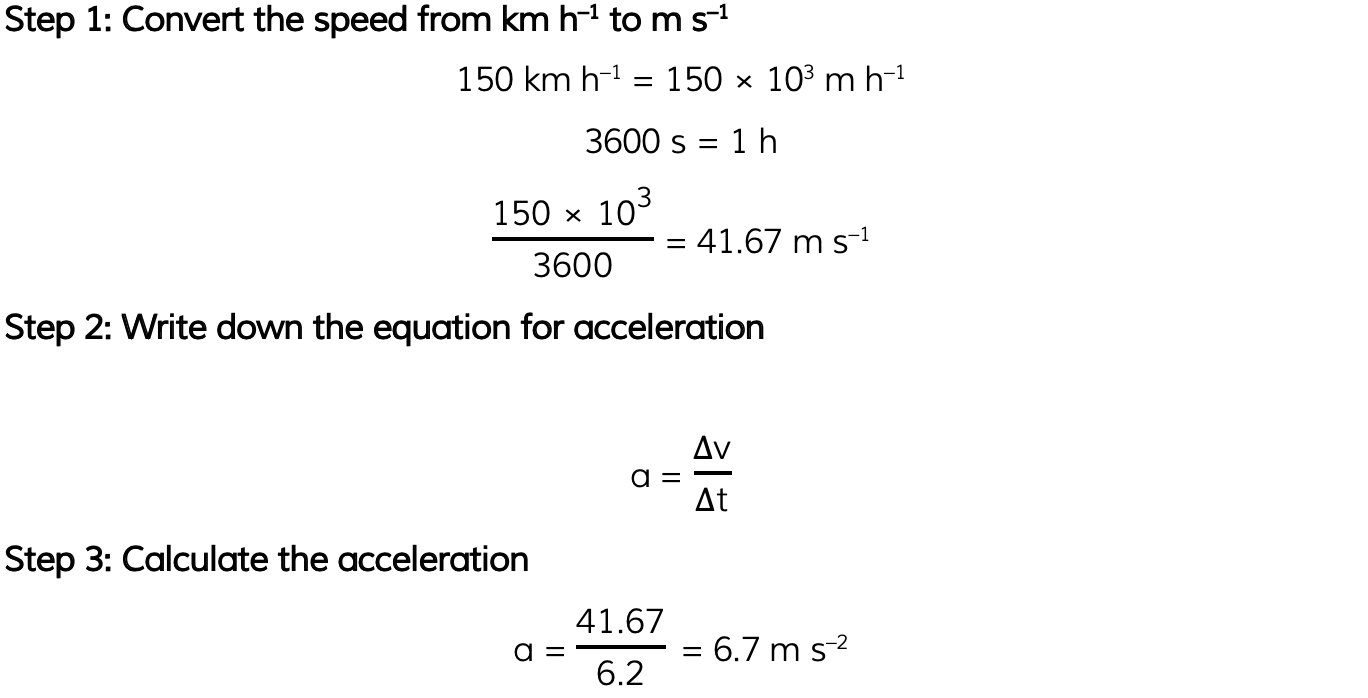
A car accelerates uniformly from rest to a speed of 150 km h–1 in 6.2 s.Calculate the magnitude of the acceleration of the car in m s–2.
Answer:
Average & Instantaneous Speed
Instantaneous Speed / Velocity
The instantaneous speed (or velocity) is the speed (or velocity) of an object at any given point in time
This could be for an object moving at a constant velocity or accelerating
An object accelerating is shown by a curved line on a displacement – time graph
An accelerating object will have a changing velocity
To find the instantaneous velocity on a displacement-time graph:
Draw a tangent at the required time
Calculate the gradient of that tangent
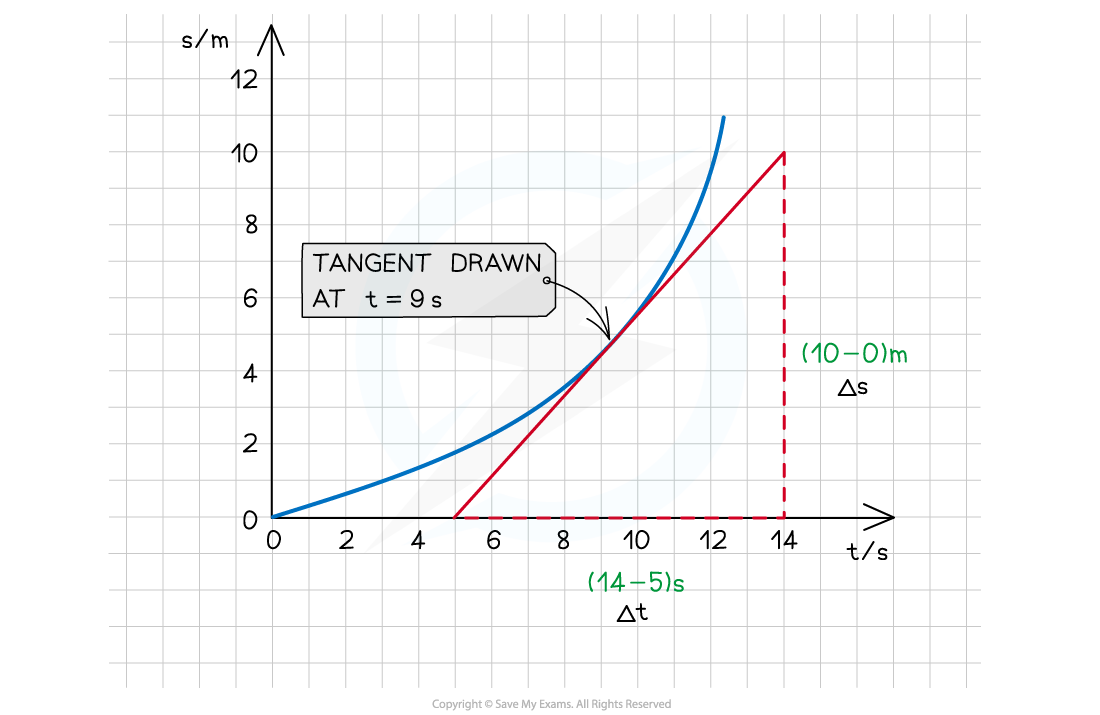
The instantaneous velocity is found by drawing a tangent on the displacement time graph
Average Speed / Velocity
The average speed (or velocity) is the total distance (or displacement) divided by the total time
To find the average velocity on a displacement-time graph, divide the total displacement (on the y-axis) by the total time (on the x-axis)
This method can be used for both a curved or a straight-line on a displacement-time graph
Worked Example
A cyclist travels a distance of 20 m at a constant speed then decelerates to a traffic light 5 m ahead. The whole journey takes 3.5 s. Calculate the average speed of the cyclist.
Answer:
Step 1: Write the average speed equation
Average speed = total distance ÷ total time
Step 2: Calculate the total distance
Total distance = 20 + 5 = 25 m
Step 3: Calculate the average speed
Average speed = 25 ÷ 3.5 = 7.1 m s-1
Uniform & Non-Uniform Acceleration Graphs
Three types of graph that can represent motion are displacement-time graphs, velocity-time graphs and acceleration-time graphs
Displacement-Time Graph
On a displacement-time graph:
The gradient (or slope) equals velocity
The y-intercept equals the initial displacement
A diagonal straight line represents a constant velocity
A positive slope represents motion in the positive direction
A negative slope represents motion in the negative direction
A curved line represents an acceleration
A horizontal line (zero slope) represents a state of rest
The area under the curve is meaningless
Remember the displacement-time graph can have positive or negative values on the displacement axis. However, a distance-time graph only has positive
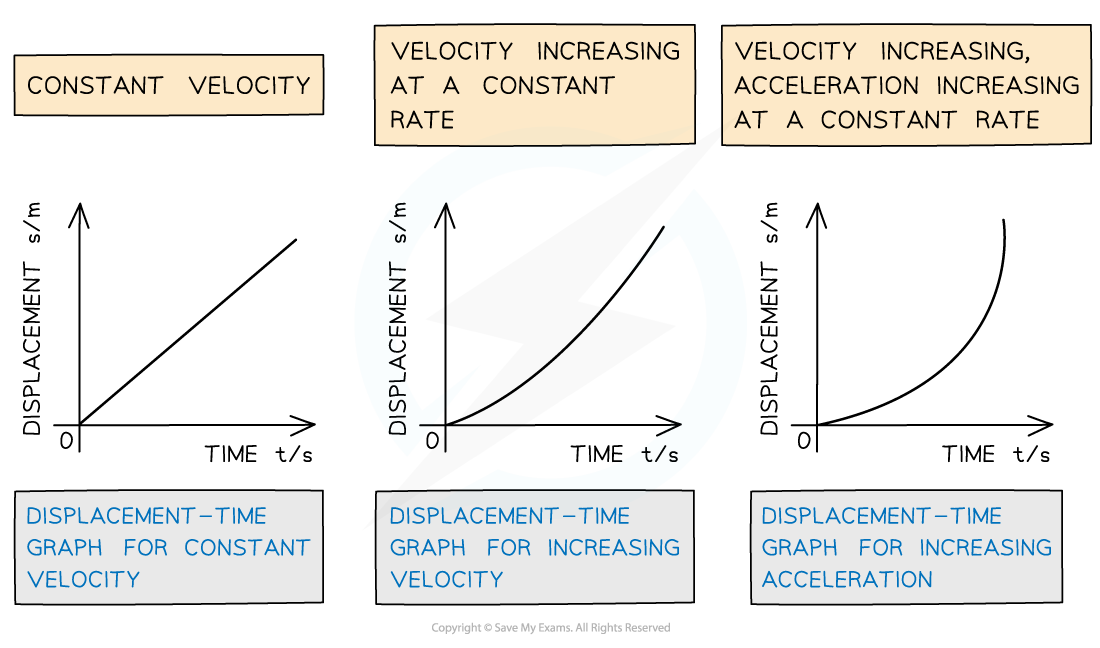
Displacement-time graph for different scenarios
Velocity-Time Graph
On a velocity-time graph:
Slope equals acceleration
The y-intercept equals the initial velocity
A straight line represents uniform acceleration
A positive slope represents an increase in velocity (acceleration) in the positive direction
A negative slope represents an increase in velocity (acceleration) in the negative direction
A curved line represents the non-uniform acceleration
A horizontal line (zero slope) represents motion with constant velocity
The area under the curve equals the displacement or distance travelled
Remember the velocity-time graph can have positive or negative values on the displacement axis. However, a speed-time graph only has positive
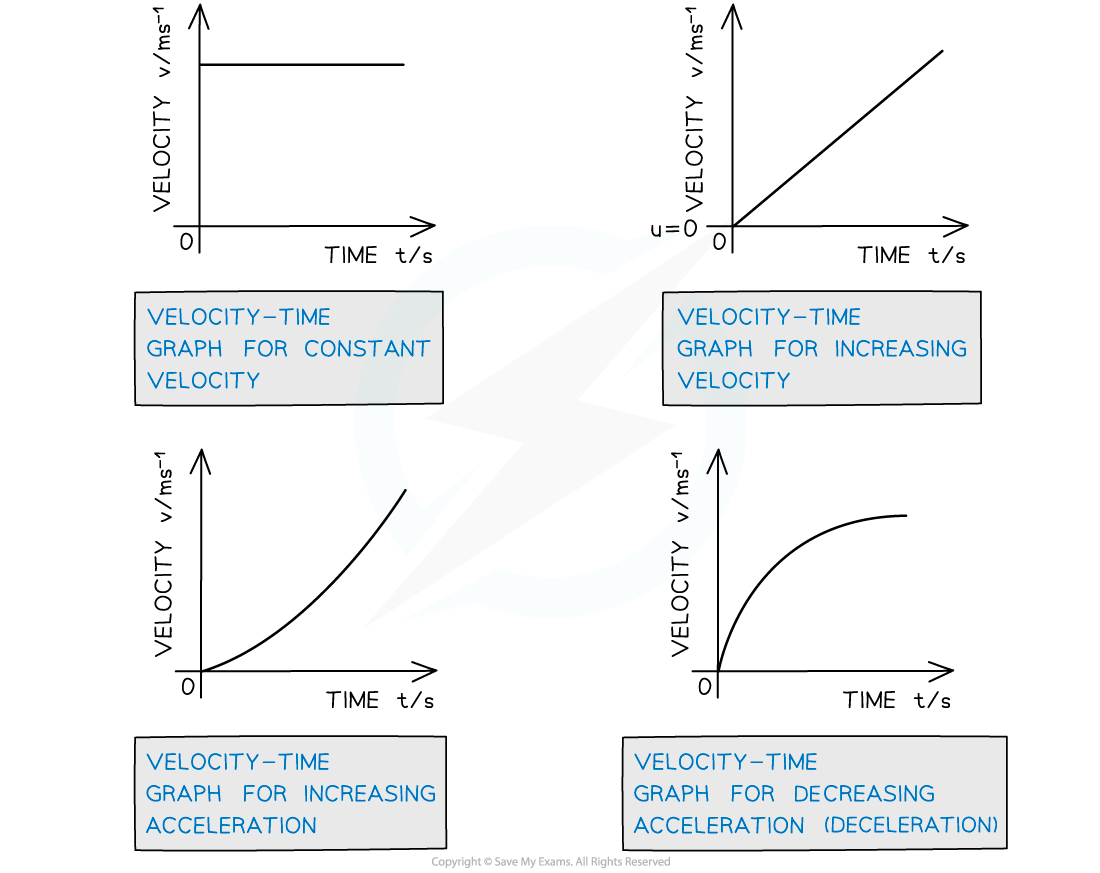
Velocity-time graph for different scenarios
Acceleration-Time Graph
On an acceleration-time graph:
The slope is meaningless
The y-intercept equals the initial acceleration
A horizontal line (zero slope) represents an object undergoing constant acceleration
The area under the curve equals the change in velocity
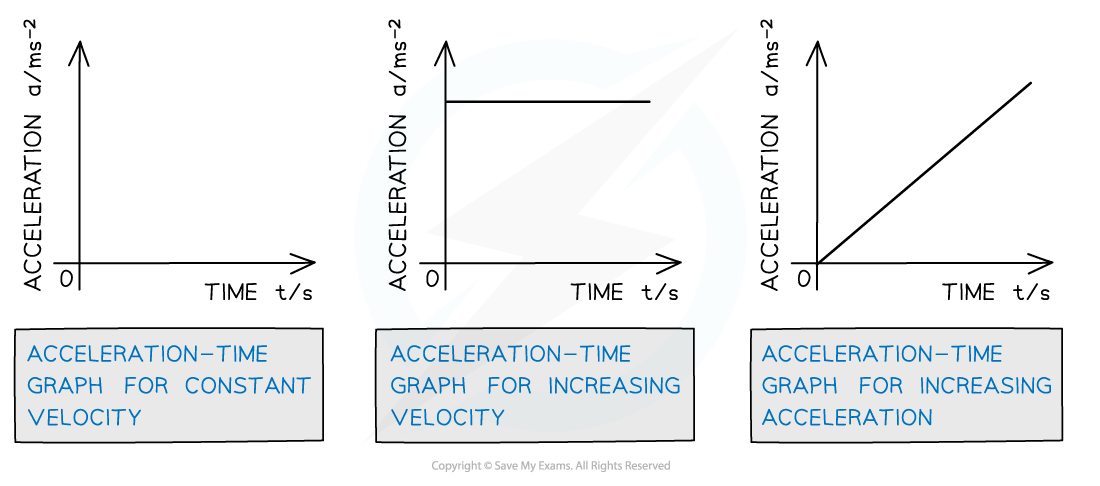
Acceleration-time graphs for different velocity scenarios

Unlock more, it's free!
Did this page help you?