Muon Lifetime Experiment (AQA A Level Physics): Revision Note

Muon Lifetime Experiment

• Muon decay experiments provide experimental evidence for time dilation and length contraction

• Muons are unstable subatomic particles that are around 200 times heavier than an electron and are produced in the upper atmosphere (as a result of pion decays produced by cosmic rays)

• Muons travel at 0.98c  and have a half-life of 1.6 µs (or mean lifetime of 2.2 µs)

  • The distance they travel in one half-life is therefore around 470 m

• Muons are created around 10 km above the surface

  • From the value above calculated with Newtonian physics, very few muons are expected to reach the surface

  • Roughly 21 half-lives pass over the course of 10 km

  • However, in practice, we find that a considerably larger number of muons can be detected on the Earth's surface

• The detection of the muons is a result of time dilation (or length contraction, depending on the viewpoint of the observer)

Muon decay from time dilation

• From the muon's own reference frame, its half-life is 1.6 μs

• For an observer on Earth, however, the muon is travelling close to the speed of light, so its half-life is dilated

• We can see this from the time dilation equation

$∆t=\gamma ∆t_0$

• Where:

  • the gamma factor, $\gamma =\frac{1}{\sqrt{1-{\left(0.98\right)}^2}}=5$

  • $∆t$ = the half-life measured by an observer on Earth

  • $∆t_0$ = the proper time for the half-life measured in the muon's inertial frame

• Therefore, in the reference frame of an observer on Earth, the muons have a lifetime of

$∆t=5\times 1.6=8\mu \mathrm{s}$

• According to the observer on Earth, the muons travel 10 km at a speed of 0.98c, taking 34 μs

  • This is 4.3 half-lives as seen by the observer on Earth

  • This is far fewer half-lives than the Newtonian prediction of 21 half-lives, so this correctly explains why a larger number of muons reach the Earth's surface before decaying

Muon decay from length contraction

• From the Earth's reference frame, the muons are perceived to cover a distance of 10 km

• However, according to the muons in their own reference frame, length is contracted, so they perceive the distance travelled as shorter than 10 km

• We can see this from the length contraction equation:

$L=\frac{L_0}{\gamma }$

• Where:

  • $L_0$ = the proper length for the distance measured in the Earth's inertial frame

  • $L$= the distance measured by an observer in the muon's reference frame

• Therefore, in the muon's reference frame, they only have to travel a distance:

$L=\frac{10000}{5}=2000\mathrm{m}$

• To travel this distance takes a time of $\frac{2000}{0.98c}$ = 6.8 µs, according to an observer in the muon's reference frame

• This is 4.3 half-lives again, so a significant number of muons remain undecayed at the Earth's surface

Proper time and length

• Proper time is the time measured in the reference frame which is stationary relative to the event being timed

  • Here, that event is the muon decaying

  • The muon is stationary relative to itself, so proper time is measured in the muon's reference frame

• Proper length is the length measured in the reference frame stationary relative to the distance being measured

  • Here, that distance is the path from where the muon is created to the Earth's surface

  • The observer on Earth is stationary relative to that distance, so proper length is measured in the Earth's reference frame

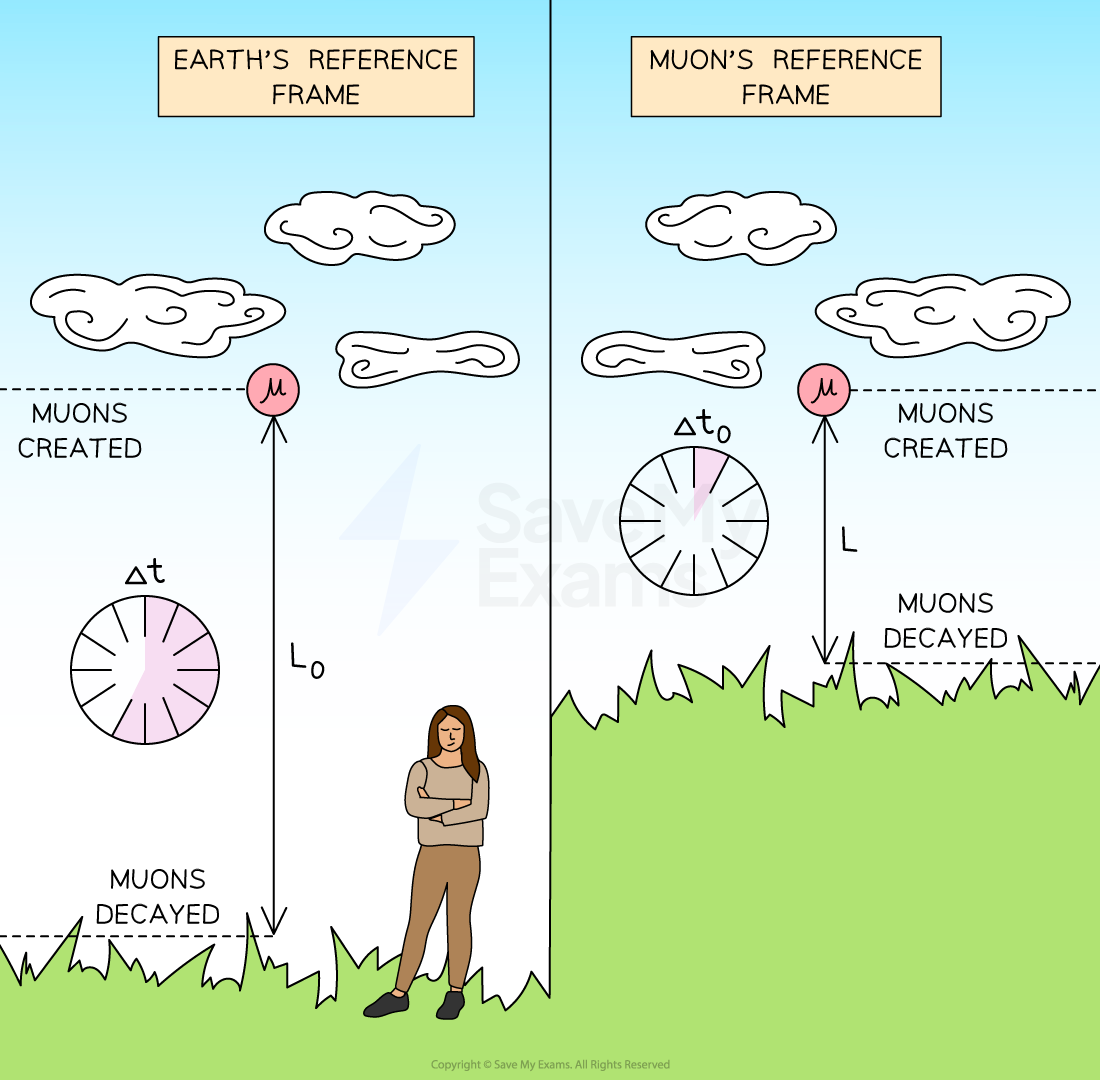
Muon decay from the Earth's reference frame and a muon's reference frame