A LevelPhysicsAQARevision Notes11. Engineering Physics11.1 Rotational Dynamics11.1.7 Angular Impulse

Angular Impulse (AQA A Level Physics)

Revision Note

Ashika

Author

Ashika

Last updated

Angular Impulse

  • In linear motion, the resultant force on a body can be defined as the rate of change of linear momentum:

F space equals space fraction numerator increment p over denominator increment t end fraction

  • This leads to the definition of linear impulse:

An average resultant force F acting for a time increment t produces a change in linear momentum increment p

increment p space equals space F increment t space equals space increment open parentheses m v close parentheses

  • Similarly, the resultant torque on a body can be defined as the rate of change of angular momentum:

tau space equals space fraction numerator increment L over denominator increment t end fraction

  • Where:
    • tau = resultant torque on a body (N m)
    • increment L = change in angular momentum (kg m2 s−1)
    • increment t = time interval (s)
  • This leads to the definition of angular impulse:

An average resultant torque tau acting for a time increment t produces a change in angular momentum increment L

increment L space equals space tau increment t space equals space increment open parentheses I omega close parentheses

  • Angular impulse is measured in kg m2 s−1, or N m s
  • This equation requires the use of a constant resultant torque
    • If the resultant torque changes, then an average of the values must be used
  • Angular impulse describes the effect of a torque acting over a time interval
    • This means a small torque acting over a long time has the same effect as a large torque acting over a short time

Angular Impulse on a Torque-Time Graph

  • The area under a torque-time graph is equal to the angular impulse or the change in angular momentum
    • This is because the area, angular impulse, is a fraction of the base × height, torque × time, increment L space equals space tau increment t
    • The fraction of torque × time depends upon the shape of the area under the graph

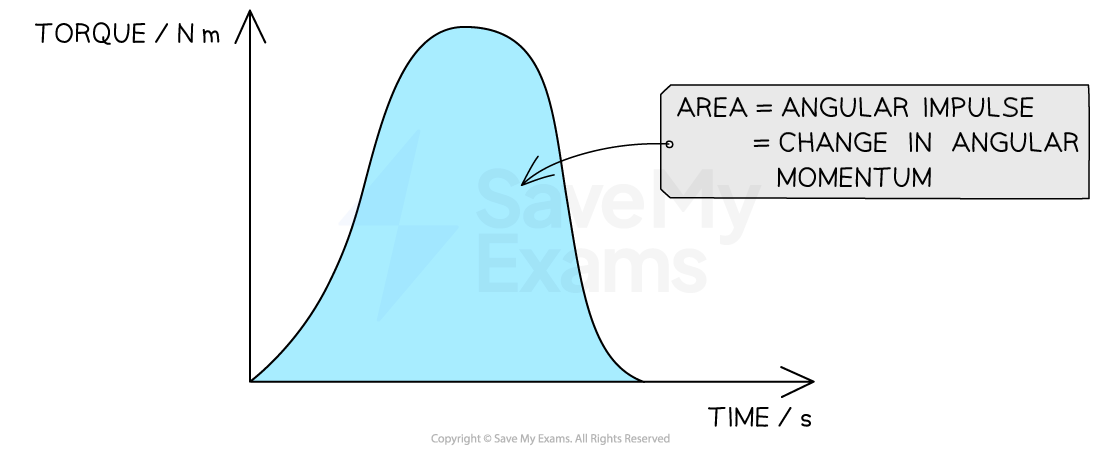
1-4-8--angular-impulse-on-a-torque-time-graph-ib-2025-physics

When the torque is not constant, the angular impulse is the area under a torque–time graph

Worked example

The graph shows the variation of time t with the net torque tau on an object which has a moment of inertia of 6.0 kg m2.

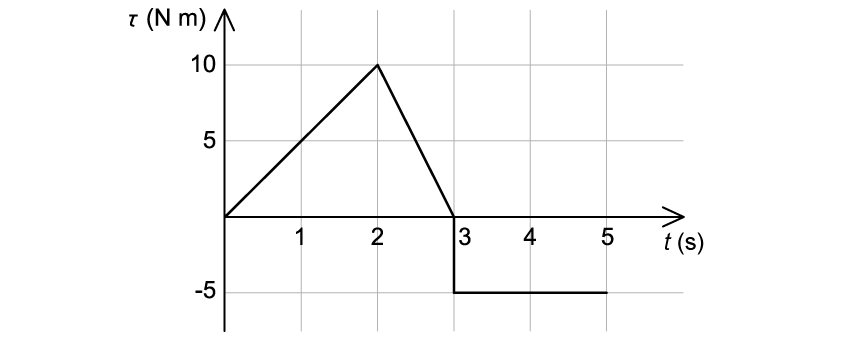
1-4-8-angular-impulse-graph-worked-example-ib-2025-physics

At t = 0, the object rotates with an angular velocity of 2.0 rad s−1 clockwise.

Determine the magnitude and direction of rotation of the angular velocity at t = 5 s.

In this question, take anticlockwise as the positive direction.

Answer:

  • The area under a torque-time graph is equal to angular impulse, or the change in angular momentum

increment L space equals space tau cross times increment t

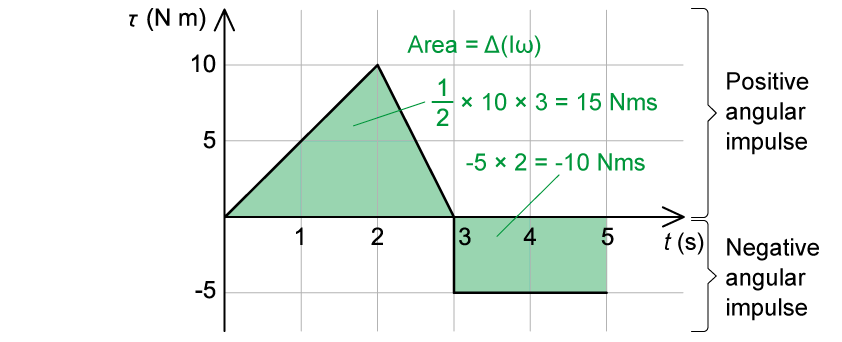
1-4-8-angular-impulse-graph-worked-example-ma-ib-2025-physics

  • The area under the positive curve (triangle) = 1 half cross times 10 cross times 3 space equals space 15 space straight N space straight m space straight s
  • The area under the negative curve (rectangle) = negative 5 cross times 2 space equals space minus 10 space straight N space straight m space straight s
  • Therefore, the overall change in angular momentum is

increment L space equals space 15 space minus space 10 space equals space 5 space straight N space straight m space straight s

  • The change in angular momentum is equal to

increment L space equals space increment open parentheses I omega close parentheses space equals space I open parentheses omega subscript f space minus space omega subscript i close parentheses

  • Where
    • Moment of inertia, I = 6.0 kg m2
    • Initial angular velocity, omega subscript i = −2.0 rad s−1 (clockwise is the negative direction)
  • Therefore, when t = 5 s, the angular velocity is

6 cross times open parentheses omega subscript f space minus space open parentheses negative 2 close parentheses close parentheses space equals space 5

6 omega subscript f space plus open parentheses 2 cross times 6 close parentheses space equals space 5

6 omega subscript f space equals space 5 space minus space 12

omega subscript f space equals space fraction numerator negative 7 over denominator 6 end fraction space equals space minus 1.17

Anti-clockwise is positive, so omega subscript f = 1.17 rad s−1 in the clockwise direction

Examiner Tip

Many applications for angular impulse will be related to sports. These are similar to the linear impulse topic.

You've read 0 of your 5 free revision notes this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

    Did this page help you?

    Previous:11.1.6 Angular MomentumNext:11.1.8 Rotational Work & Power
    Ashika

    Author: Ashika

    Expertise: Physics Project Lead

    Ashika graduated with a first-class Physics degree from Manchester University and, having worked as a software engineer, focused on Physics education, creating engaging content to help students across all levels. Now an experienced GCSE and A Level Physics and Maths tutor, Ashika helps to grow and improve our Physics resources.