Revision NotesExam QuestionsPast Papers
A LevelPhysicsAQARevision NotesMedical PhysicsX-ray ImagingProduction of X-rays

Production of X-rays (AQA A Level Physics)

Revision Note

Katie M

Author

Katie M

Last updated

Production of X-rays

  • When the fast-moving electrons collide with the target, X-rays are produced by one of two methods

    • Method 1: Bremsstrahlung

    • Method 2: Characteristic Radiation

Method 1: Bremsstrahlung

  • When high-speed electrons collide with a metal target (often tungsten), they undergo a steep deceleration

    • When a charged particle decelerates quickly, some of the energy released is converted into a photon

  • A small amount of the kinetic energy (~ 1%) from the incoming electrons is converted into X-rays as the electrons decelerate in the tungsten, due to conservation of energy 

    • The rest of the energy heats up the anode, which usually requires some form of cooling

  • The energy of the X-ray photon can be of any value, up to the original kinetic energy of the electron, giving a range of possible X-ray energies

    • These X-rays cause the continuous or ‘smooth hump shaped’ line on an intensity wavelength graph

Ranges of Wavelengths in Bremsstrahlung Radiation

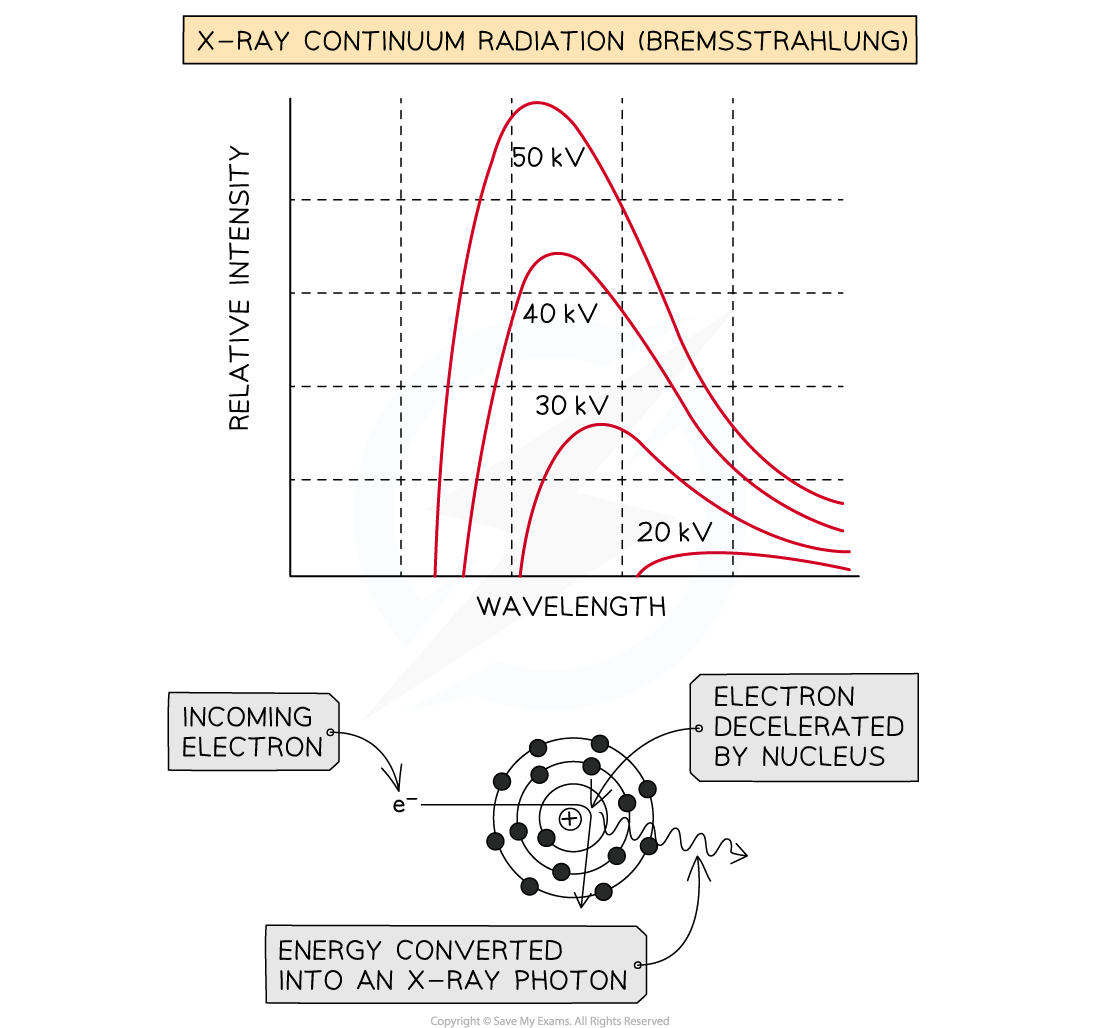
6-11-1-bremsstrahlung-graph_ocr-al-physics

The continuous spectra of Bremsstrahlung radiation at different acceleration potentials. As wavelength decreases, the energy of the X-rays photons increases.

  • When an electron is accelerated, it gains energy equal to the product of its charge and the accelerating potential, V , this energy can be calculated using:

Emax = eV

  • This is the maximum energy that an X-ray photon can have

  • The smallest possible wavelength is equivalent to the highest possible frequency and therefore, the highest possible energy

    • This is assuming all of the electron’s kinetic energy has turned into electromagnetic energy

  • Therefore, the maximum X-ray frequency fmax, or the minimum wavelength λmin, that can be produced is calculated using the equation:

E subscript m a x end subscript equals e V equals h f subscript m a x end subscript equals fraction numerator h c over denominator lambda subscript m i n end subscript end fraction

  • The maximum X-ray frequency, fmax, is therefore equal to:

f subscript m a x end subscript equals fraction numerator e V over denominator h end fraction

  • The minimum X-ray wavelength, λmin, is therefore equal to:

lambda subscript m i n end subscript equals fraction numerator h c over denominator e V end fraction

  • Where:

    • e = elementary charge (C)

    • V = potential difference between the anode and cathode (V)

    • h = Planck's constant (J s)

    • c = the speed of light (m s−1)

Method 2: Characteristic Radiation

  • Some of the incoming fast electrons cause inner shell electrons of the tungsten to be ‘knocked out’ of the atom, leaving a vacancy

    • This vacancy is filled by an outer electron moving down and releasing an X-ray photon as it does (equal in energy to the difference between the two energy levels)

    • Because these X-rays are caused by energy level transitions, they have only specific discrete energies

    • They cause sharp spikes on an intensity wavelength graph

    • The number of spikes depends on the element used for the target - there are two sets of spikes for a tungsten target, representing two sets of possible energy transitions

Characteristic Discrete X-Ray Wavelengths

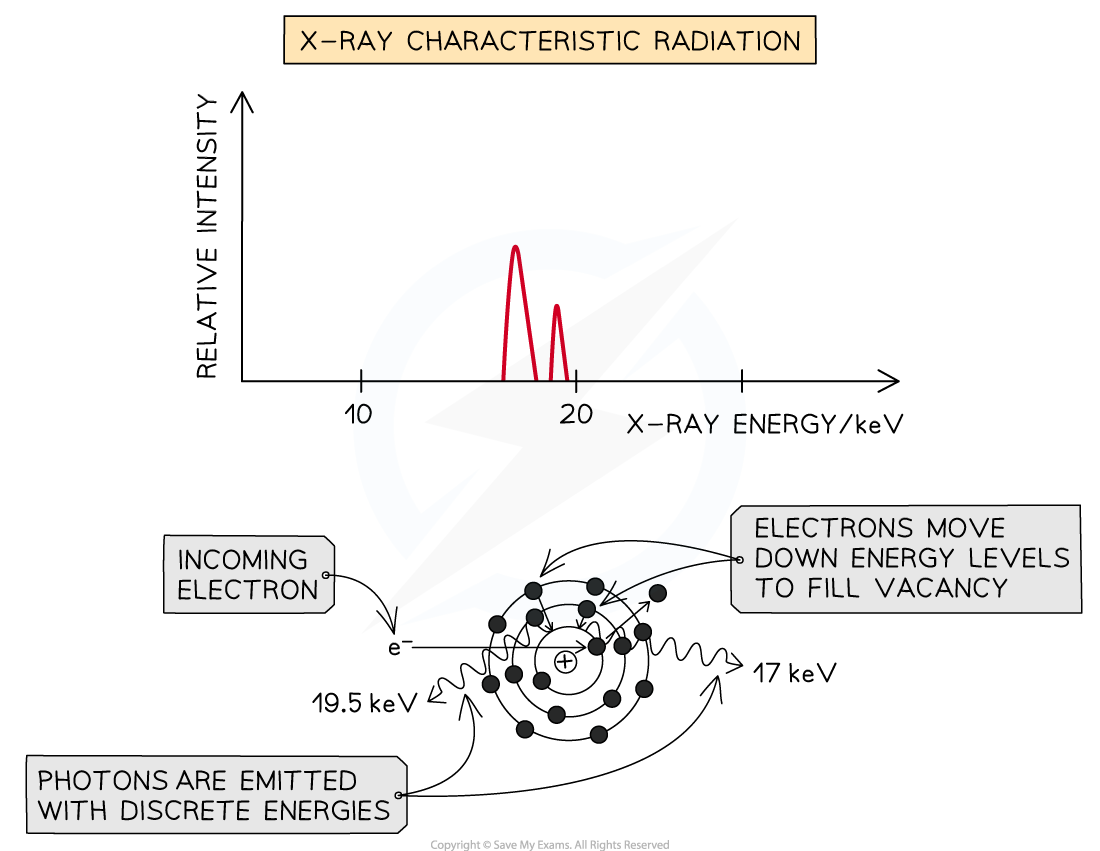
6-11-1-characteristic-xray-graph_ocr-al-physics

Electron transitions emit photons with discrete energies. An incoming electron can cause these transitions, making tungsten emit characteristic photons.

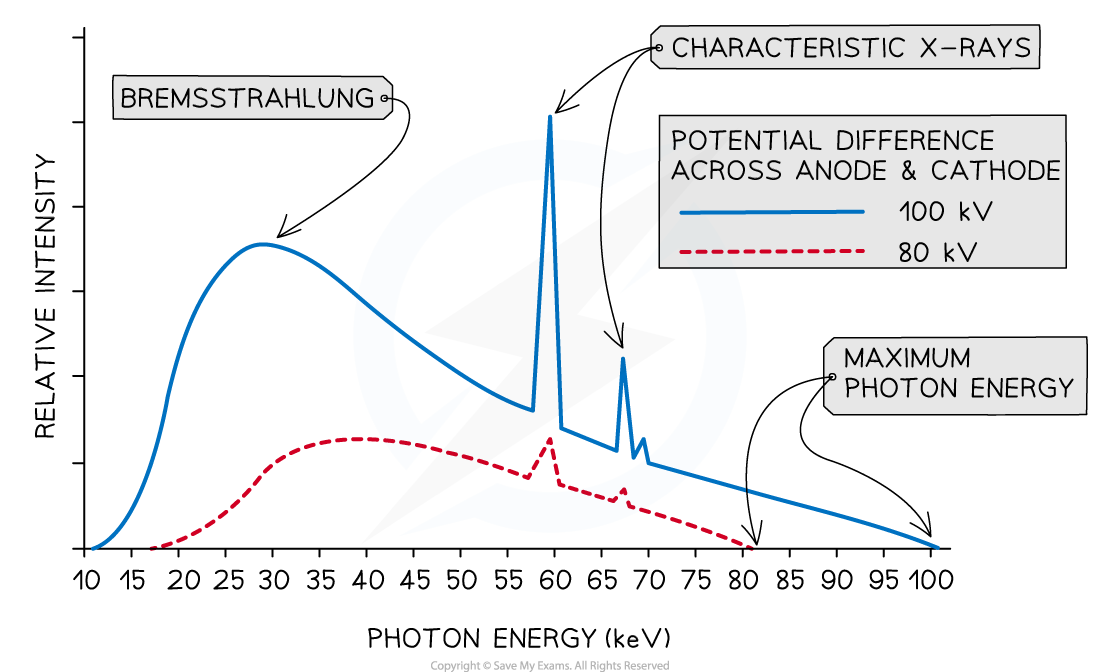
Combined X-Ray Spectra of Bremsstrahlung and Characteristic Photons

6-11-1-xray-combined-graph_ocr-al-physics

Worked Example

X-rays are a type of electromagnetic wave with wavelengths in the range 10−8 to 10−13 m

If the accelerating potential difference in an X-ray tube is 60 kV, determine if the photons emitted fall within this range.

Answer:

Step 1: Write out known quantities

  • Charge on an electron, e = 1.6 × 10−19 C

  • Accelerating potential difference, V = 60 000 V

  • Planck’s constant, h = 6.63 × 10−34 J s

  • Speed of light, c = 3 × 108 m s−1

 

Step 2: Determine the maximum possible energy of a photon

  • The maximum possible energy of a photon corresponds to the maximum energy an electron could have:

Emax = eV

Step 3: Determine an expression for minimum wavelength

Planck relation:    E = hf

Wave equation:    c = fλ

  • When energy is a maximum:

Emax = eV = hfmax

  • Maximum energy corresponds to a minimum wavelength:

e V equals fraction numerator h c over denominator lambda subscript m i n end subscript end fraction

  • Rearrange for minimum wavelength, λmin:

lambda subscript m i n end subscript equals fraction numerator h c over denominator e V end fraction

Step 4: Calculate the minimum wavelength λmin

lambda subscript m i n end subscript equals fraction numerator open parentheses 6.63 cross times 10 to the power of negative 34 end exponent close parentheses open parentheses 3 cross times 10 to the power of 8 close parentheses over denominator open parentheses 1.6 cross times 10 to the power of negative 19 end exponent close parentheses open parentheses 60 space 000 close parentheses end fraction

λmin = 2.1 × 10−11 m

Step 5: Comment on whether this is within the range for the wavelength of an X-ray 

  • X-ray wavelengths are within 10−8 to 10−13 m

  • The minimum wavelength for a 60 kV supply is 2.1 × 10−11 m, which means the photons produced will be X-rays

Worked Example

A typical spectrum of the X-ray radiation produced by electron bombardment of a metal target is shown below.

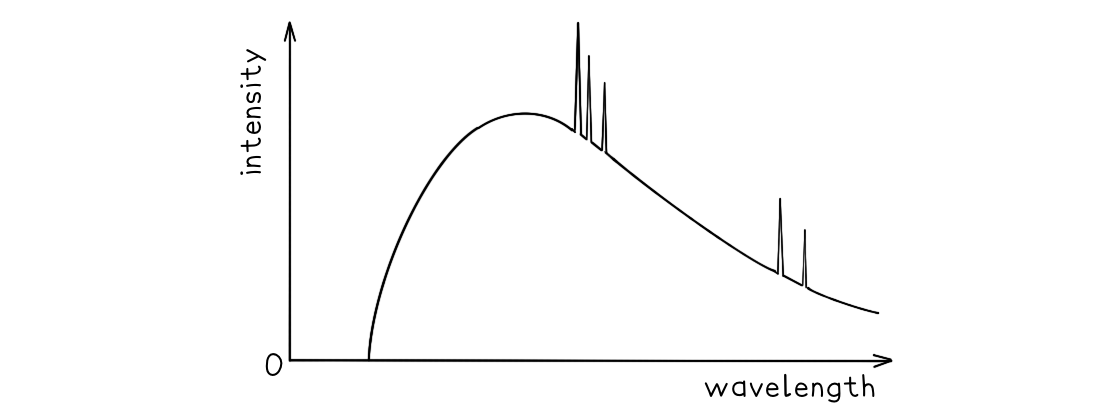
Explain why:

(a) A continuous spectrum of wavelengths is produced.

(b) The gradient is steeper at shorter wavelengths.

(c) The spectrum has a sharp cut-off at short wavelengths.

 Answer:

Part (a)

Step 1: Consider the path of the electrons from the cathode to the anode

  • Photons are produced whenever a charged particle undergoes a large acceleration or deceleration

  • X-ray tubes fire high-speed electrons at a metal target

  • When an electron collides with the metal target, it loses energy in the form of an X-ray photon as it decelerates

Step 2: Consider the relationship between the energy of the electron and the wavelength of the photon

  • The wavelength of a photon depends on the energy transferred by a decelerating electron

  • The electrons don't all undergo the same deceleration when they strike the target

  • This leads to a distribution of energies, hence, a range, or continuous spectrum, of wavelengths is observed

Part (b)

Step 1: Identify the significance of the intensity 

  • The intensity of the graph signifies the proportion of photons produced with a specific energy, or wavelength

  • The higher the intensity, the more photons of a particular wavelength are produced

  • In other words, the total intensity is the sum of all the photons with a particular wavelength

Step 2: Explain the shape of the graph

  • When a single electron collides with the metal target, a single photon is produced

  • Most electrons only give up part of their energy, and hence there are more X-rays produced at wavelengths higher than the minimum (or energies lower than the maximum)

  • At short wavelengths, there is a steeper gradient because only a few electrons transfer all, or most of, their energy

Part (c)

Step 1: Identify the relationship between minimum wavelength and maximum energy

  • The minimum wavelength of an X-ray is equal to

lambda subscript m i n end subscript equals fraction numerator h c over denominator E subscript m a x end subscript end fraction

  • The equation shows the maximum energy of the electron corresponds to the minimum wavelength, they are inversely proportional 

lambda subscript m i n end subscript proportional to 1 over E subscript m a x end subscript

  • Therefore, the higher the energy of the electron, the shorter the wavelength of the X-ray produced

Step 2: Explain the presence of the cut-off point

  • The accelerating voltage determines the kinetic energy which the electrons have before striking the target

  • The value of this accelerating voltage, therefore, determines the value of the maximum energy

  • This corresponds to the minimum, or cut-off, wavelength

You've read 0 of your 5 free revision notes this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

    Test yourself

    Did this page help you?

    Previous:Magnetic Resonance ImagingNext:Rotating-Anode X-ray Tube
    Katie M

    Author: Katie M

    Expertise: Physics

    Katie has always been passionate about the sciences, and completed a degree in Astrophysics at Sheffield University. She decided that she wanted to inspire other young people, so moved to Bristol to complete a PGCE in Secondary Science. She particularly loves creating fun and absorbing materials to help students achieve their exam potential.