Hypothesis Testing for Correlation (Edexcel A Level Maths): Revision Note
Exam code: 9MA0
Did this video help you?
Hypothesis testing for correlation
You should be familiar with using a hypothesis test to determine bias within probability problems. It is also possible to use a hypothesis test to determine whether a given product moment correlation coefficient calculated from a sample could be representative of the same relationship existing within the whole population. For full information on hypothesis testing, see the revision notes from section 5.1.1 Hypothesis Testing
Why use a hypothesis test for correlation?
In most cases it is too difficult to get the value of the PMCC for a whole population
This would involve having data on each individual within the whole population
It is very rare that a statistician would have the time or resources to collect all of that data
The PMCC for the whole population can instead be estimated using information from a sample taken from the population
The PMCC for a whole population is denoted
(pronounced rho)
The PMCC for a sample taken from the population is denoted r
A hypothesis test would be conducted using the value of to r determine whether the population can be said to have positive, negative or zero correlation
How do I use the table of critical values for correlation coefficients?
The table of critical values is found near the end of the Mathematical Formulae and Statistical Tables booklet you will have in your exam
It displays the minimum values that need to be reached by a value of r in order for the test to be significant
Find the significant value by looking at the value that is in both the row of the number in the sample, n, and in the column for the significance level of the test
Values are given for sample sizes of
and then 40, 50, 60, 70, 80, 90 and 100
Values are given for significance levels of 10% (0.1), 5% (0.05), 2.5% (0.025), 1% (0.01) and 0.5% (0.005)
The table gives values for one - tailed tests only
To find the significant values for two - tailed tests halve the significance level first and then carry out the test as if doing a one-tailed test
The table gives values for positive values of r only
To find the significant values if you are testing for negative correlation look for the corresponding positive value of r and change the sign to a negative
A two-tailed test will have two critical values (both the same value but one positive and one negative)
How do I lay out a hypothesis test for correlation?
Most of the time the hypothesis test will be carried out by using a critical value
You won't be expected to calculate p-values but you might be given a p-value
Step 1. Write the null and alternative hypotheses clearly
The hypothesis test could either be a one-tailed test or a two-tailed test
The null hypothesis will always be
The alternative hypothesis will depend on if it is a one-tailed or two-tailed test
A one-tailed test would test to see if the population PMCC, ρ, is either positive or negative
The alternative hypothesis, H1 will be
or
A two-tailed test would test to see if the population PMCC, ρ , is not equal to zero (meaning there is some form of linear correlation)
The alternative hypothesis, H1 will be
Step 2. Find the critical value for the test
The critical value will be determined by both the significance level of the test and the sample size,
For a positive value of r :
The greater the sample size, the lower r needs to be to determine that
is also positive
The greater the significance level the lower r needs to be to determine that
is also positive
The critical value for can be found by using the table of critical values given in the Mathematical Formulae and Statistical Tables booklet
Make sure you know how to find this and are familiar with using it before your exam
Step 3. Compare the value of r calculated from the sample with the critical value
If r is in the critical region the test is significant and the null hypothesis should be rejected
It will be in the critical region if
If r is not in the critical region the null hypothesis should be accepted and the alternative hypothesis should be rejected
Step 4. Write a conclusion in context
Use the wording in the question to help you write your conclusion
If rejecting the null hypothesis your conclusion should state that there is sufficient evidence to suggest the alternative hypothesis is true at this level of significance
If accepting the null hypothesis your conclusion should state that there is not enough evidence to suggest the alternative hypothesis is true at this level of significance
Occasionally the hypothesis test will be carried out by comparing a p - value with the significance level instead
You will not be expected to calculate the p - value, it will be given in the question
Steps 1 and 4 will be the same, however you should compare the p - value with the significance level in step 3 (there is no step 2)
If the p - value is less than the significance level the test is significant and the null hypothesis should be rejected
If the p - value is greater than the significance level the null hypothesis should be accepted and the alternative hypothesis should be rejected
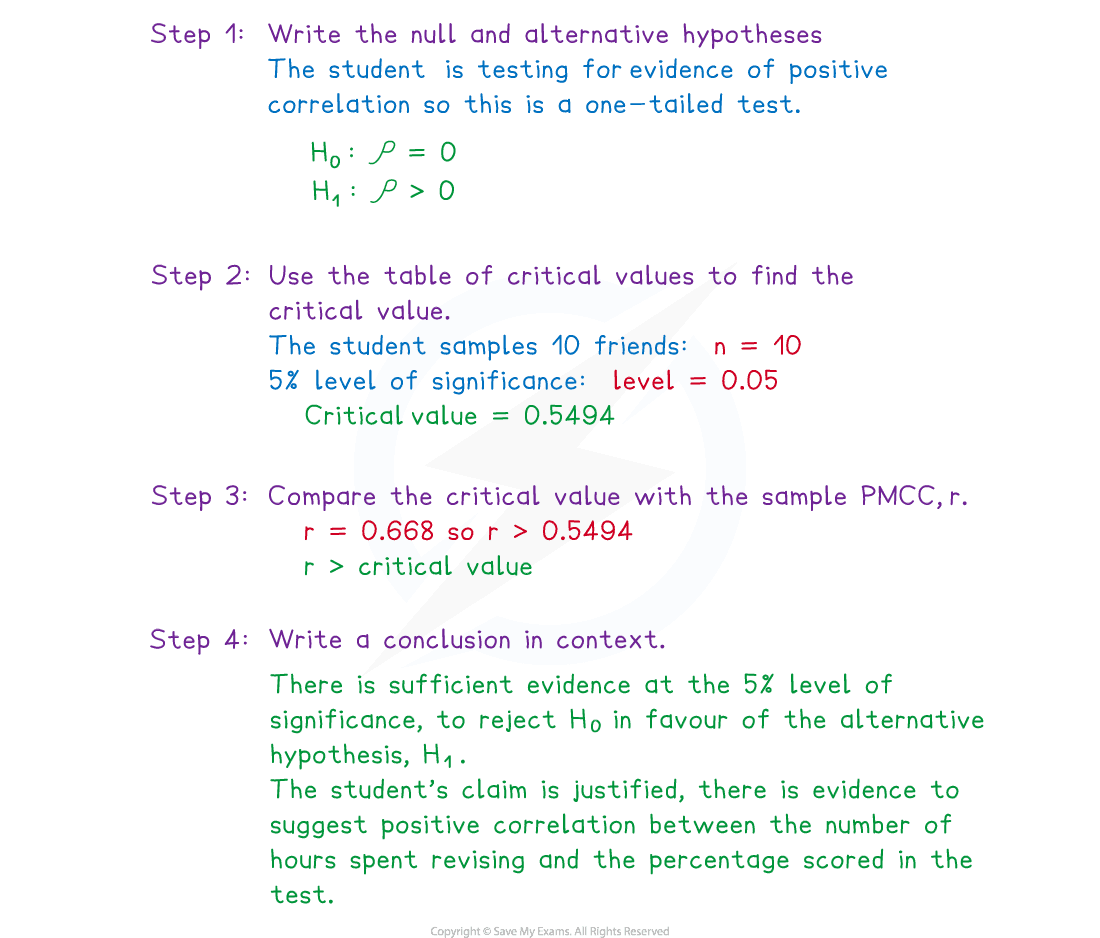
Worked Example
A student believes that there is a positive correlation between the number of hours spent studying for a test and the percentage scored on it.
The student takes a random sample of 10 of his friends and records the amount of revision they did and percentage they score in the test.
The student calculates the product moment correlation coefficient for these data as .
Carry out a hypothesis test at the 5% level of significance to test whether the student’s claim is justified.
Examiner Tips and Tricks
Make sure you read the question carefully to determine whether the test you are carrying out is for a one-tailed or a two-tailed test and use the level of significance accordingly. A test is only one-tailed if you are told to test for positive or negative correlation. If the questions says test for correlation then it is a two-tailed test, even if you think it is correlation would be positive.

Unlock more, it's free!
Did this page help you?