Revision Notes Exam Questions Past Papers

A LevelMathsOCRMaths APureRevision NotesDifferentiationFurther DifferentiationDifferentiating Other Functions

Differentiating Other Functions (OCR A Level Maths A) : Revision Note

Roger B

Author

Last updated

2 December 2024

Did this video help you?

Differentiating Other Functions (Trig, ln & e etc)

How do I differentiate common functions?

These are the common results
- $\frac{d}{d x} (x^{n}) = n x^{n - 1}$
- $\frac{d}{d x} (e^{x}) = e^{x}$
- $\frac{d}{d x} (a^{x}) = a^{x} \ln a$ for $a > 0$
- $\frac{d}{d x} (\ln x) = \frac{1}{x}$
- $\frac{d}{d x} (\sin x) = \cos x$
- $\frac{d}{d x} (\cos x) = - \sin x$
- $\frac{d}{d x} (\tan x) = \sec^{2} x$
- $\frac{d}{d x} (\cot x) = - \cos {ec}^{2} x$
- $\frac{d}{d x} (\sec x) = \sec x \tan x$
- $\frac{d}{d x} (\cos ec x) = - \cos ec x \cot x$

How do I differentiate exponentials and logarithms?

The two basic differentiation formulae are:

Diff Other Funct Illustr 1, AS & A Level Maths revision notes

From those basic formulae are derived these two additional formulae:

Diff Other Funct Illustr 2_forms, AS & A Level Maths revision notes

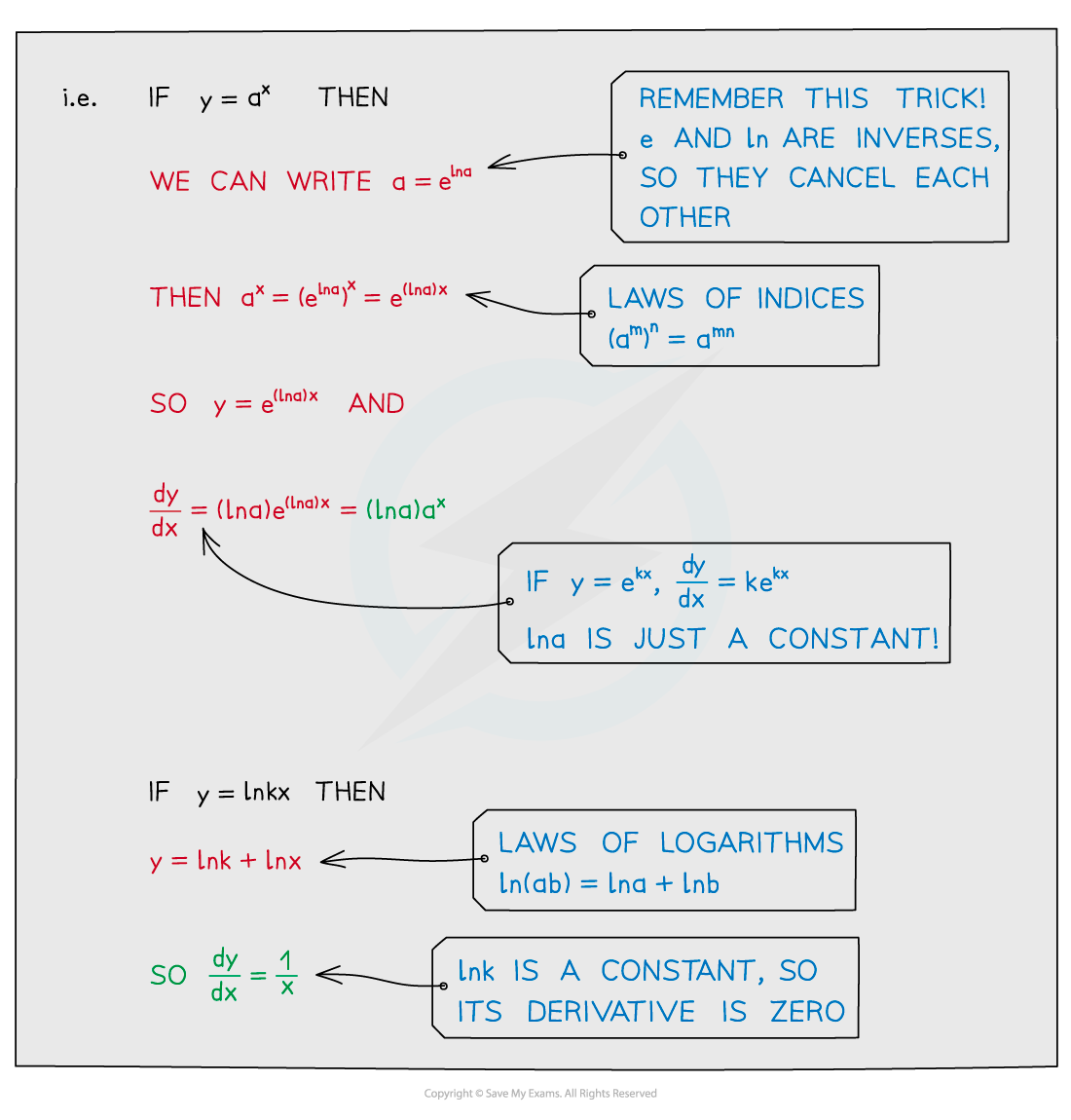
Diff Other Funct Illustr 2_derivs, AS & A Level Maths revision notes

And for exponentials more generally:

Diff Other Funct Illustr 3, AS & A Level Maths revision notes

This last formula can be derived from Formula 3 by using the chain rule

Examiner Tips and Tricks

The formulae for some of these derivatives are not given in the formulae booklet – you need to know them.
The formulae for e^kx and ln x are the ones you absolutely do need to know .
The other formulae can be derived from those two as shown above, and remember – the derivative of ln kx is $\frac{1}{x}$ , NOT $\frac{k}{x}$ !

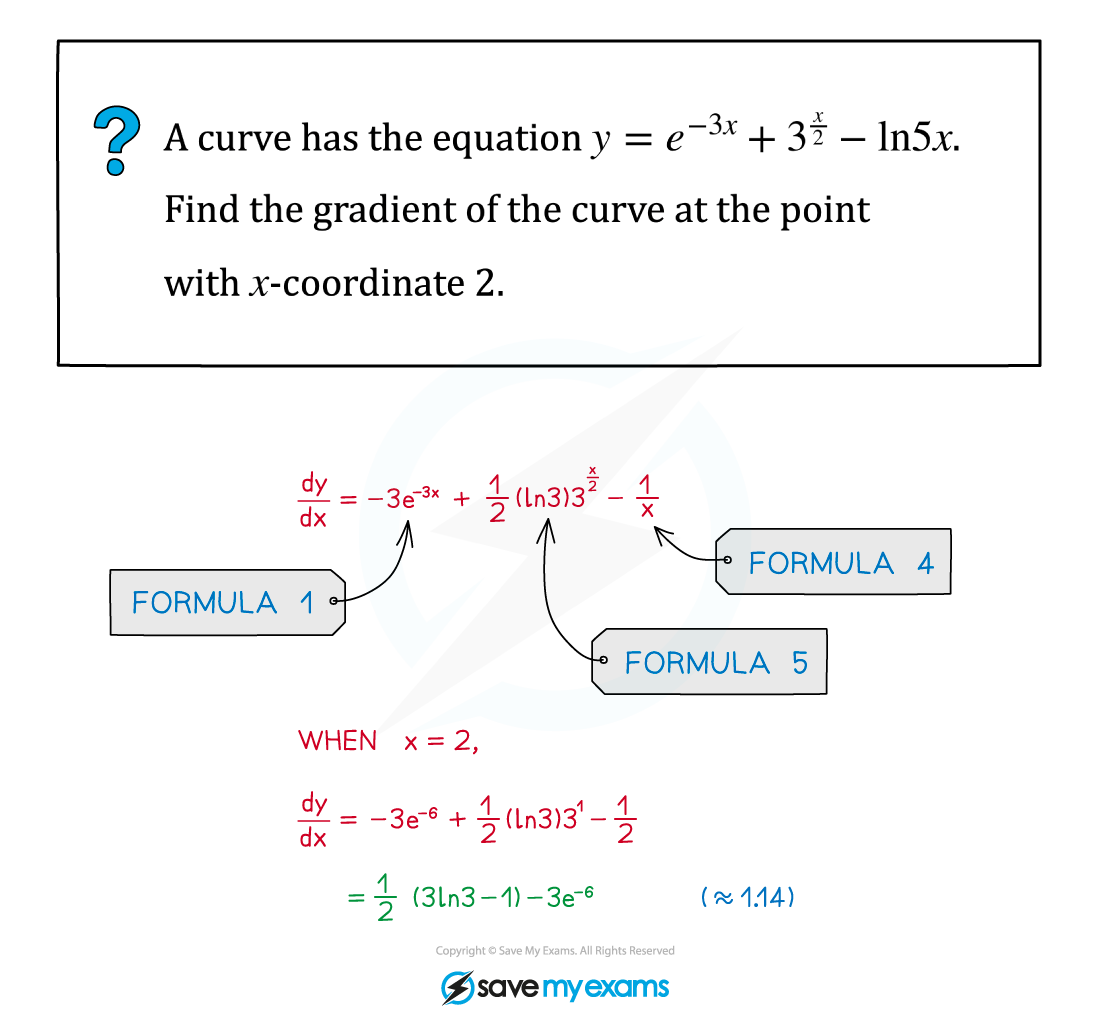
Worked Example

Diff Other Funct Example, AS & A Level Maths revision notes

You've read 0 of your 5 free revision notes this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

Did this page help you?

Previous:Differentiation of Trigonometric Functions from First PrinciplesNext:Chain Rule

Roger B

Author: Roger B

Expertise: Maths

Roger's teaching experience stretches all the way back to 1992, and in that time he has taught students at all levels between Year 7 and university undergraduate. Having conducted and published postgraduate research into the mathematical theory behind quantum computing, he is more than confident in dealing with mathematics at any level the exam boards might throw at you.