Translations (Edexcel A Level Maths: Pure)

What are graph transformations?

• When you alter a function in certain ways, the effects on the graph of the function can be described by geometrical transformations
• With a translation the shape, size, and orientation of the graph remain unchanged – the graph is merely shifted (up or down, left or right) in the xy plane

 

• A particular translation (how far left/right, how far up/down) is specified by a translation vector: 

 

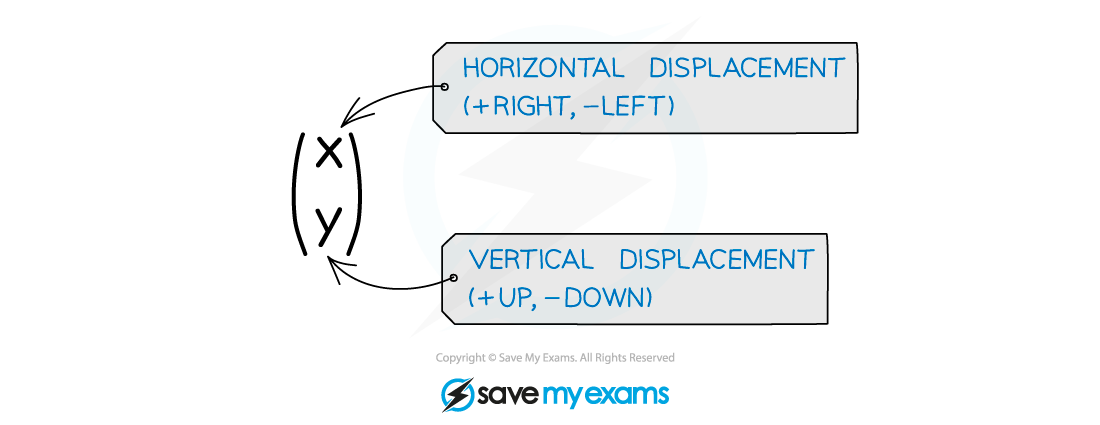
What do I need to know about graph translations?

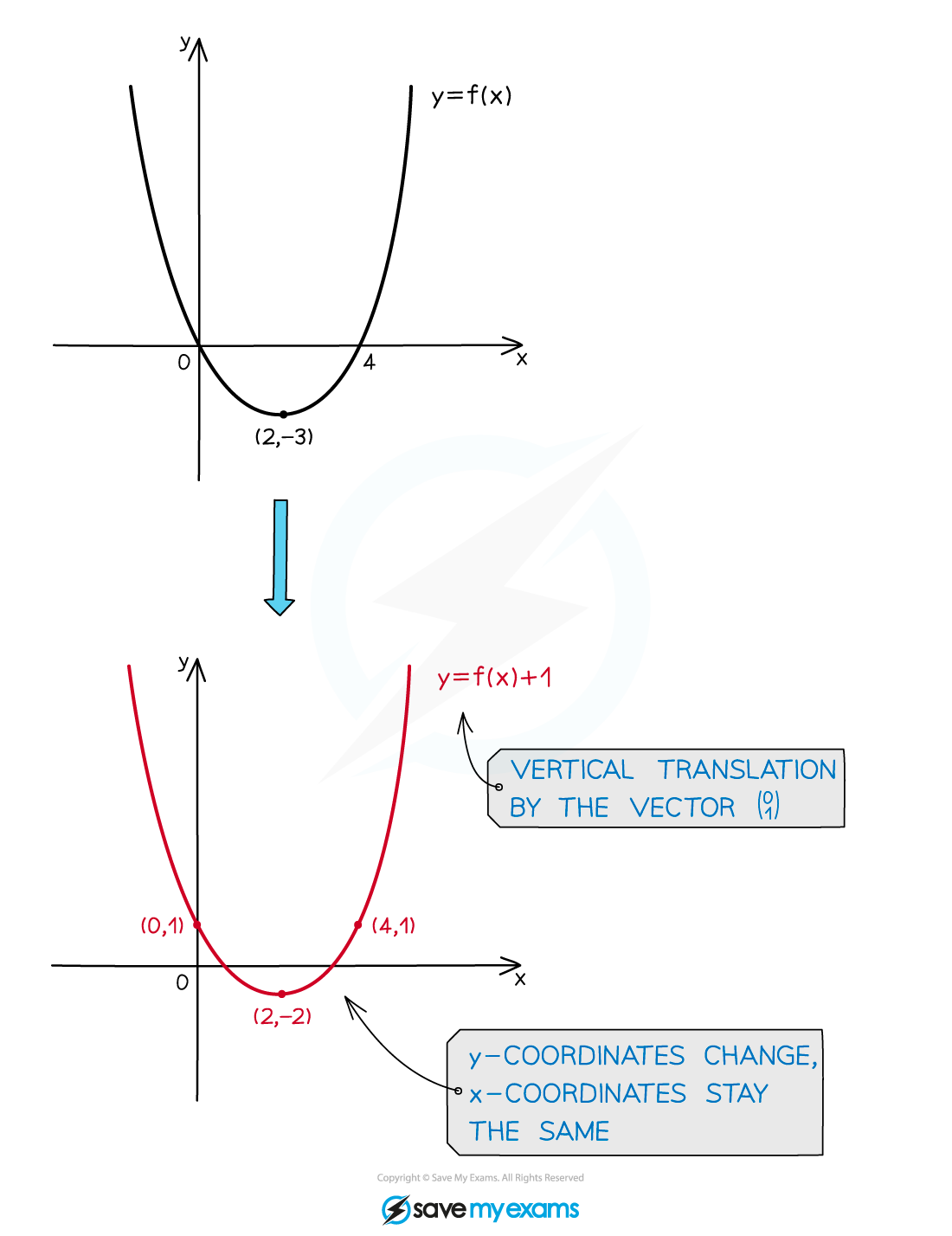
• The graph of  is a vertical translation of the graph  by the vector 
  • The graph moves up for positive values of a and down for negative values of a
  • The x-coordinates stay the same

 

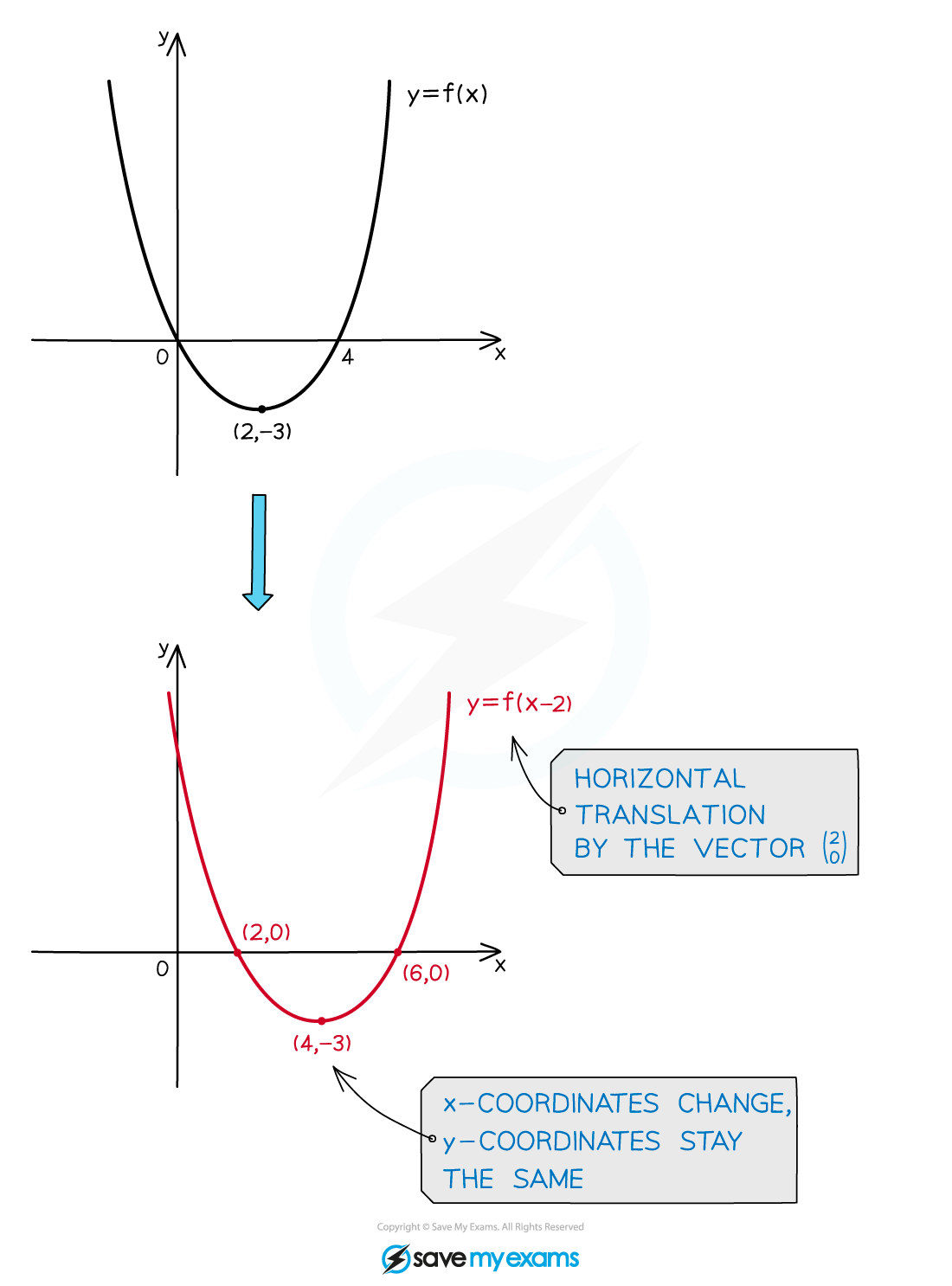
•  The graph of is a horizontal translation of the graph by the vector 
  • The graph moves left for positive values of a and right for negative values of a
  • The y-coordinates stay the same

 

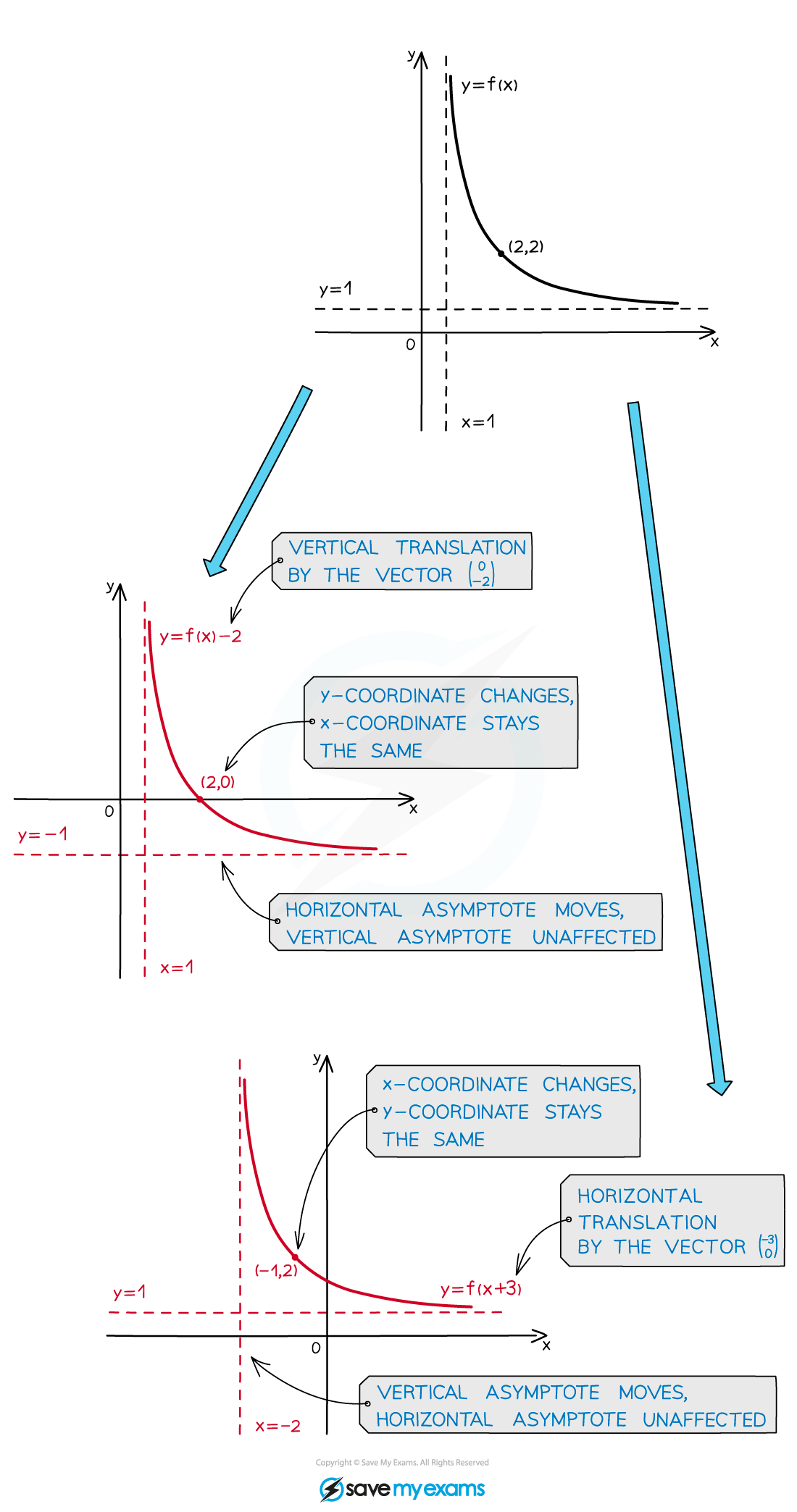
•  Any asymptotes of f(x) are also translated. If an asymptote is parallel to the direction of translation, however, it will not be affected