8.2.3 Modulus & Argument | CIE A Level Maths: Pure 3 Revision Notes 2020

Modulus & Argument

How do I find the modulus of a complex number?

The modulus of a complex number is its distance from the origin when plotted on an Argand diagram
The modulus of $z$ is written $|z|$
If $z = x + i y$ , then we can use Pythagoras to show…
- $|z| = \sqrt{x^{2} + y^{2}}$
A modulus is always positive
the modulus is related to the complex conjugate by…
- $z z^{*} = z^{*} z = {|z|}^{2}$
- This is because $z z^{*} = (x + i y) (x - i y) = x^{2} + y^{2}$
In general, $|z_{1} + z_{2}| \neq |z_{1}| + | z_{2} |$
- e.g. both $z_{1} = 3 + 4 i$ and $z_{2} = - 3 + 4 i$ have a modulus of 5, but $z_{1} + z_{2}$ simplifies to $8 i$ which has a modulus of 8

8-2-3_notes_fig1

How do I find the argument of a complex number?

The argument of a complex number is the anti-clockwise angle that it makes when starting at the positive real axis on an Argand diagram
Arguments are measured in radians
- Sometimes these can be given exact in terms of $π$
The argument of $z$ is written $\arg z$
Arguments can be calculated using right-angled trigonometry
- This involves using the tan ratio plus a sketch to decide whether it is positive/negative and acute/obtuse
Arguments are usually given in the range $- π < \arg z \leq π$
- Negative arguments are for complex numbers in the third and fourth quadrants
- Occasionally you could be asked to give arguments in the range $0 \leq \arg z < 2 π$
The argument of zero, $\arg 0$ is undefined (no angle can be drawn)

8-2-3_notes_fig2

Worked example

8-2-3_example_fig1-part-1

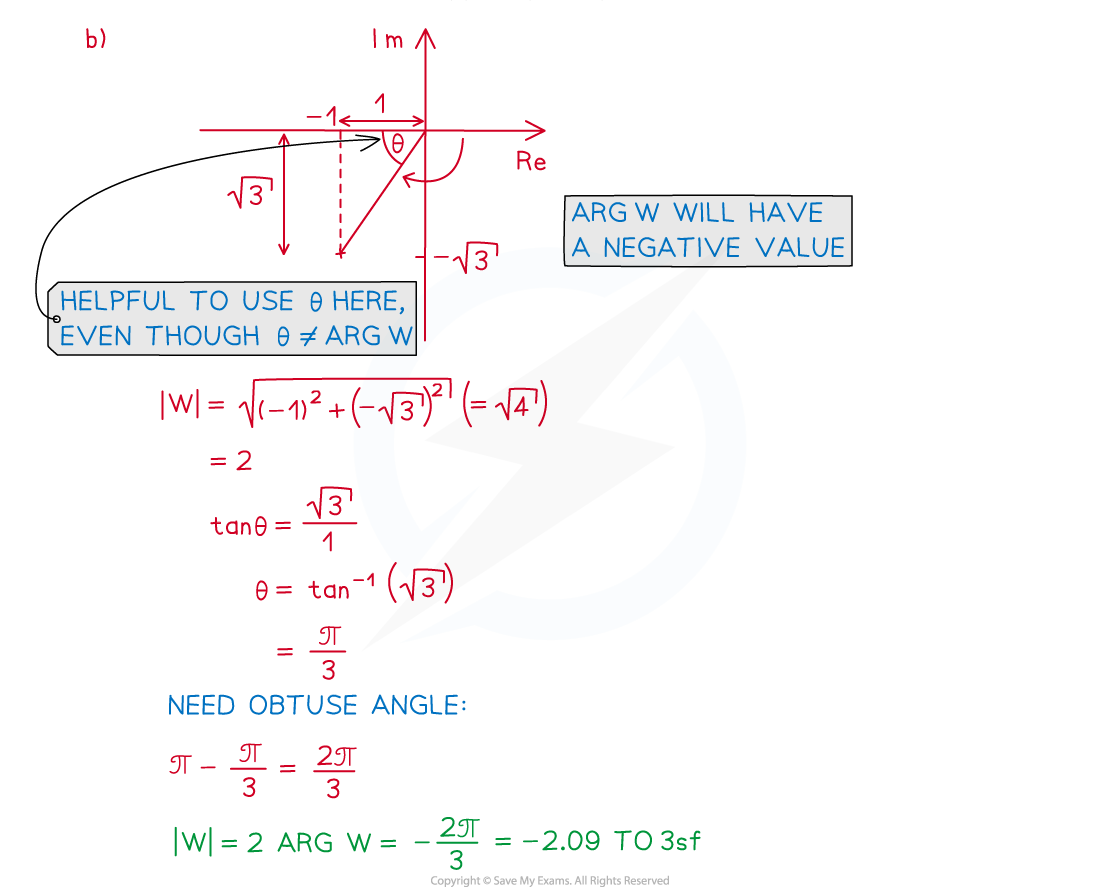
8-2-3_example_fig1-part-2

Exam Tip

Give non-exact arguments in radians to 3 significant figures.

Modulus-Argument (Polar) Form

The complex number $z = x + i y$ is said to be in Cartesian form. There are, however, other ways to write a complex number, such as in modulus-argument (polar) form.

How do I write a complex number in modulus-argument (polar) form?

The Cartesian form of a complex number, $z = x + i y$ , is written in terms of its real part, $x$ , and its imaginary part, $y$
If we let $r = | z |$ and $θ = \arg z$ , then it is possible to write a complex number in terms of its modulus, $r$ , and its argument, $θ$ , called the modulus-argument (polar) form, given by...
- $z = r (\cos θ + isin θ)$
It is usual to give arguments in the range $- π < θ \leq π$
- Negative arguments should be shown clearly, e.g. $z = 2 (\cos (- \frac{π}{3}) + isin (- \frac{π}{3}))$ without simplifying $\cos (- \frac{π}{3})$ to either $\cos (\frac{π}{3})$ or $\frac{1}{2}$
- Occasionally you could be asked to give arguments in the range $0 \leq θ < 2 π$
If a complex number is given in the form $z = r (\cos θ - isin θ)$ , then it is not currently in modulus-argument (polar) form due to the minus sign, but can be converted as follows…
- By considering transformations of trigonometric functions, we see that $- \sin θ \equiv \sin (- θ)$ and $\cos θ \equiv \cos (- θ)$
- Therefore $z = r (\cos θ - isin θ)$ can be written as $z = r (\cos (- θ) + isin (- θ))$ , now in the correct form and indicating an argument of $- θ$
To convert from modulus-argument (polar) form back to Cartesian form, evaluate the real and imaginary parts
- E.g. $z = 2 (\cos (- \frac{π}{3}) + isin (- \frac{π}{3}))$ becomes $z = 2 (\frac{1}{2} + i (- \frac{\sqrt{3}}{2})) = 1 - \sqrt{3} i$

8-2-3_notes_fig3

What are the rules for moduli and arguments under multiplication and division?

When two complex numbers, $z_{1}$ and $z_{2}$ , are multiplied to give $z_{1} z_{2}$ , their moduli are also multiplied
- $|z_{1} z_{2}| = |z_{1}| | z_{2} |$
When two complex numbers, $z_{1}$ and $z_{2}$ , are divided to give $\frac{z_{1}}{z_{2}}$ , their moduli are also divided
- $|\frac{z_{1}}{z_{2}}| = \frac{|z_{1}|}{|z_{2}|}$
When two complex numbers, $z_{1}$ and $z_{2}$ , are multiplied to give $z_{1} z_{2}$ , their arguments are added
- $\arg (z_{1} z_{2}) = \arg z_{1} + \arg z_{2}$
When two complex numbers, $z_{1}$ and $z_{2}$ , are divided to give $\frac{z_{1}}{z_{2}}$ , their arguments are subtracted
- $\arg (\frac{z_{1}}{z_{2}}) = \arg z_{1} - \arg z_{2}$

How do I multiply complex numbers in modulus-argument (polar) form?

The main benefit of writing complex numbers in modulus-argument (polar) form is that they multiply and divide very easily (often quicker than when in Cartesian form)
To multiply two complex numbers, $z_{1}$ and $z_{2}$ , in modulus-argument (polar) form we use the rules from above to multiply their moduli and add their arguments
- $|z_{1} z_{2}| = |z_{1}| |z_{2}|$
- $\arg (z_{1} z_{2}) = \arg z_{1} + \arg z_{2}$
So if $z_{1} = r_{1} (\cos θ_{1} + isin θ_{1})$ and $z_{2} = r_{2} (\cos θ_{2} + isin θ_{2})$ then the rules above give…
- $z_{1} z_{2} = r_{1} r_{2} (\cos (θ_{1} + θ_{2}) + isin (θ_{1} + θ_{2}))$
Sometimes the new argument, $θ_{1} + θ_{2}$ , does not lie in the range $- π < θ \leq π$ (or $0 \leq θ < 2 π$ if this is being used)
- An out-of-range argument can be adjusted by either adding or subtracting $2 π$
- E.g. If $θ_{1} = \frac{2 π}{3}$ and $θ_{2} = \frac{π}{2}$ then $θ_{1} + θ_{2} = \frac{7 π}{6}$
  - This is currently not in the range , but by subtracting $2 π$ from $\frac{7 π}{6}$ to give $- \frac{5 π}{6}$ , a new argument is formed that lies in the correct range and represents the same angle on an Argand diagram
The rules of multiplying the moduli and adding the arguments can also be applied when…
- …multiplying three complex numbers together, $z_{1} z_{2} z_{3}$ , or more
- …finding powers of a complex number (e.g. $z^{2}$ can be written as $z z$ )
Whilst not examinable, the rules for multiplication can be proved algebraically by multiplying $z_{1} = r_{1} (\cos θ_{1} + isin θ_{1})$ by $z_{2} = r_{2} (\cos θ_{2} + isin θ_{2})$ , expanding the brackets and using compound angle formulae

How do I divide complex numbers in modulus-argument (polar) form?

To divide two complex numbers, $z_{1}$ and $z_{2}$ in modulus-argument (polar) form, we use the rules from above to divide their moduli and subtract their arguments
- $|\frac{z_{1}}{z_{2}}| = \frac{|z_{1}|}{| z_{2} |}$
- $\arg (\frac{z_{1}}{z_{2}}) = \arg z_{1} - \arg z_{2}$
So if $z_{1} = r_{1} (\cos θ_{1} + isin θ_{1})$ and $z_{2} = r_{2} (\cos θ_{2} + isin θ_{2})$ then the rules above give…
- $\frac{z_{1}}{z_{2}} = \frac{r_{1}}{r_{2}} (\cos (θ_{1} - θ_{2}) + isin (θ_{1} - θ_{2}))$
As with multiplication, sometimes the new argument, $θ_{1} - θ_{2}$ , can lie out of the range $- π < θ \leq π$ (or the range $0 \leq θ < 2 π$ if this is being used)
- You can add or subtract $2 π$ to bring out-of-range arguments back in range
Whilst not examinable, the rules for division can be proved algebraically by dividing $z_{1} = r_{1} (\cos θ_{1} + isin θ_{1})$ by $z_{2} = r_{2} (\cos θ_{2} + isin θ_{2})$ , using complex division and compound angle formulae

Worked example

8-2-3_example_fig2-part-1

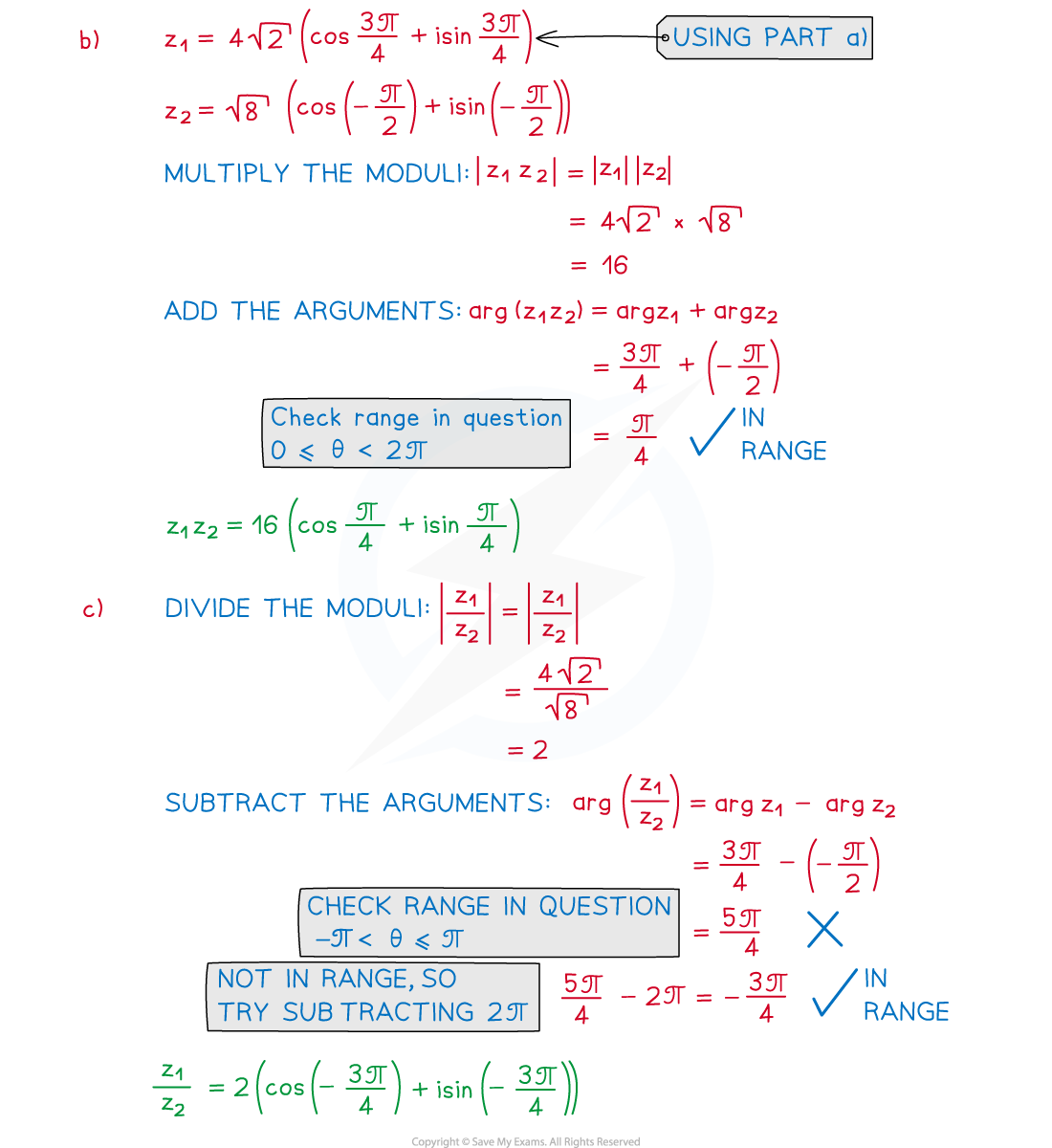
8-2-3_example_fig2-part-2

Exam Tip

The rules for multiplying and dividing in modulus-argument (polar) form must be learnt (they are not given in the formula booklet).
Remember to add or subtract $2 π$ to any out-of-range arguments to bring them back in range.
If a question does not give a clear range for arguments, then both $- π < θ \leq π$ or $0 < θ \leq 2 π$ would be accepted.

Modulus & Argument (CIE A Level Maths: Pure 3)

Revision Note

Modulus & Argument

How do I find the modulus of a complex number?

How do I find the argument of a complex number?

Worked example

Exam Tip

Modulus-Argument (Polar) Form

How do I write a complex number in modulus-argument (polar) form?

What are the rules for moduli and arguments under multiplication and division?

How do I multiply complex numbers in modulus-argument (polar) form?

How do I divide complex numbers in modulus-argument (polar) form?

Worked example

Exam Tip

You've read 0 of your 0 free revision notes

Get unlimited access

Join the 100,000+ Students that ❤️ Save My Exams

Author: Mark