Complex Roots of Polynomials (Cambridge (CIE) A Level Maths): Revision Note
Exam code: 9709
Complex roots of quadratics
What are complex roots?
Complex numbers provide solutions for quadratic equations which have no real roots

Complex roots occur when solving a quadratic with a negative discriminant
This leads to square rooting a negative number
How do I solve a quadratic equation with complex roots?
We solve an equation with complex roots in the same way we solve any other quadratic equations
If in the form
we can rearrange to solve
If in the form
we can complete the square or use the quadratic formula
We use the property
along with a manipulation of surds
When the coefficients of the quadratic equation are real, complex roots occur in complex conjugate pairs
If
is a root of a quadratic with real coefficients then
is also a root
When the coefficients of the quadratic equation are non-real, the solutions will not be complex conjugates
To solve these use the quadratic formula
How do I find a quadratic equation given one complex root?
We can find the equation of the form
if you are given a complex root in the form
We know that the complex conjugate
is another root,
This means that
and
are factors of the quadratic equation
Therefore
Writing this as the difference of two squares -
- will speed up expanding
Expanding and simplifying gives us a quadratic equation where
and
are real numbers
Worked Example

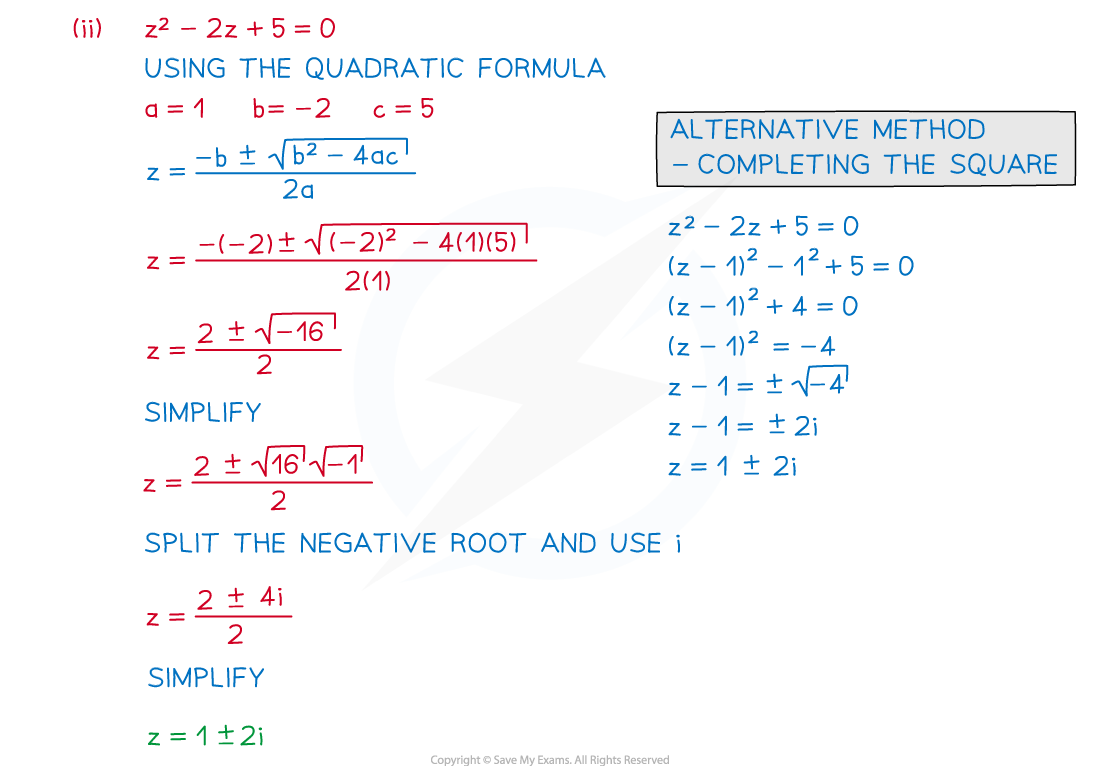
Examiner Tips and Tricks
Once you have your final answers you can check your roots are correct by substituting your solutions back into the original equation.
You should get 0 if correct! [Note: 0 is equivalent to
]
Did this video help you?
Complex Roots of cubics & quartics
How many roots should a polynomial have?
We know from previous sections that every quadratic equation has two roots (not necessarily distinct)
This is a particular case of a more general rule:
Every polynomial equation, with real coefficients, of degree n has n roots
The n roots are not necessarily all distinct and therefore we need to count any repeated roots that may occur individually
From the above rule we can state the following:
A cubic equation of the form
can have either:
3 real roots
Or 1 real root and a complex conjugate pair
A quartic equation of the form
will have the following cases for roots:
4 real roots
2 real and 2 non-real roots(a complex conjugate pair)
4 non-real roots (two complex conjugate pairs)

Number of roots of a cubic function

Number of roots of a quartic function
How do I solve a cubic equation given one complex root?
Steps to solve a cubic equation with complex roots
If we are told that
is a root, then we know
is also a root
This means that
and
are factors of the cubic equation
Multiply the above factors together gives us a quadratic factor of the form
We need to find the third factor
Multiply the factors and equate to our original equation to get
From there either
Expand and compare coefficients to find
Or use polynomial division to find the factor
Finally, write your three roots clearly
How do I solve a quartic equation given one complex root?
When asked to find the roots of a quartic equation when we are given one, we use almost the same method as we did for a cubic equation
State the initial root and its conjugate and write their factors as a quadratic factor (as above) we will have two unknown roots to find, write these as factors
and
The unknown factors also form a quadratic factor
Then continue with the steps from above, either comparing coefficients or using polynomial division
If using polynomial division, then solve the quadratic factor you get to find the roots
and
How do I find unknown coefficients of cubics and quartics?
Steps to find unknown variables in a given equation when given a root:
Substitute the given root into the equation
Expand and group together the real and imaginary parts (these expressions will contain our unknown values)
Solve as simultaneous equations to find the unknowns
Substitute the values into the original equation
From here continue using the previously described methods for finding other roots for either cubic/quartic equations
Worked Example


Examiner Tips and Tricks
As with solving quadratic equations, we can substitute our solutions back into the original equation to check we get 0.

Unlock more, it's free!
Did this page help you?