A LevelMaths: Pure 3CIERevision Notes3. Trigonometry3.2 Compound & Double Angle Formulae3.2.1 Compound Angle Formulae

Compound Angle Formulae (CIE A Level Maths: Pure 3)

Revision Note

Test yourself

Author

Roger

Last updated

19 May 2023

Did this video help you?

Compound Angle Formulae

What are the compound angle formulae?

There are six compound angle formulae (also known as addition formulae), two each for sin, cos and tan:
For sin the +/- sign on the left-hand side matches the one on the right-hand side

\sin (A + B) \equiv \sin A \cos B + \cos A \sin B

\sin (A - B) \equiv \sin A \cos B - \cos A \sin B

For cos the +/- sign on the left-hand side is opposite to the one on the right-hand side

\cos (A + B) \equiv \cos A \cos B - \sin A \sin B

\cos (A - B) \equiv \cos A \cos B + \sin A \sin B

For tan the +/- sign on the left-hand side matches the one in the numerator on the right-hand side, and is opposite to the one in the denominator

\tan (A + B) \equiv \frac{\tan A + \tan B}{1 - \tan A \tan B}

\tan (A - B) \equiv \frac{\tan A - \tan B}{1 + \tan A \tan B}

You can derive the tan identity by:
- Writing $\tan (A + B) \equiv \frac{\sin (A + B)}{\cos (A + B)}$
- Dividing the numerator and denominator by $\cos A \cos B$

Examiner Tip

All these formulae are in the formulae booklet – you don't have to memorise them.

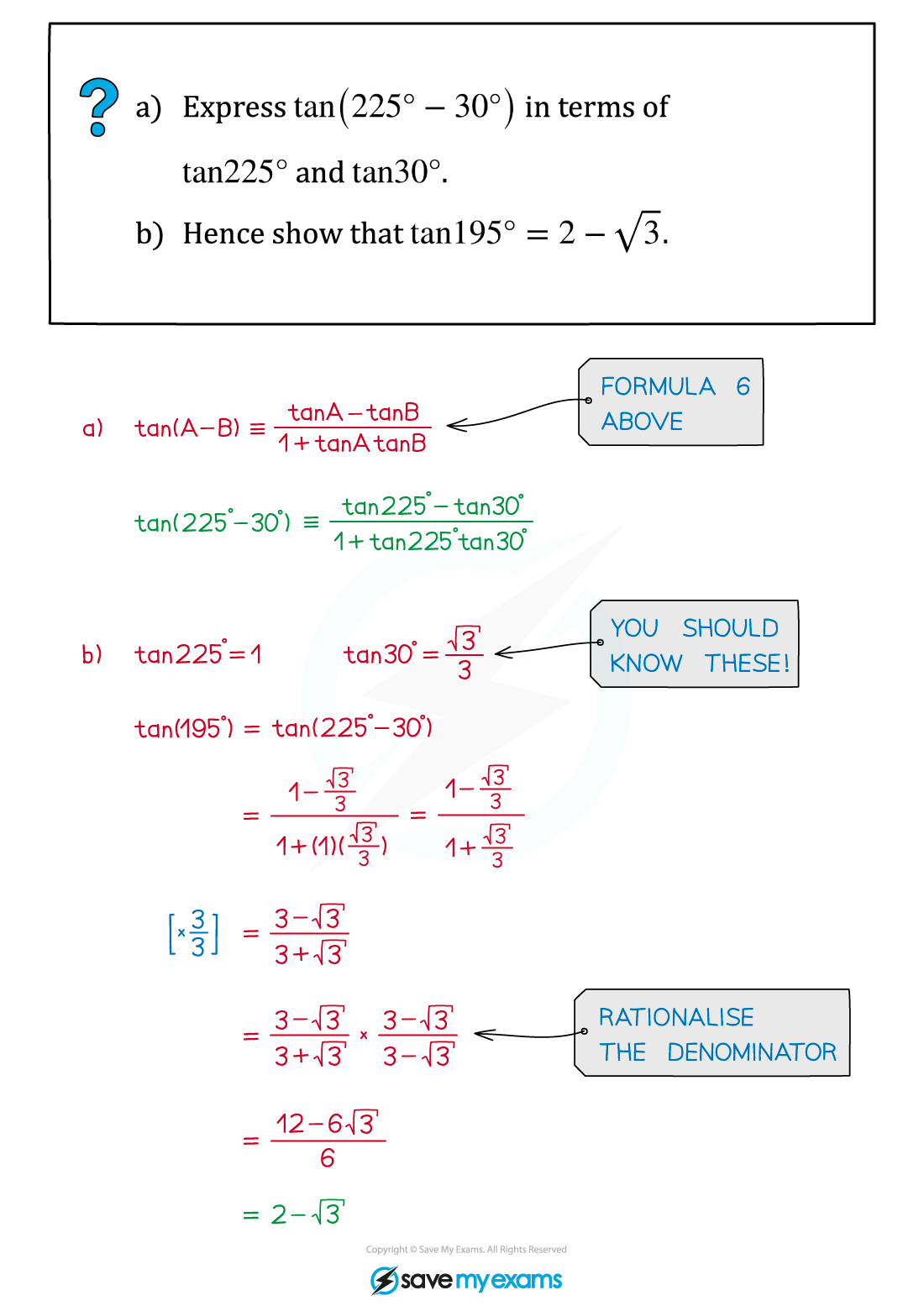
Worked example

Comp Angle Forms Example, A Level & AS Maths: Pure revision notes

You've read 0 of your 10 free revision notes

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

Test yourself Next topic

Did this page help you?

Author: Roger

Expertise: Maths

Roger's teaching experience stretches all the way back to 1992, and in that time he has taught students at all levels between Year 7 and university undergraduate. Having conducted and published postgraduate research into the mathematical theory behind quantum computing, he is more than confident in dealing with mathematics at any level the exam boards might throw at you.