Derivatives of Exponential Functions (Cambridge (CIE) A Level Maths) : Revision Note
Did this video help you?
Derivatives of Exponential Functions
What is the derivative of e?
y = ex has the particular property
ie for every real number x, the gradient of y = ex is also equal to ex (see Derivatives of Exponential Functions)
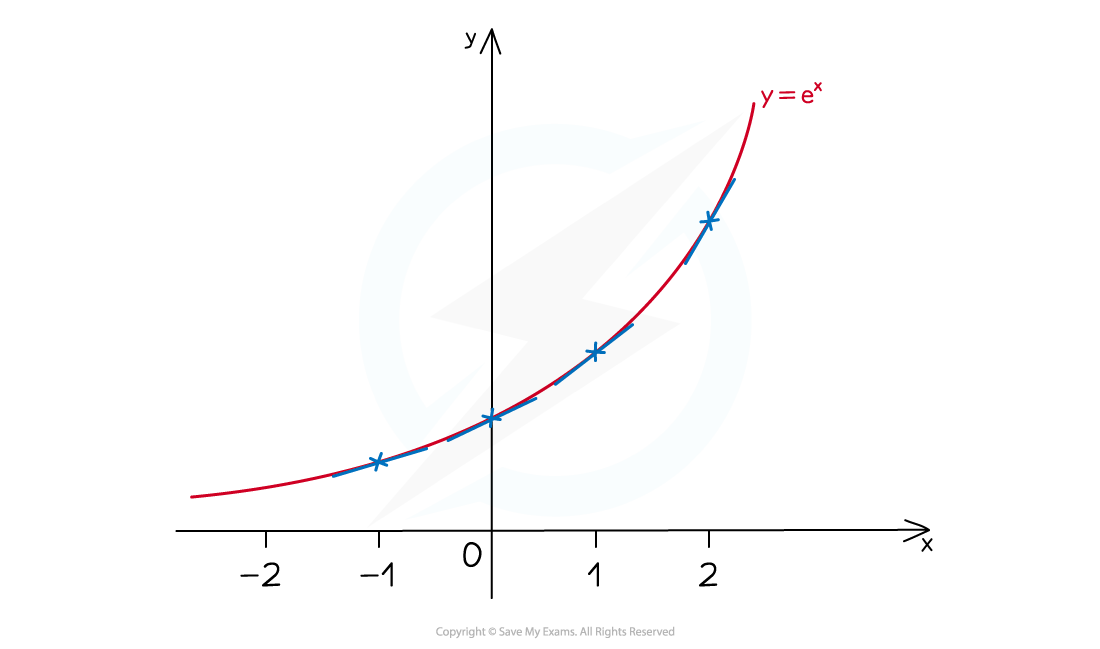

e ≈ 2.718 (see "e")
Recall that the derivative is the gradient function for a curve (see First Principles Differentiation)
Graphs and derivatives related to e
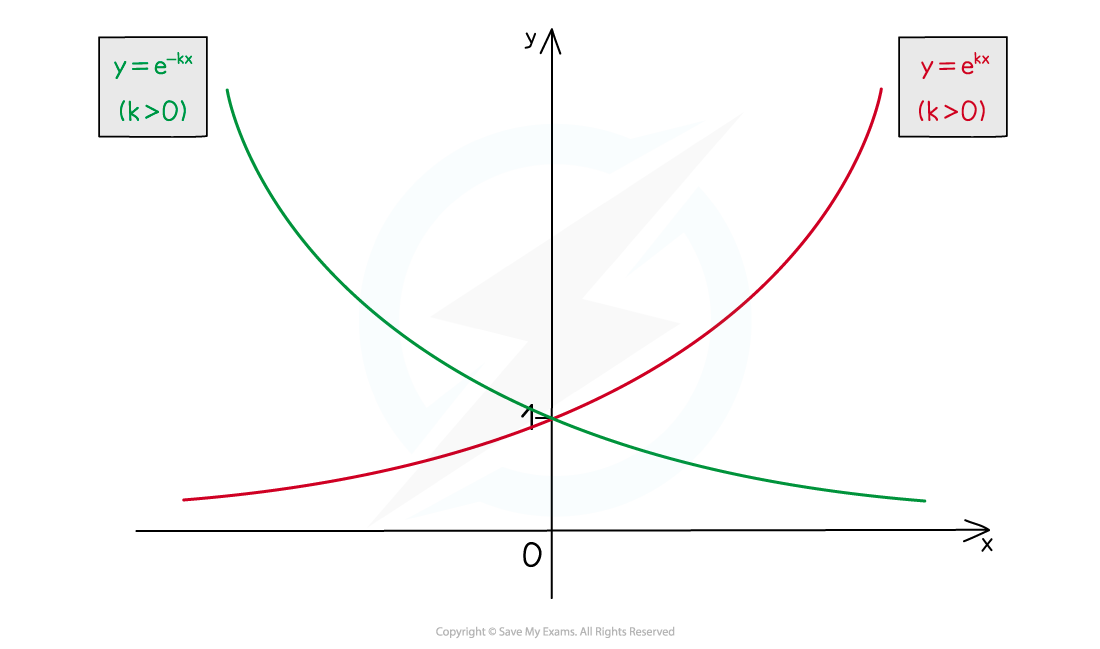
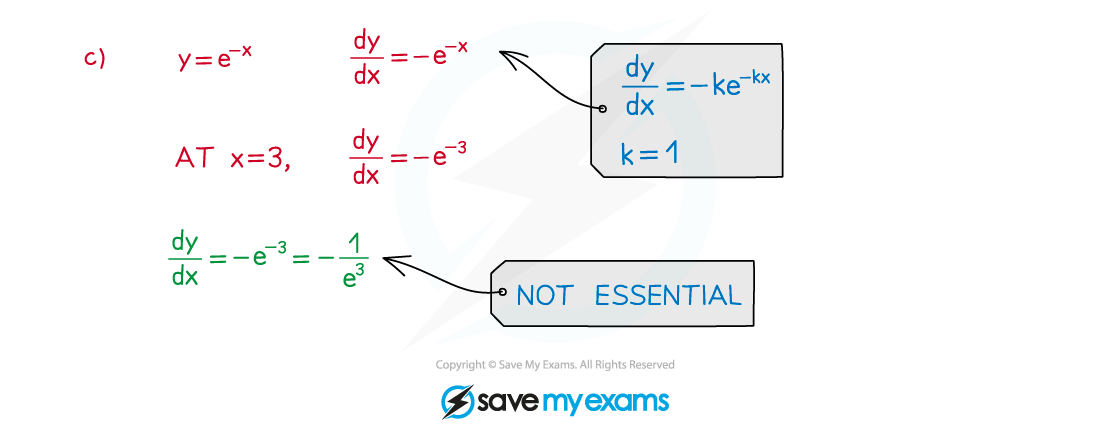
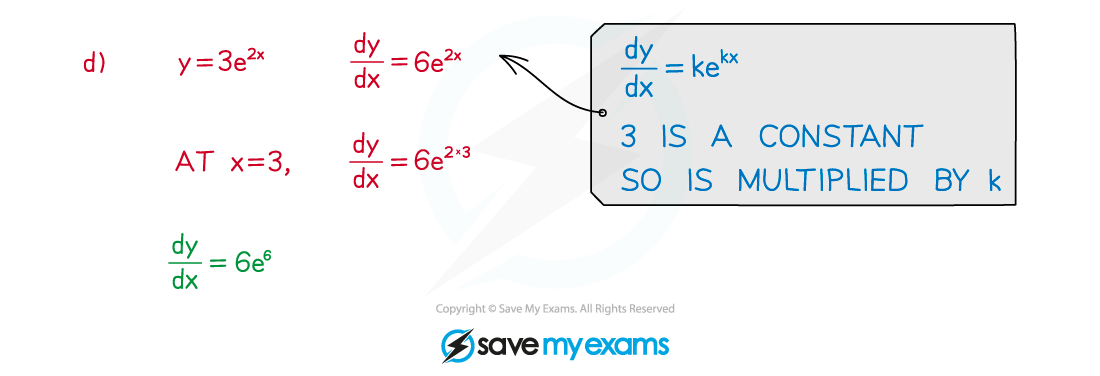
The derivative of y = ekx is
The derivative of y = e-kx is
Examiner Tips and Tricks
Remember that (like π) e is a number.
Exam questions can ask for answers to be given as exact values in terms of e (see the Worked Example below).
Worked Example
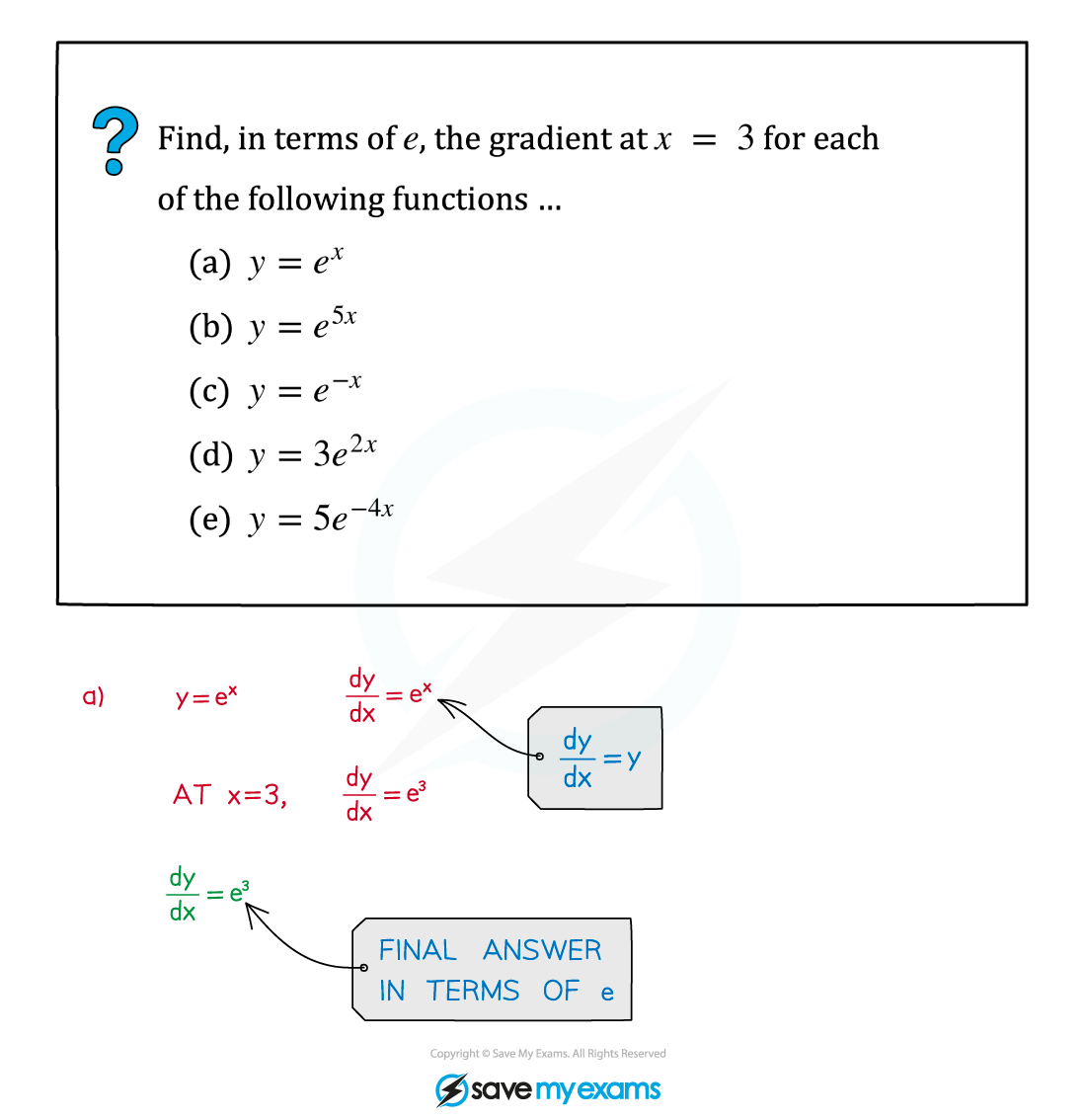
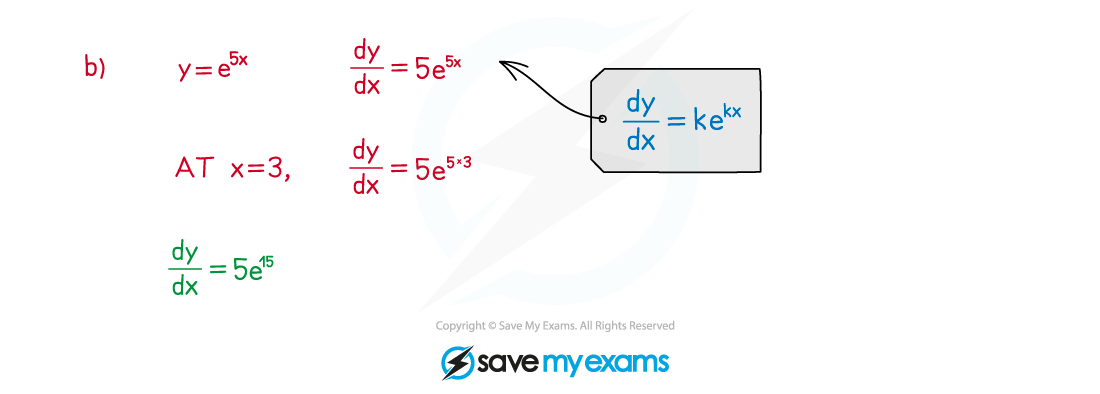
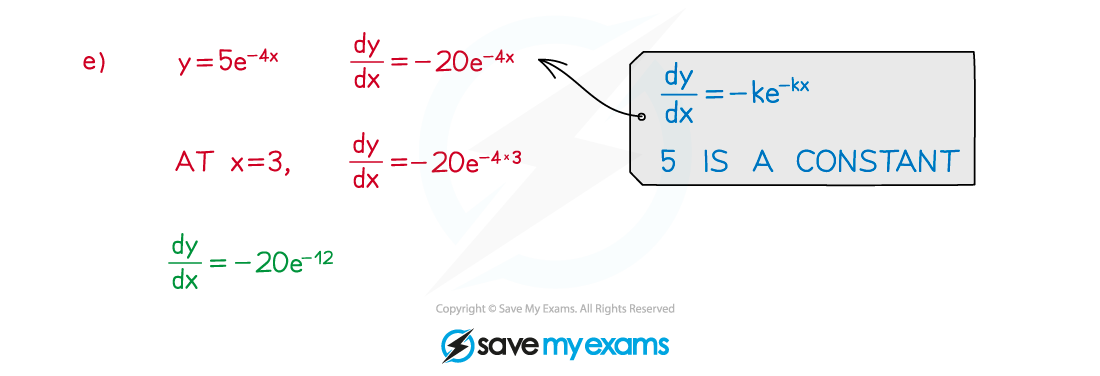

You've read 0 of your 5 free revision notes this week
Sign up now. It’s free!
Did this page help you?
