Sketching Reciprocal Graphs (Cambridge (CIE) A Level Maths): Revision Note
Exam code: 9709
Did this video help you?
Sketching reciprocal curves
What are reciprocal graphs?
Reciprocal graphs involve equations with an
term on the denominator e.g.
There are two basic reciprocal graphs to know for A level
and
The second one of these is always positive

More reciprocal graphs
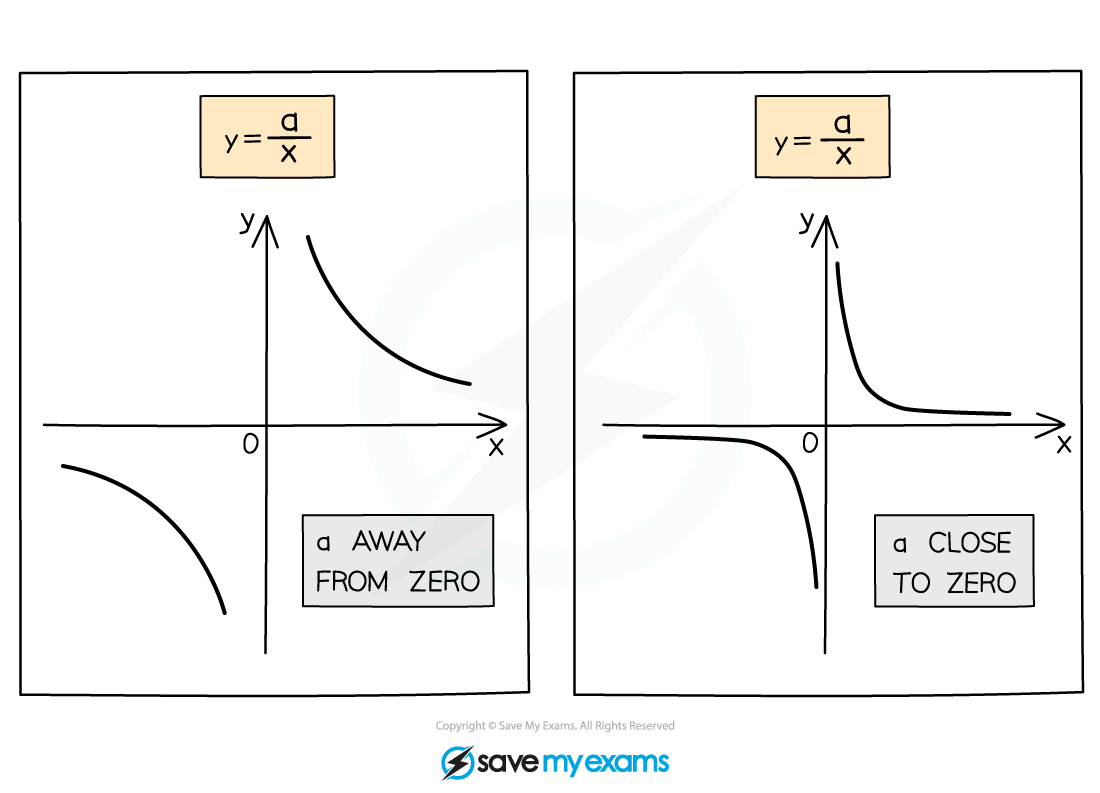
You also need to recognise graphs where the numerator is not one

The sign of a shows which part of the graph the curves are located
The size of a shows how steep the curves are
The closer a is to 0 the more L-shaped the curves are
horizontal, y = 0 (x-axis)
vertical, x = 0 (y-axis)All have two asymptotes
How do I sketch a reciprocal graph?

STEP 1 Use the sign of “a” to locate the curves
and use the size of “a” to gauge the steepness of the curve
STEP 2 Sketch the graph
STEP 3 Label the points x = 1 and x = -1 as a guide to the scale of your graph
STEP 4 Draw asymptotes with a dotted line Draw asymptotes with dotted lines
These graphs do not intercept either axis
Graph transformations of them could cross the axes (see Translations)
Worked Example


Unlock more, it's free!
Did this page help you?